Notes
two hexagons and a triangle solution

Solution to the Puzzle

Solution by Angle at the Centre is Twice the Angle at the Circumference

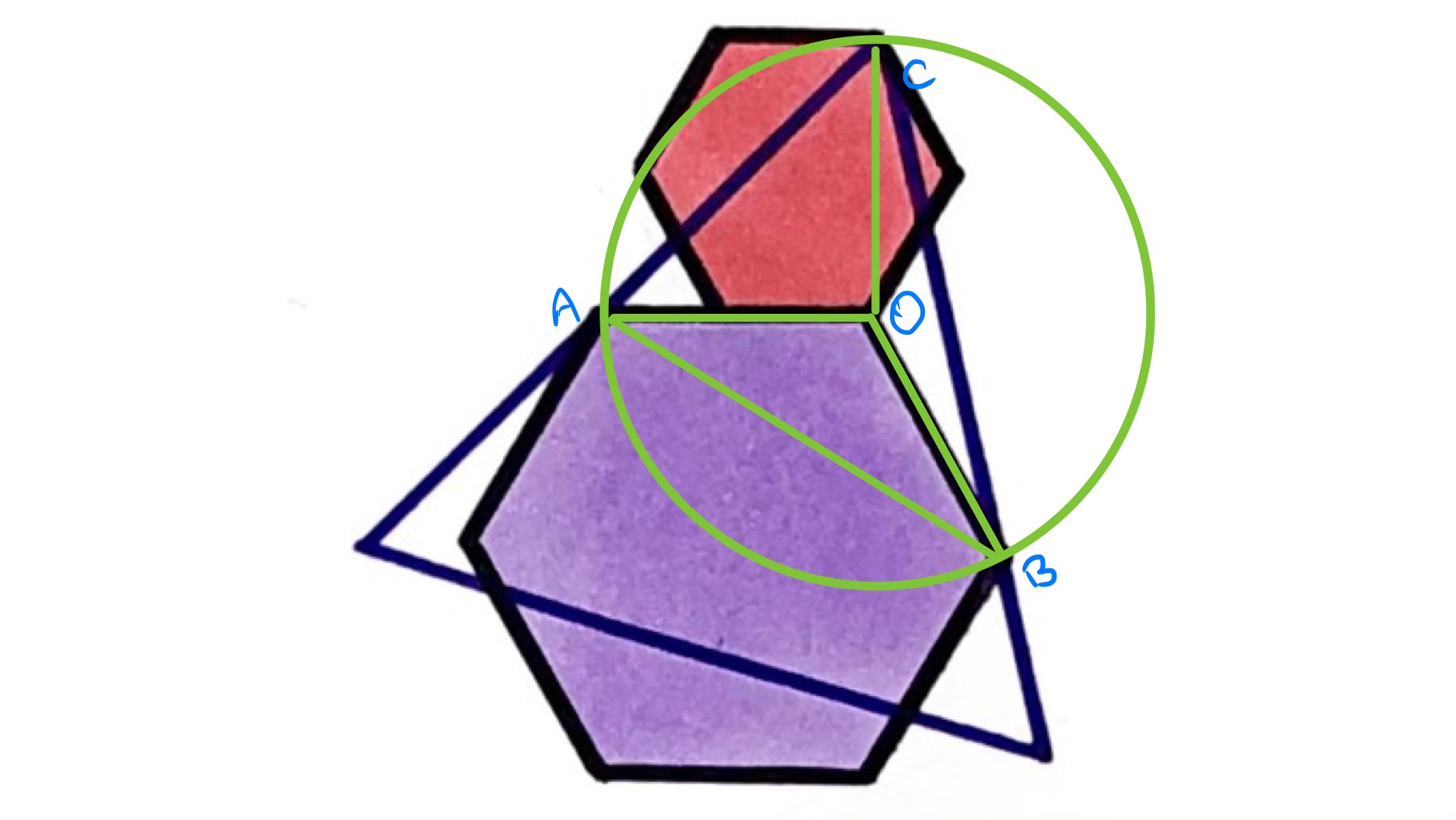
With the points labelled as above, consider the circle centre $O$ through $A$. As the purple hexagon is regular, $O B = O A$ so this circle also passes through $B$. The angle $A \hat{O} B$ is $120^\circ$ as it is the interior angle in a regular hexagon. Angle $A \hat{C} B$ is $60^\circ$ as the interior angle in an equilateral triangle which is half $120^\circ$. Hence by the fact that the angle at the centre is twice the angle at the circumference, $C$ lies on the circle. Hence $O C = O A$.

Now $O C$ is $\sqrt{3}$ times the side length of the smaller hexagon (see the page on hexagons for how the various lengths are related) so the scale factor from the red hexagon to the purple hexagon is $\sqrt{3}$ and hence the area scale factor is $3$.

Thus the area of the larger hexagon is $3 \times 5 = 15$.