Notes
quartered hexagon solution

Solution to the Quartered Hexagon Puzzle

Solution by Properties of Regular Hexagons and Equilateral Triangles, and Areas of a Triangle and a Trapezium

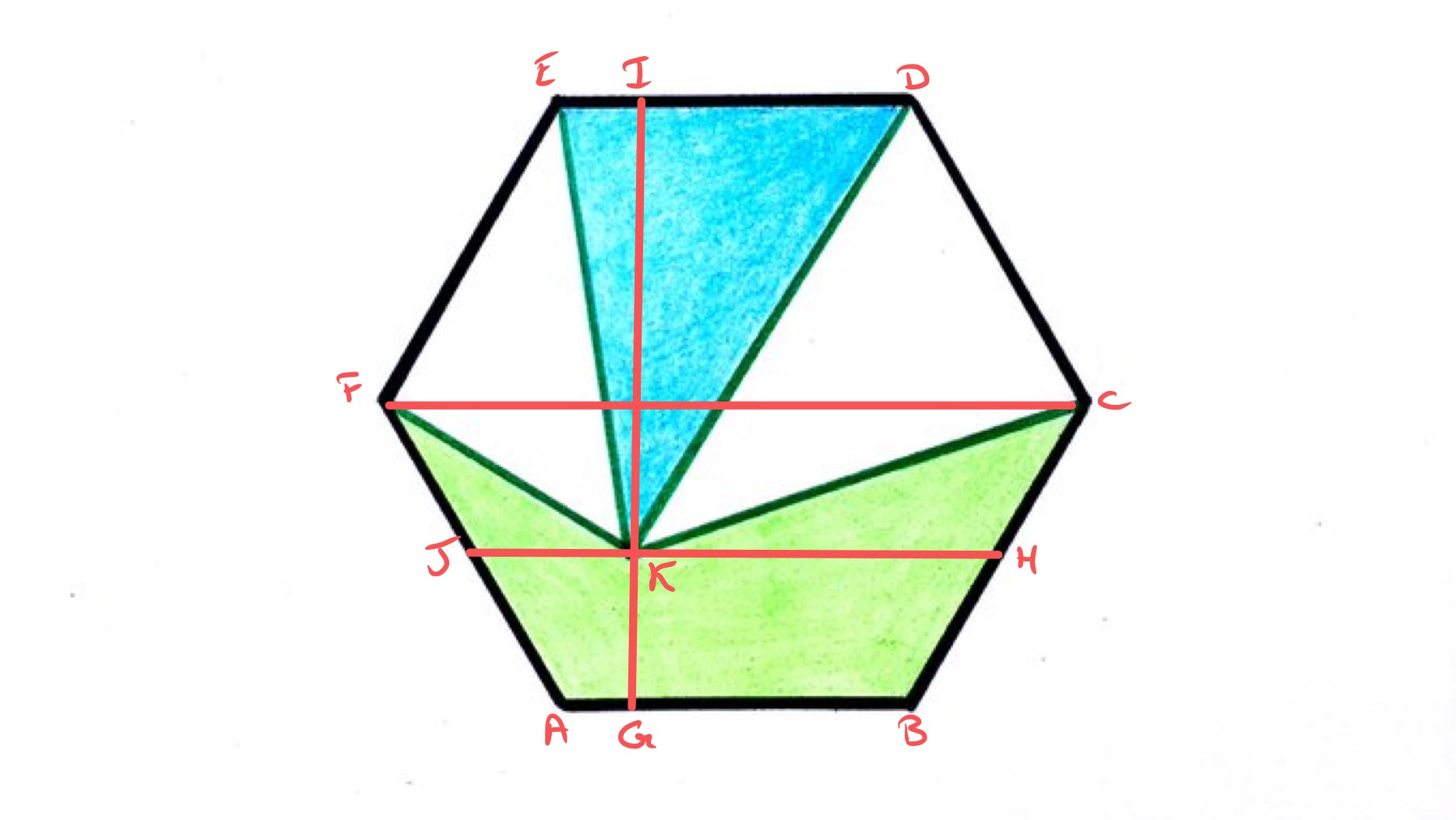
In the above diagram, the line $J H$ is horizontal and passes through $K$, while $I G$ is vertical and likewise through $K$.

The regular hexagon decomposes into six equilateral triangles with the same side length as the hexagon. Therefore, the area of one of these triangles is a sixth of the area of the full hexagon. The height of one of these triangles is the perpendicular distance from the centre to a side, which is half the length of $I G$. So a triangle with base $E D$ and full height the length of $I G$ has area one third of the hexagon. Since $E K D$ has area one quarter of the hexagon, the length of $I K$ must be $\frac{3}{4}$ of the length of $I G$.

The green area can then be decomposed in different ways to calculate its area, but ultimately they end up comparing it to the trapezium $A B C F$ with area half that of the hexagon. Writing $a$ for the side length of the hexagon and $b$ for the perpendicular distance between $F C$ and $A B$ then the area of the trapezium is $\frac{3}{2} a b$ so $a b$ represents a third of the hexagon.

Slicing the green region along $J H$ splits it into a trapezium and two triangles. The length of $J H$ is $\frac{3}{2} a$ and of $G K$ is $\frac{1}{2} b$ so the area of the trapezium is $\frac{5}{8} a b$. The two triangles have height $\frac{1}{2} b$ and their combined base is $\frac{3}{2} a$ so their combined area is $\frac{3}{8} a b$. The green region thus has total area $a b$ which, as established above, is a third of the hexagon.

An alternative decomposition is to view the green region as being half the hexagon with triangle $K C F$ removed. With $C F$ as its “base”, this triangle has base $2 a$ and height $\frac{1}{2} b$ so its area is $\frac{1}{2} a b$. Its area is therefore one sixth of the hexagon so the green area is one third.

Solution by Invariance Principle

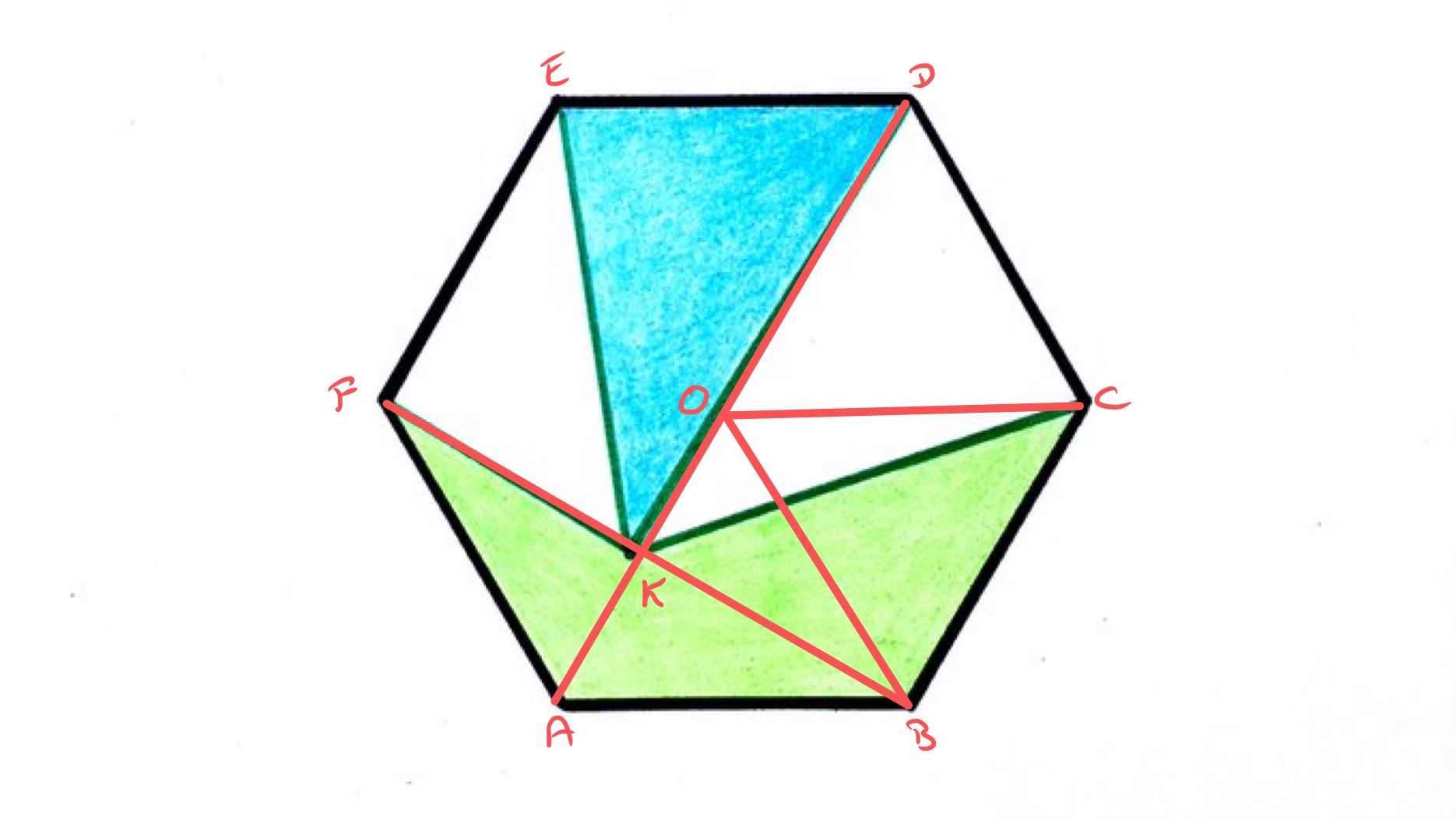
The point $K$ in the diagram can be anywhere in the hexagon provided triangle $E K D$ occupies a quarter of the total area. There is a possible configuration where $K$ lies on the diagonal $A D$, since if $K$ is at $A$ then the blue triangle is a third of the hexagon while if $K$ is at $O$ (the centre) then the blue triangle is a sixth.

With $K$ on the line $A D$ then the triangle $C K B$ has the same area as $C O B$ since both have the same “base” $C B$ and the same “height” above this base. Therefore, triangle $C K B$ is one sixth of the hexagon. Triangle $A B F$ is also one sixth, so the area of the green region is one third of the area of the hexagon.