Notes
hexagon and concentric circles solution

Skip the Navigation Links | Home Page | Feeds

Hexagon and Concentric Circles

Hexagon and Concentric Circles

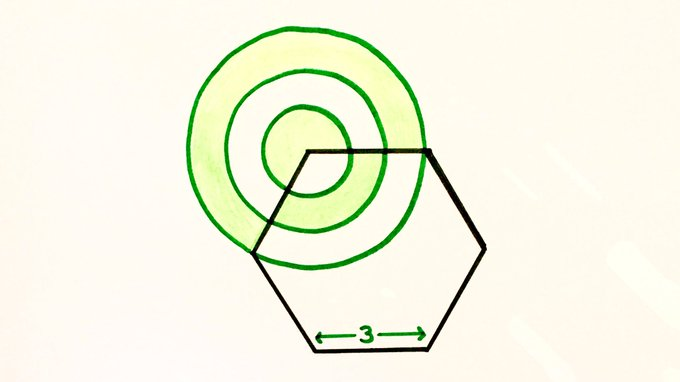
The regular hexagon has a side length of 33, and the rings are equally spaced. What’s the total shaded area?

Solution by Interior Angle of a Regular Hexagon and Area of a Circle

The interior angle of a regular hexagon is 120 ∘120^\circ, which is one third of a full turn. The part of the outer ring is 23(π3 2−π2 2)\frac{2}{3} (\pi 3^2 - \pi 2^2), that of the middle ring is 13(π2 2−π1 2)\frac{1}{3} (\pi 2^2 - \pi 1^2), and of the inner ring is 23(π1 2)\frac{2}{3} (\pi 1^2). Putting that together gives:

23(π3 2−π2 2)+13(π2 2−π1 2)+23(π1 2)=5π. \frac{2}{3} (\pi 3^2 - \pi 2^2) + \frac{1}{3} (\pi 2^2 - \pi 1^2) + \frac{2}{3} (\pi 1^2) = 5 \pi.
Created on October 3, 2021 15:23:09 by Andrew Stacey
Views: Print | TeX | Source | Linked from: hexagon and concentric circles