Notes
two triangles in a circle and semi-circle solution

Solution to the Two Triangles in a Circle and Semi-Circle Puzzle

Solution by Cyclic quadrilateral, Angles at a point on a straight line, and Crossed trapezium

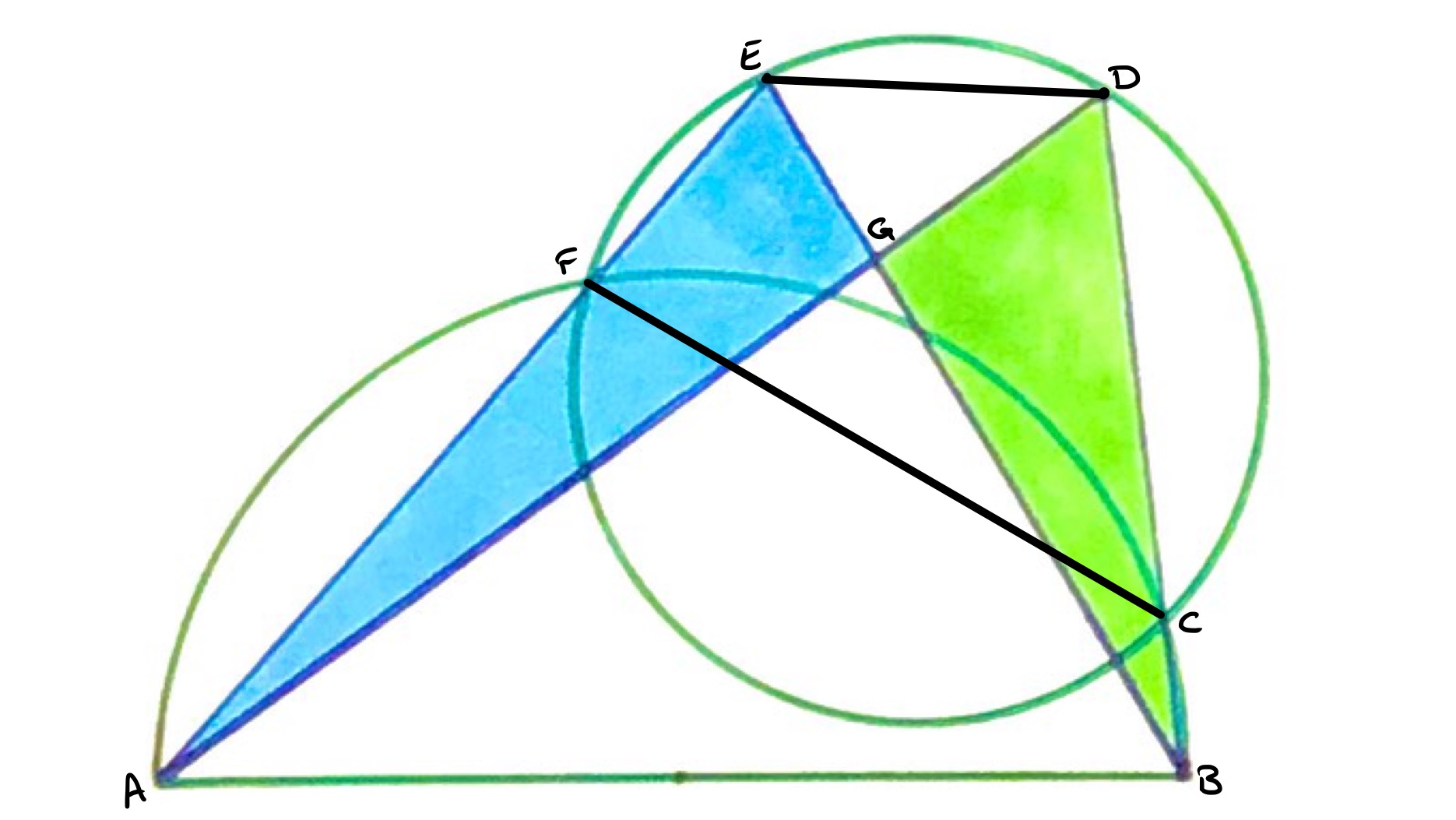
In the above diagram, quadrilateral $A B C F$ is cyclic (in the semi-circle) and so angles $A \hat{F} C$ and $C \hat{B} A$ add up to $180^\circ$ (that is, are supplementary). For the same reason, angles $C \hat{F} E$ and $E \hat{D} C$ also add up to $180^\circ$. Since angles at a point on a straight line add up to $180^\circ$, angles $A \hat{F} C$ and $C \hat{F} E$ add up to $180^\circ$. Putting all those together, angles $E \hat{D} C$ and $C \hat{B} A$ add up to $180^\circ$. This establishes $E D$ and $A B$ as parallel and so quadrilateral $A B D E$ is a trapezium.

As detailed at crossed trapezium, the two triangles therefore have the same area.