Notes
two pentagons solution
Two Pentagons
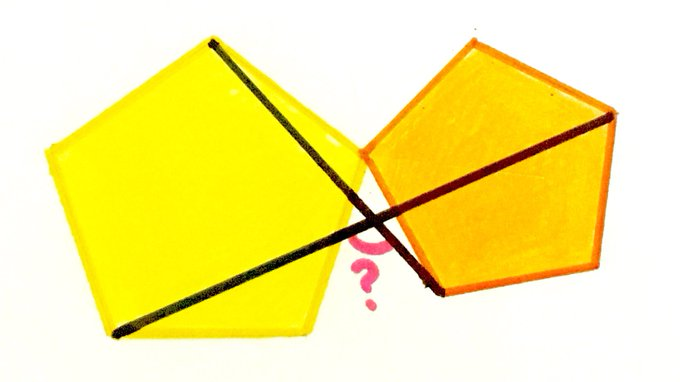
Two regular pentagons. What’s the angle?
Solution by Transformation and Similar Triangles
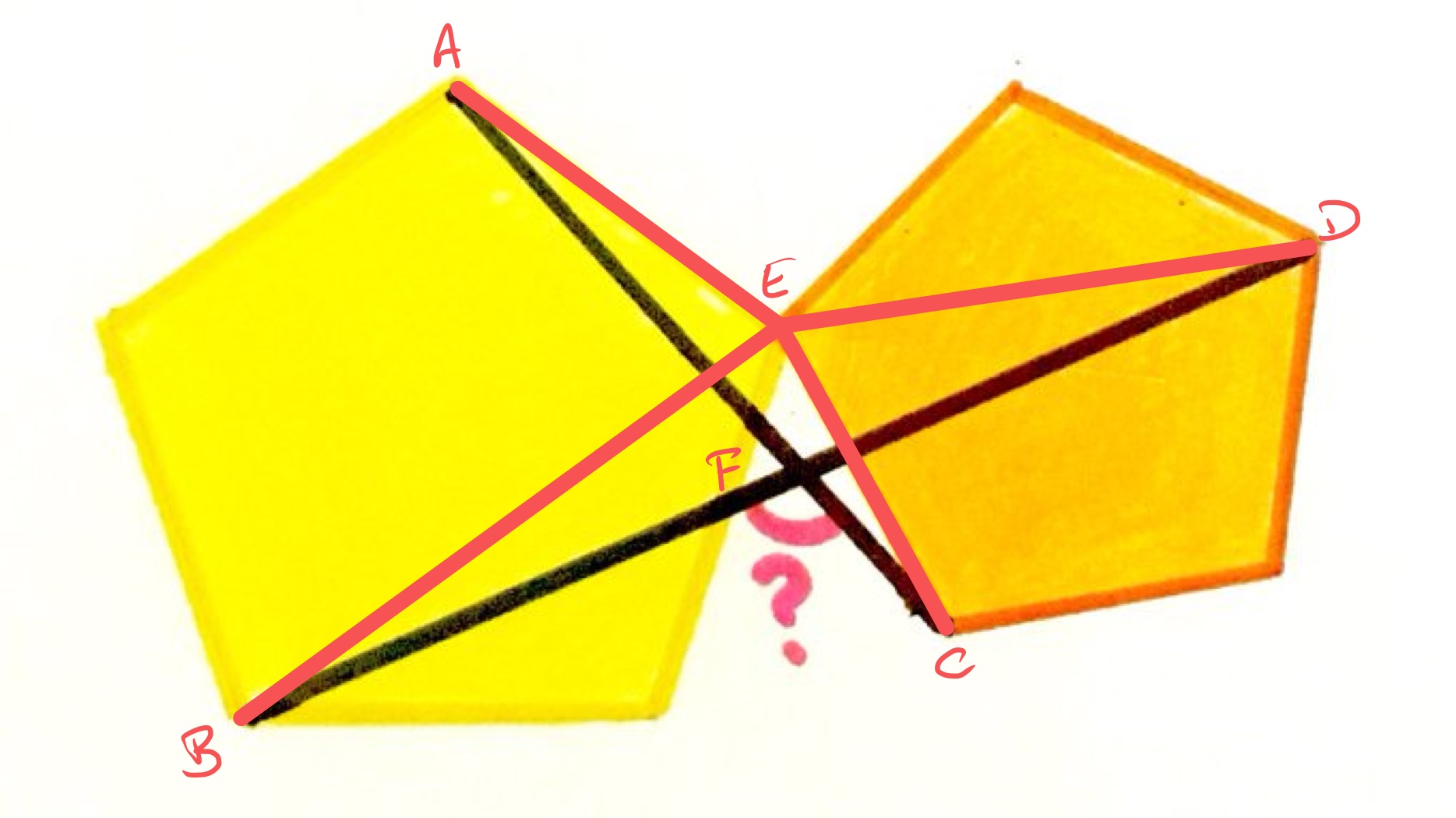
With the points labelled as above, rotation about point of angle followed by a uniform scaling by the golden ratio also from takes to and to . Therefore, applying this transformation to triangle produces triangle and so these triangles are similar. This means that is obtained from by rotation of (and scaling) and so angle is . The requested angle is therefore .
Solution by Invariance Principle
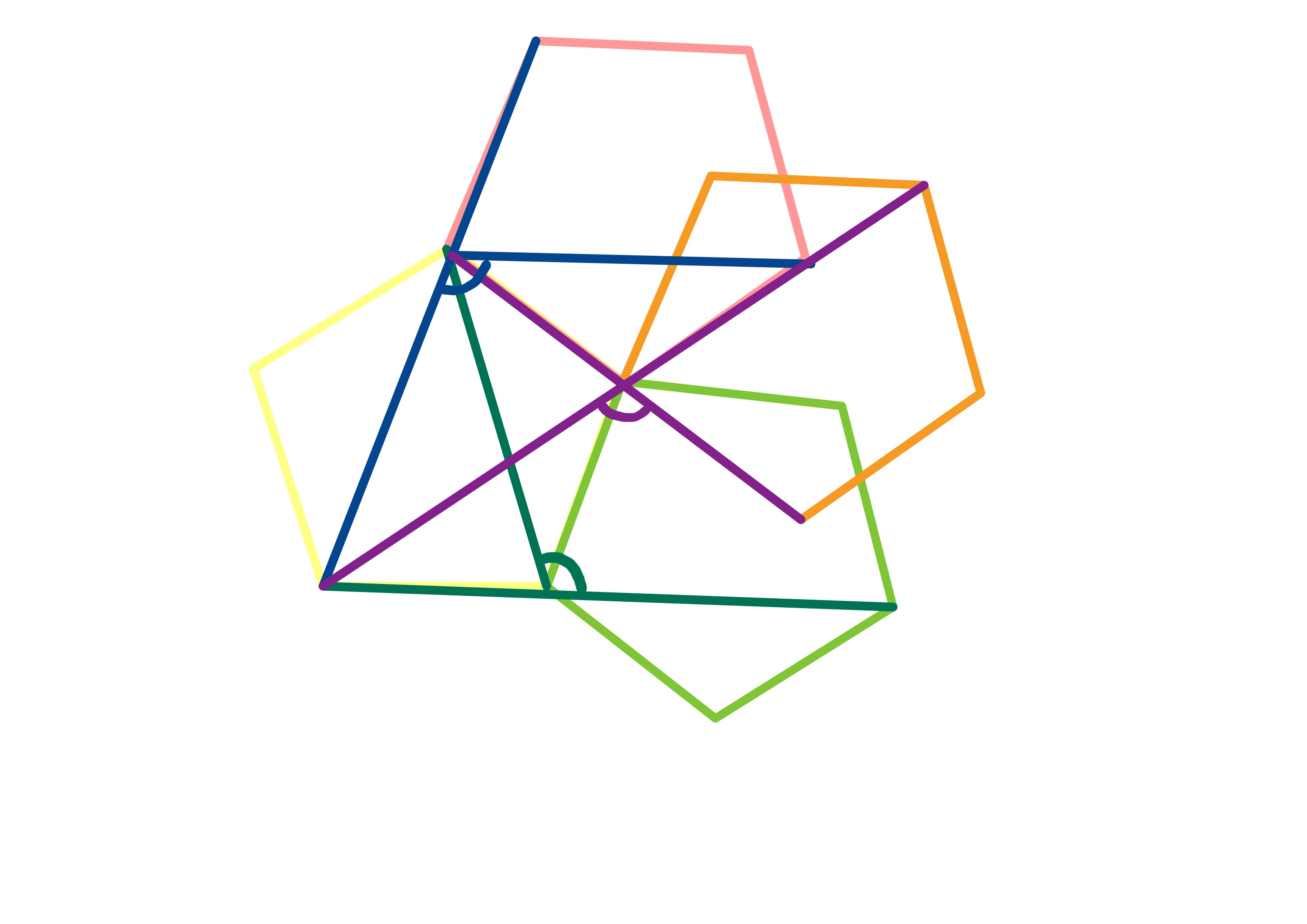
The above diagram contains three special cases of the diagram. The yellow pentagon is common to all three. The pink and green each share an edge with the yellow, while the orange is such that at the common vertex then the sides of it and the yellow form straight lines. Using the angles in a regular pentagon, the required angle can be seen to be .