Notes
tilted triangles solution

Solution to the Tilted Triangles Puzzle

Solution by Angle at the Centre is Twice the Angle at the Circumference

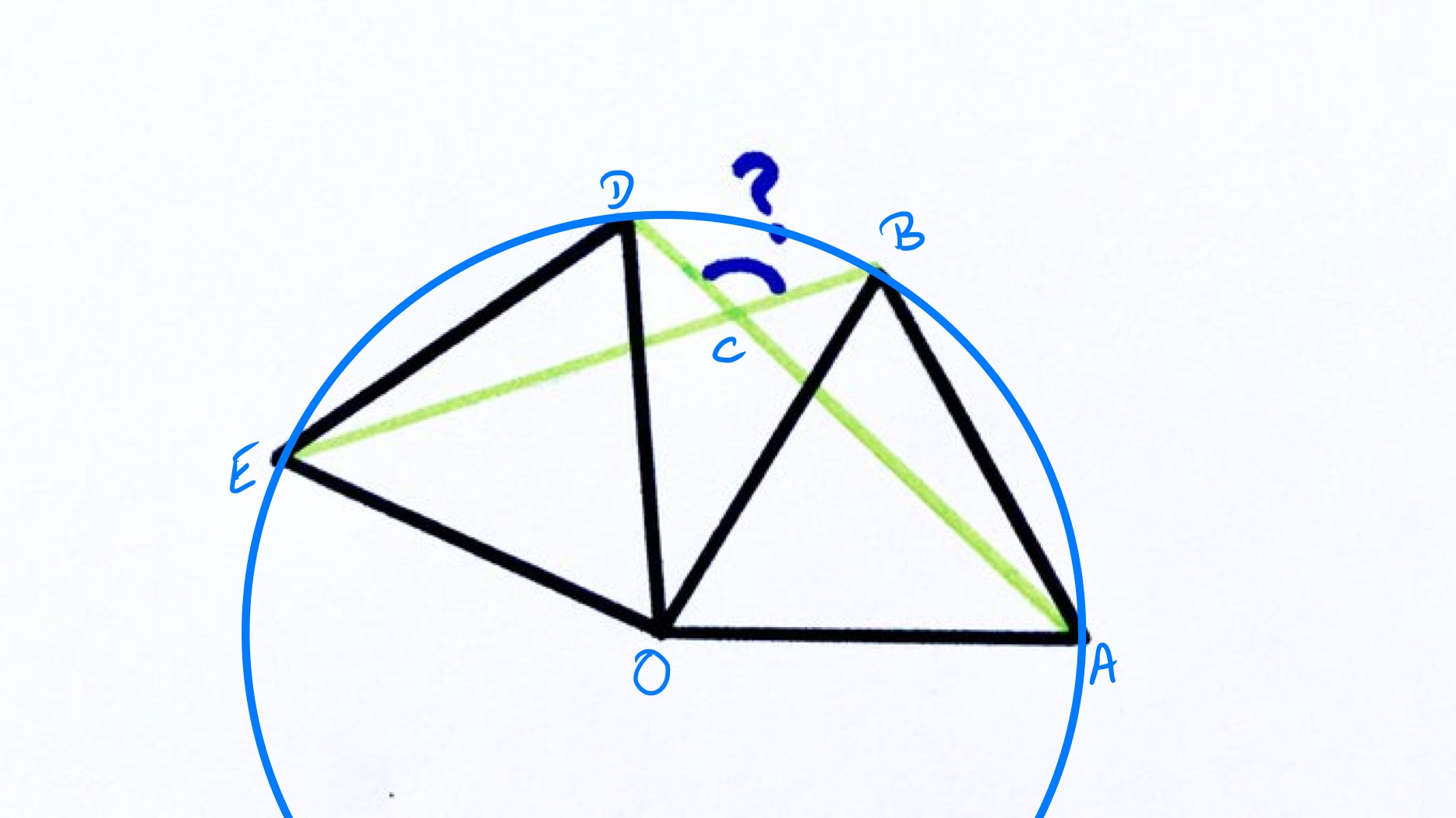
With the points labelled as in the above diagram, since the triangles are equilateral and congruent the lengths of $O E$, $O D$, $O B$, and $O A$ are all the same so the circle centred on $O$ that passes through $E$ also passes through $D$, $B$, and $A$.

Since the angle at the centre is twice the angle at the circumference, the reflex angle $E \hat{O} A$ is twice angle $E \hat{B} A$. The same argument shows that angle $B \hat{O} D$ is twice angle $B \hat{A} D$. Adding together angles $B \hat{A} D$ and $E \hat{B} A$ therefore gives half of the sum of $B \hat{O} D$ and $E \hat{O} A$. Angles $D \hat{O} E$ and $A \hat{O} B$ are both the interior angle of an equilateral triangle so are $60^\circ$. Since the angles at a point add up to $360^\circ$, this means that angles $B \hat{A} D$ and $E \hat{B} A$ add up to $120^\circ$. Then as the angles in a triangle add up to $180^\circ$, angle $A \hat{C} B$ is $60^\circ$ and so $B \hat{C} D$ is $120^\circ$.