Notes
three overlapping hexagons solution
Solution to the Three Overlapping Hexagons Puzzle
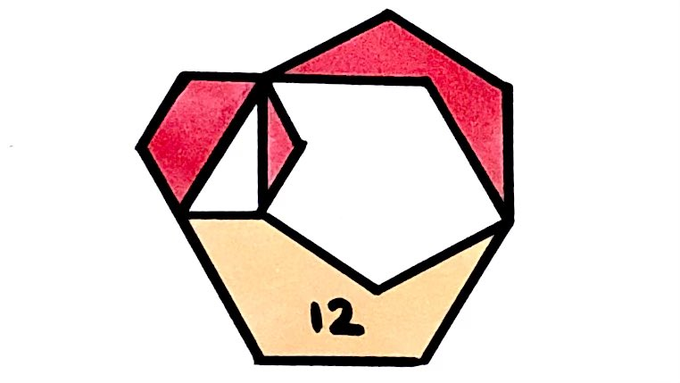
Here are three overlapping regular hexagons. What’s the total red area?
Solution by Properties of a Regular Hexagon

In the above diagram, the diagonal of the smallest hexagon is the same length as the side of the largest. From lengths in a regular hexagon, this means that the side length of the smallest hexagon is half that of the largest, so its total area is one quarter that of the largest.
The upper right red region can be dissected by extending the sides of the largest hexagon. Region fits into the smallest hexagon to fill it, while region is congruent to region and region can be moved to the right of to show that and have the same area as half the smallest hexagon. Therefore the area of the red region is of the smallest hexagon, so is of the largest.
The regions and also fit into the divot of the yellow region, completing that to half the largest hexagon. The regions and are, from above, in area half the smallest hexagon, which is a quarter the are of the largest. So and , and hence the divot from the yellow region, is th of the area of the largest hexagon.
The yellow region therefore comprises of the largest hexagon.
Therefore the red region has area .
Solution by Dissection

In the above diagram, the red regions can be dissected and rearranged to fit exactly into the yellow region as indicated.