Notes
stacked shapes solution

Stacked Shapes

Solution by Lengths in an Equilateral Triangle

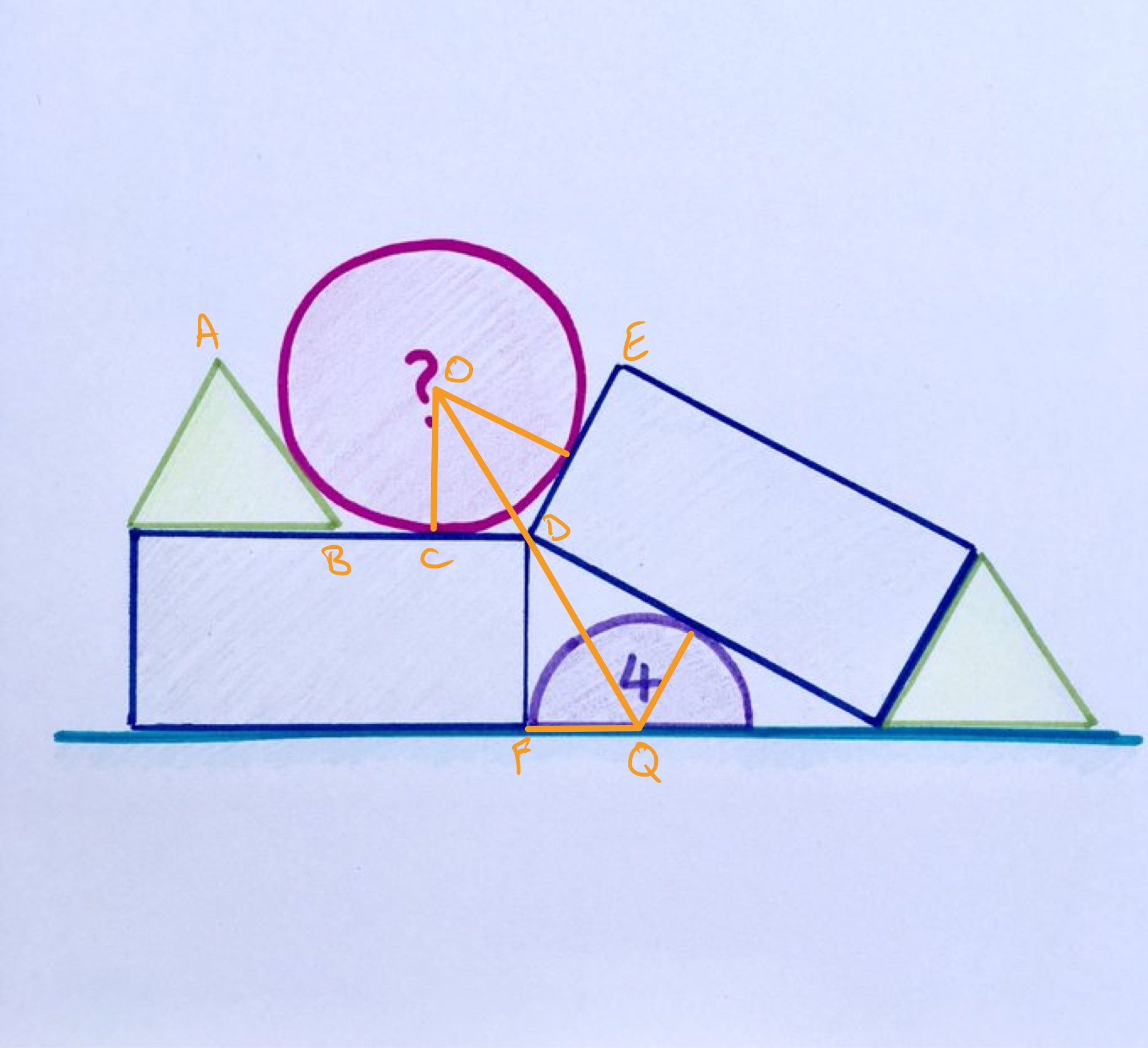
As clarified in the twitter thread, shapes of the same colour are congruent. The side of the equilateral triangle has the same length as the short side of the rectangle, and so half the length of the long side. Let $a$ be this length. Then $B D$ also has length $a$. Angles $D \hat{B} A$ and $E \hat{D} B$ are both the exterior angles of an equilateral triangle so are both $120^\circ$. Angle $O \hat{D} C$ is half that angle, so triangle $O D C$ is half an equilateral triangle. From the relationships between lengths in an equilateral triangle, $O C$ has length $\frac{\sqrt{3}}{2} a$. Triangle $D Q F$ is also half an equilateral triangle, since $D F$ has length $a$, $F Q$ has length $\frac{1}{\sqrt{3}} a$. The area of the semi-circle is then $\frac{1}{6} \pi a^2$ so $\pi a^2 = 24$. The area of the circle is therefore $\frac{3}{4} \pi a^2 = 18 \pi$.