Notes
shaded spikes in a hexagon solution

Solution to the Shaded Spikes in a Hexagon Puzzle

Solution by Properties of a Regular Hexagon and the Area of a Triangle

Consider the diagram labelled as below.

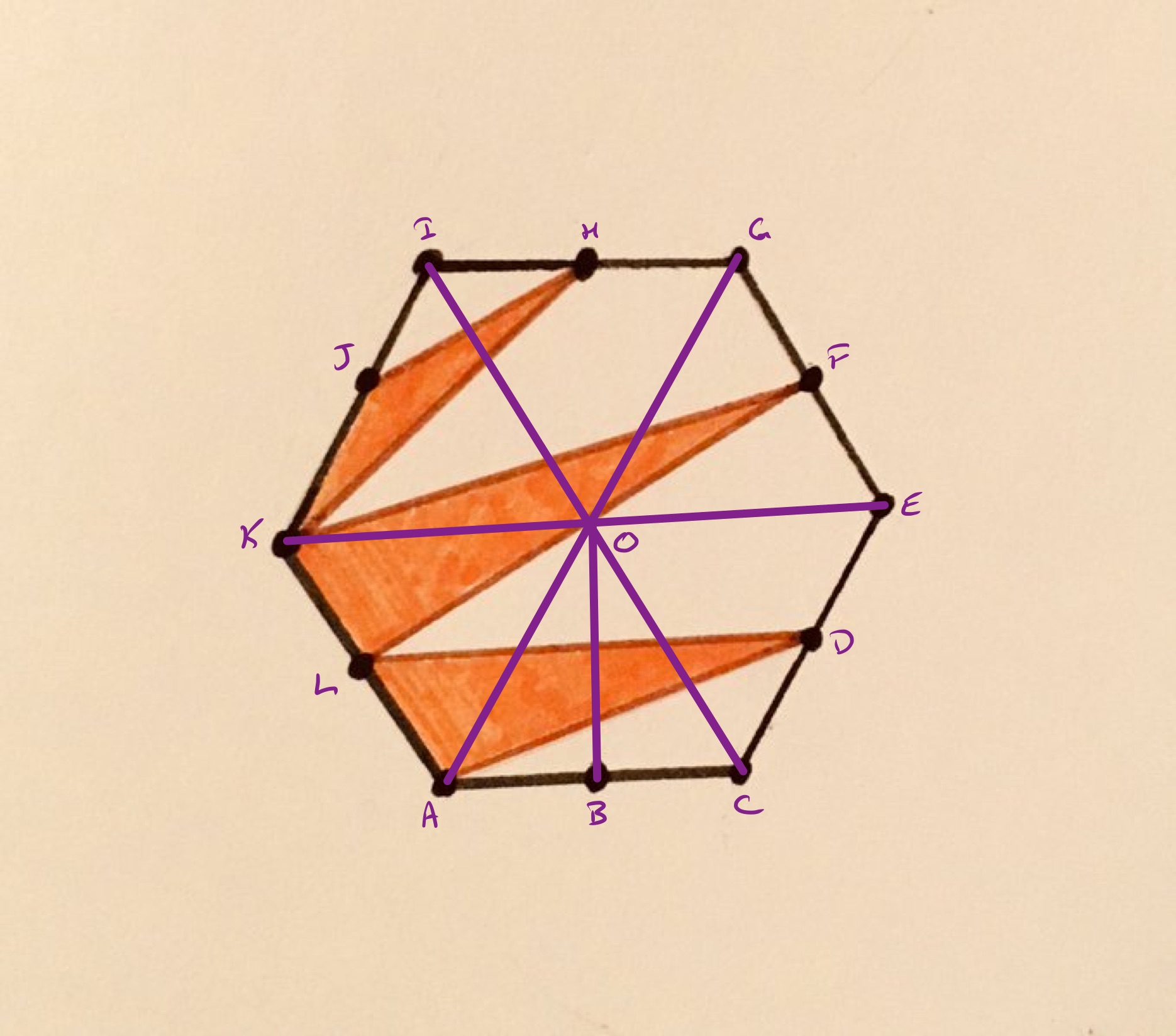
From the properties of a regular hexagon, the triangles with apex at $O$ and side one of those of the hexagon are all equilateral. Each has area $\frac{1}{6}$th of that of the hexagon.

Let $h$ be the height of one equilateral triangle, so the length of $O B$, and let $b$ be the base, so the length of $A C$. Then the area of the hexagon is $6 \times \frac{1}{2} b h = 3 b h$.

As the points $L$ and $D$ are the midpoints of their sides, the length of $L D$ is half way between that of $A C$, namely $b$, and of $K E$, namely $2 b$. So $L D$ has length $\frac{3}{2} b$. Similarly, the height of $A$ above the line $L D$ is $\frac{1}{2} h$, so the area of triangle $A D L$ is $\frac{1}{2} \times \frac{3}{2} b \times \frac{1}{2} h = \frac{3}{8} b h$.

The length of $L F$ is $2 h$ and of $K L$ is $\frac{1}{2} b$, so the area of triangle $L F K$ is $\frac{1}{2} \times \frac{1}{2} b \times 2 h = \frac{1}{2} b h$.

The height of $H$ above $K J$ is half of the height of $G$ (and of $O$) so is $\frac{1}{2} h$. Since $K J$ has length $\frac{1}{2} b$ this means that triangle $K H J$ has area $\frac{1}{2} \times \frac{1}{2} b \times \frac{1}{2} h = \frac{1}{8} b h$.

The total shaded area is therefore:

Since the area of the hexagon is $3 b h$ this means that one third of the hexagon is shaded.

Solution by Properties of a Regular Hexagon and Dissection

Consider the diagram labelled as below.

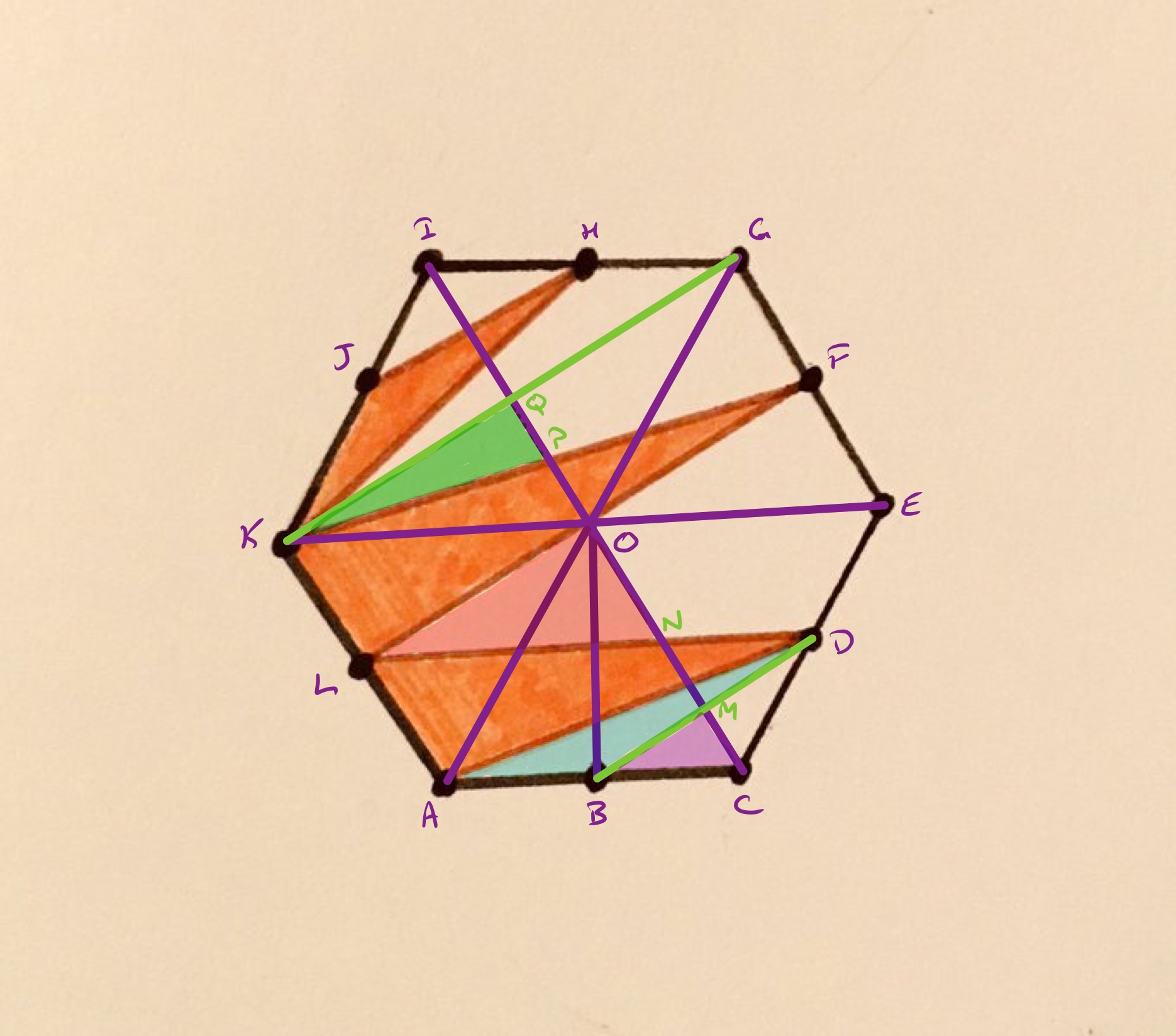
The (orange) shaded areas are cut and reassembled as follows.

1. Triangle $K H J$ is flipped and translated to $A B D$, where it fits exactly.
2. Triangle $M D N$ is rotated round to fit in $B C M$.
3. Triangle $O F P$ is rotated round to fit in $Q P K$.
4. Triangle $O Q K$ is translated to fit in $N O L$.

This leaves the shaded area being parallelogram $K A C O$ which consists of two of the six equilateral triangles that make up the hexagon. The shaded area is therefore one third of the total.