Notes
rectangle on a hexagon solution
Solution to the Rectangle on a Hexagon Puzzle
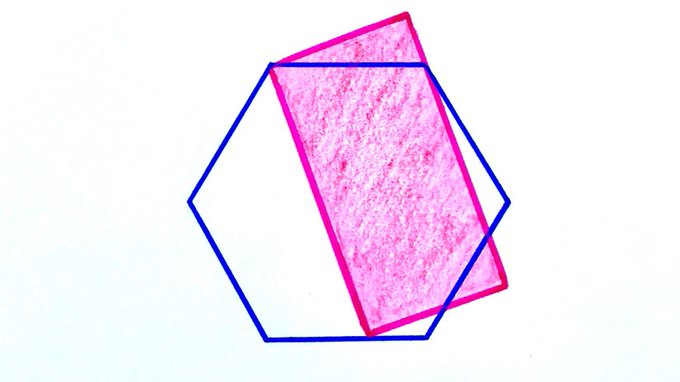
The area of the regular hexagon is . What’s the area of the rectangle?
Solution by Dissection
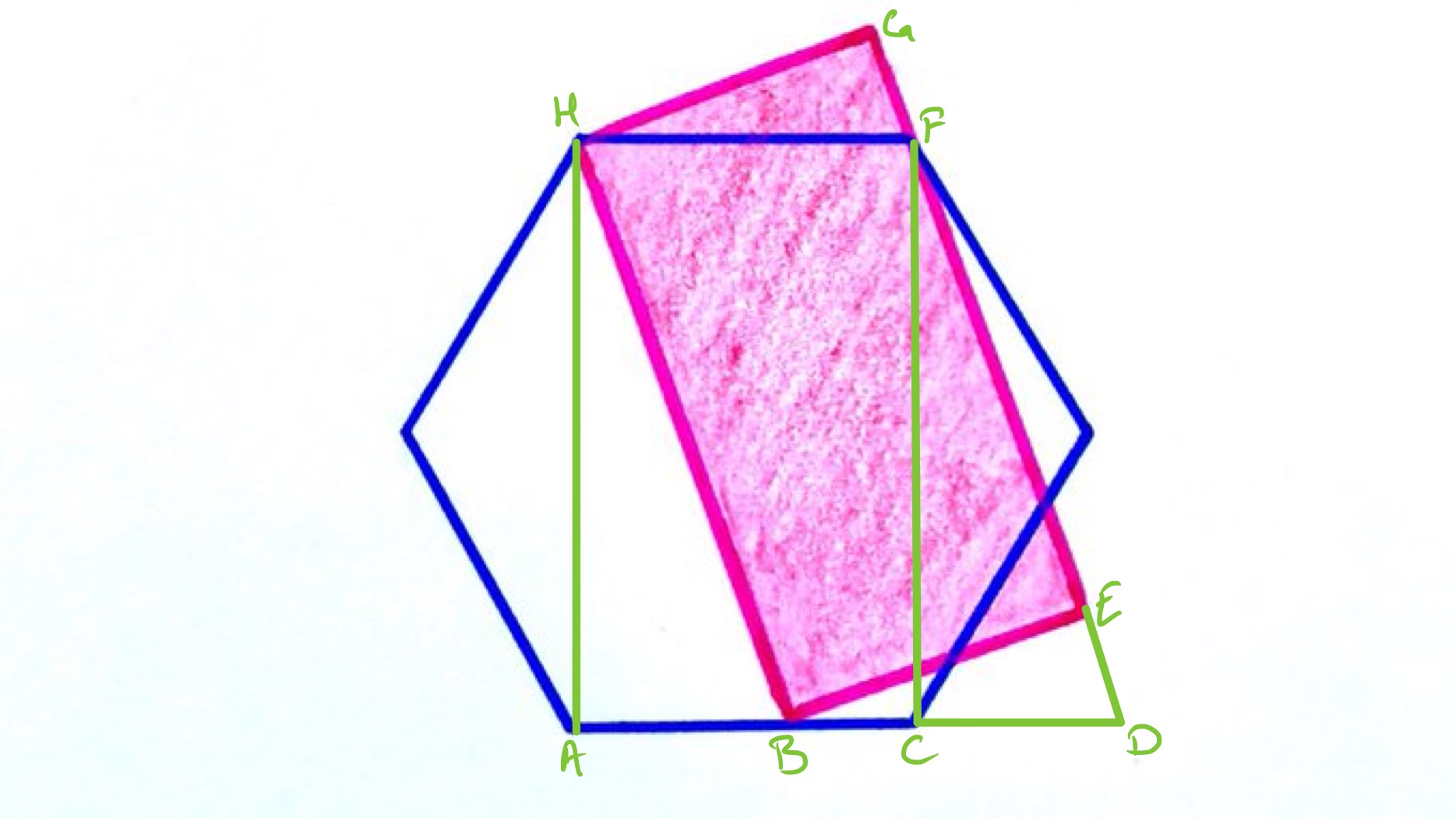
In the above diagram, triangles and are congruent so the rectangle has the same area as parallelogram . Then triangles and are congruent, so the rectangle has the same area as the rectangle . From the properties of a regular hexagon, this rectangle comprises four of the six equilateral triangles that make up the hexagon, so its area is .
Solution by Shearing
Shear the rectangle parallel to so that ends up at , then shear the resulting parallelogram parallel to so that ends up at . This shows that the shaded rectangle has the same area as .
Solution by Invariance Principle
The shaded rectangle can be drawn with at any point between and . In particular, it can be drawn with at which results in the rectangle .