Notes
isosceles and equilateral triangles solution

Solution to the Isosceles and Equilateral Triangles Puzzle

Solution by Direct Calculation

Consider the labelled diagram.

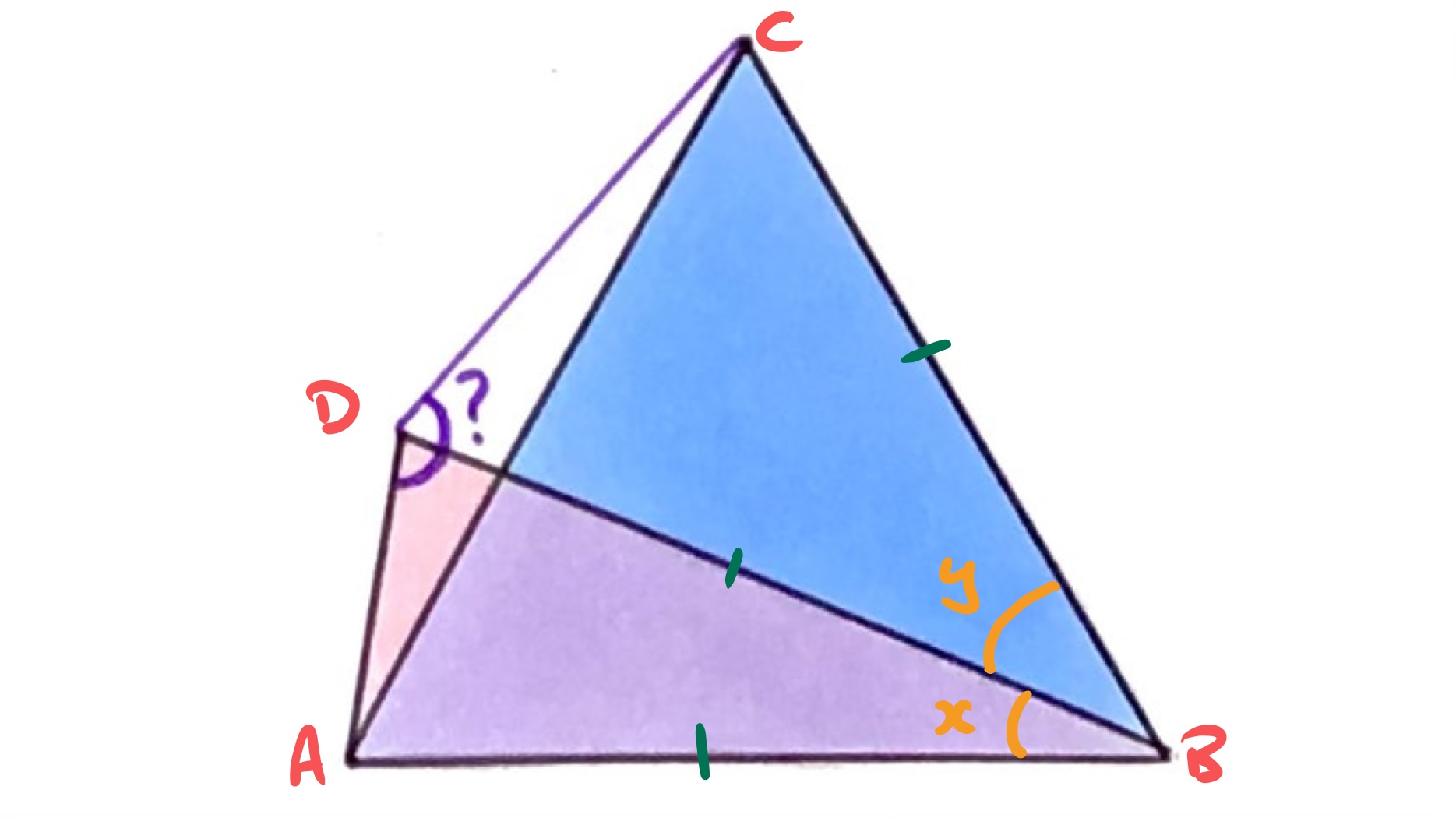
Let $x = A\hat{B} D$ and $y = D\hat{B} C$. Then $x + y = 60^\circ$ as the interior angle in an equilateral triangle.

As triangle $A B D$ is isosceles, angle $A \hat{D} B$ is half of $180^\circ - x$. Also, as it is isosceles then $D B = A B$, then as triangle $A B C$ is equilateral, $D B = C B$ so triangle $D B C$ is also isosceles. By the same reasoning, then, angle $B \hat{D} C$ is half of $180^\circ - y$. Putting these together, angle $A \hat{D} C$ is:

Solution using Circles

Since triangle $A B D$ is isosceles and $A B C$ is equilateral, drawing a circle centred on $B$ with radius $A B$ passes through both $C$ and $D$.

Using the angle on the circumference is half the angle at the centre then angle $A \hat{D} C$ is half of angle $A \hat{B} C$, where the latter is the reflex angle. Hence: