Notes
angles in two circles solution
Solution to the Angles in Two Circles Puzzle
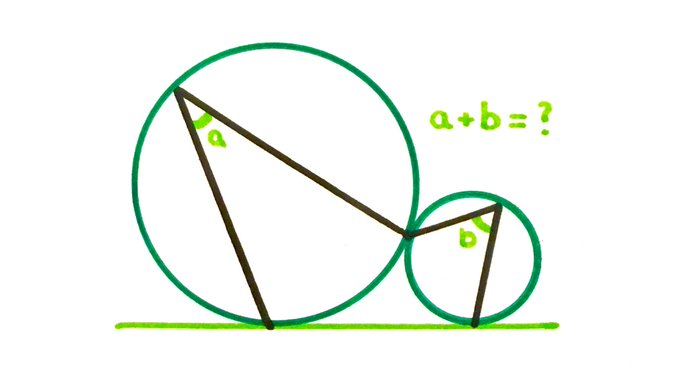
What’s the sum of the two marked angles?
Solution by the Alternate Segment Theorem and Angles in a Triangle

With the points labelled as above, the alternate segment theorem gives that both angles and are both while and are both . As angles in a triangle add up to , considering triangle shows that , and so .