Notes
a hexagon and a nonagon solution

Solution to the Puzzle

Solution by Angles in a triangle

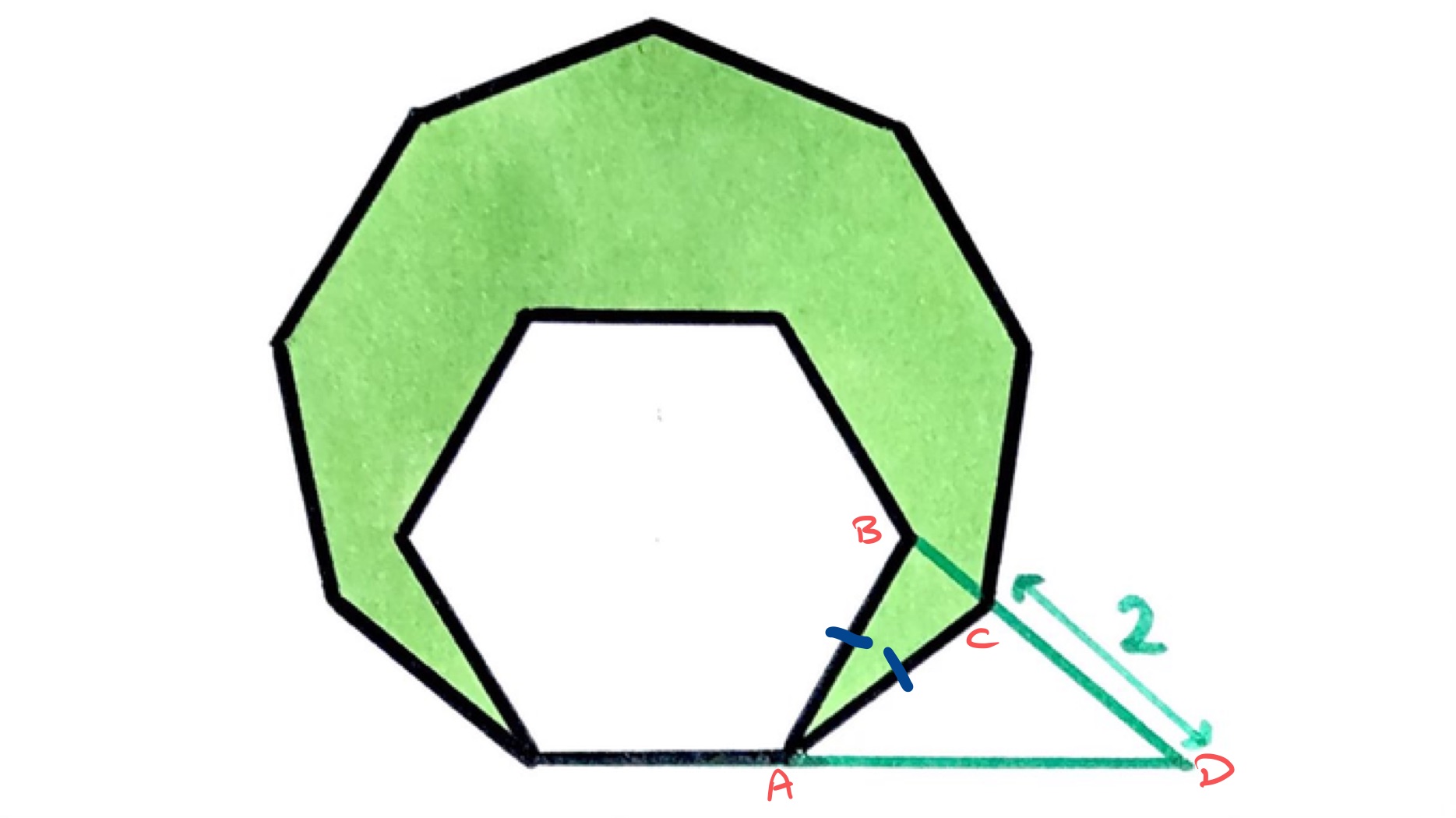
As the polygons are regular and share a side, all their sides are the same length. In particular, in the above diagram then $A B$ and $A C$ have the same length so triangle $A B C$ is isosceles. The angle at $A$ is the difference between the exterior angles of a hexagon and nonagon, which is $60^\circ - 40^\circ = 20^\circ$. This means that angle $A \hat{C} B = 80^\circ$ and so angle $A \hat{C} D = 100^\circ$ since angles at a point on a straight line add up to $180^\circ$.

Angle $C \hat{A} D$ is the exterior angle of a nonagon, which is $40^\circ$, so angle $C \hat{D} A = 180^\circ - 100^\circ - 40^\circ = 40^\circ$. This means that triangle $A C D$ is isosceles with $C A$ and $C D$ being the same length. Since $C D$ has length $2$, this means that $C A$ also has length $2$ and so the side lengths of the polygons are $2$.

The shaded area is bounded by $5$ edges from the hexagon and $8$ edges from the nonagon so its perimeter is $2 \times (5 + 8) = 26$.