Notes
a circle and two semi-circles in two hexagons solution

Solution to the Circle and Two Semi-Circles in Two Hexagons Puzzle

Solution by Angles in an Equilateral Triangle and Regular Hexagon

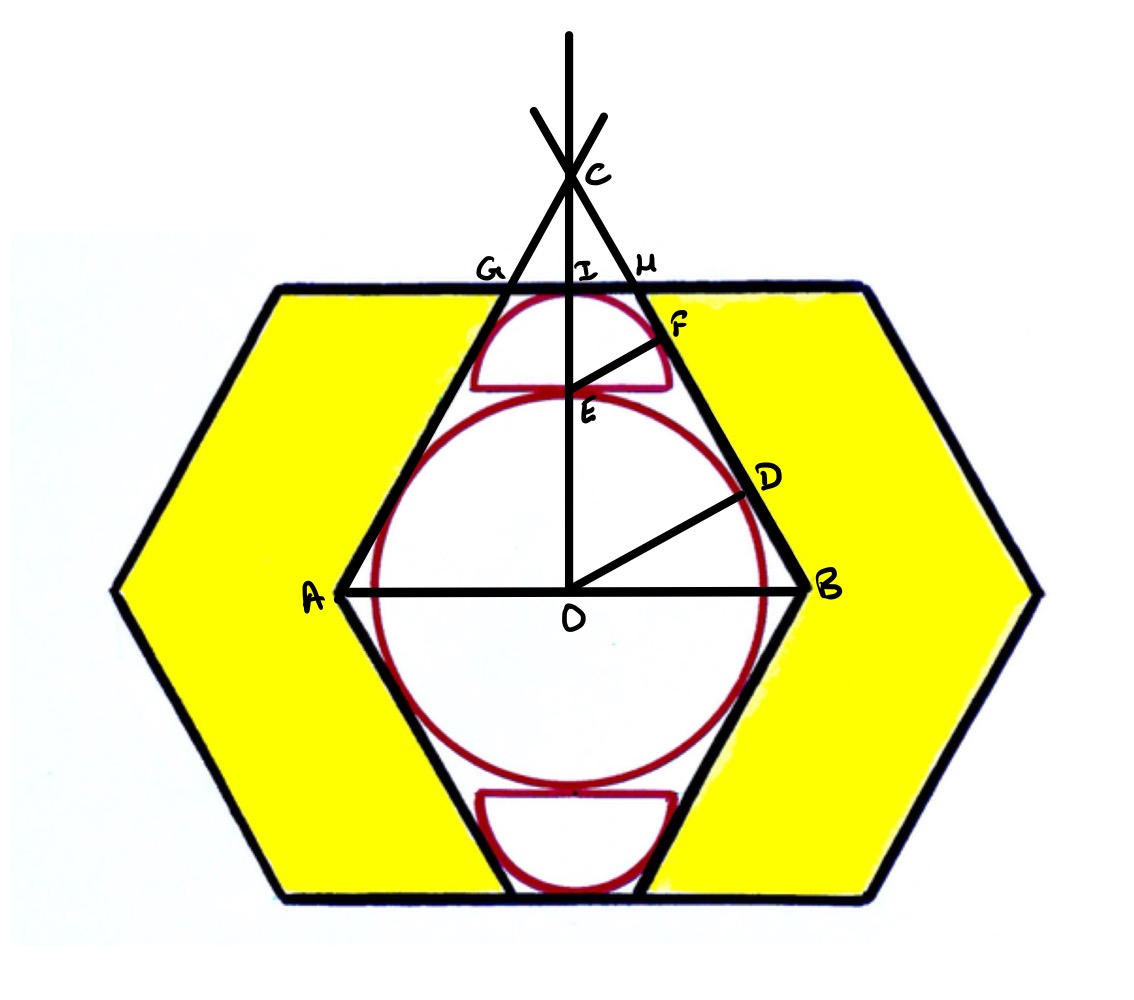
In the above diagram, the edges $A G$ and $B H$ are extended to meet at $C$. The point $O$ is the centre of the larger circle and $E$ of the upper semi-circle.

Angles $O \hat{A} G$ and $H \hat{B} O$ are both $60^\circ$ so triangle $A B C$ is an equilateral triangle. Angle $C \hat{D} O$ is a right-angle as it is the angle between a radius and tangent and angle $O \hat{C} D$ is half of $60^\circ$, so triangle $O D C$ is half an equilateral triangle, so the length of $O D$ is half that of $O C$. Hence the length of $E C$ is the same as that of $O D$. A similar argument applies to the triangle $E F C$ so the length of $I C$ is the same as that of $E F$. Putting that together shows that the length of $I C$ is a third of that of $O I$. So the length of $H G$ is a third of the length of $B H$, which is a side of one of the hexagons.

This means that dividing each side of the hexagon in three and drawing lines parallel to the sides is a subdivision of each of the regions.

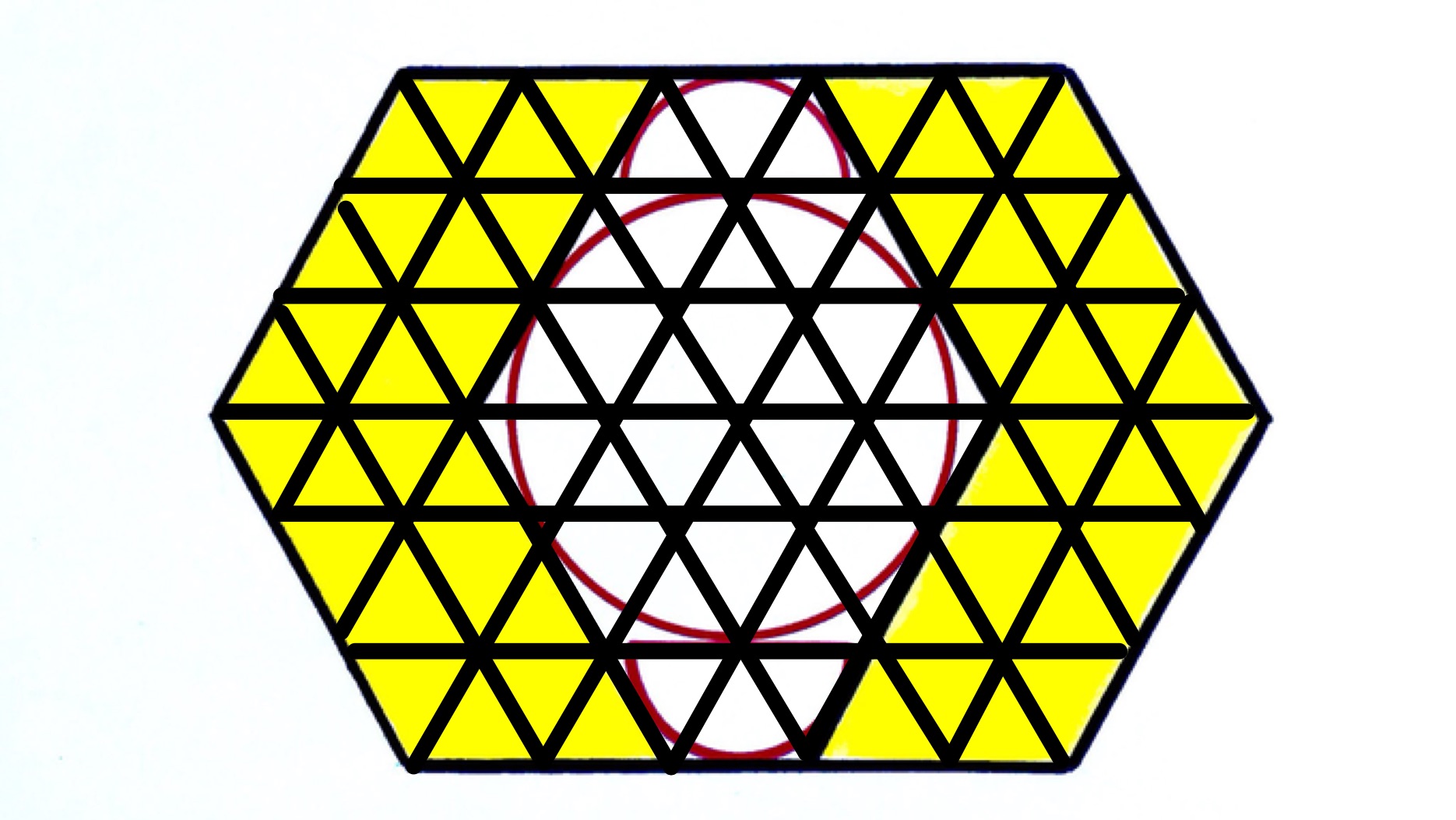
There are $24$ triangles in each of the yellow regions, and $30$ in the white region. So there are $78$ in total and $48$ that are yellow, so the yellow region is $\frac{48}{78} = \frac{24}{39}$ths of the total.