Notes
two triangles overlapping a rectangle solution

Solution to the Two Triangles Overlapping a Rectangle Puzzle

Solution by Lengths in an Equilateral Triangle

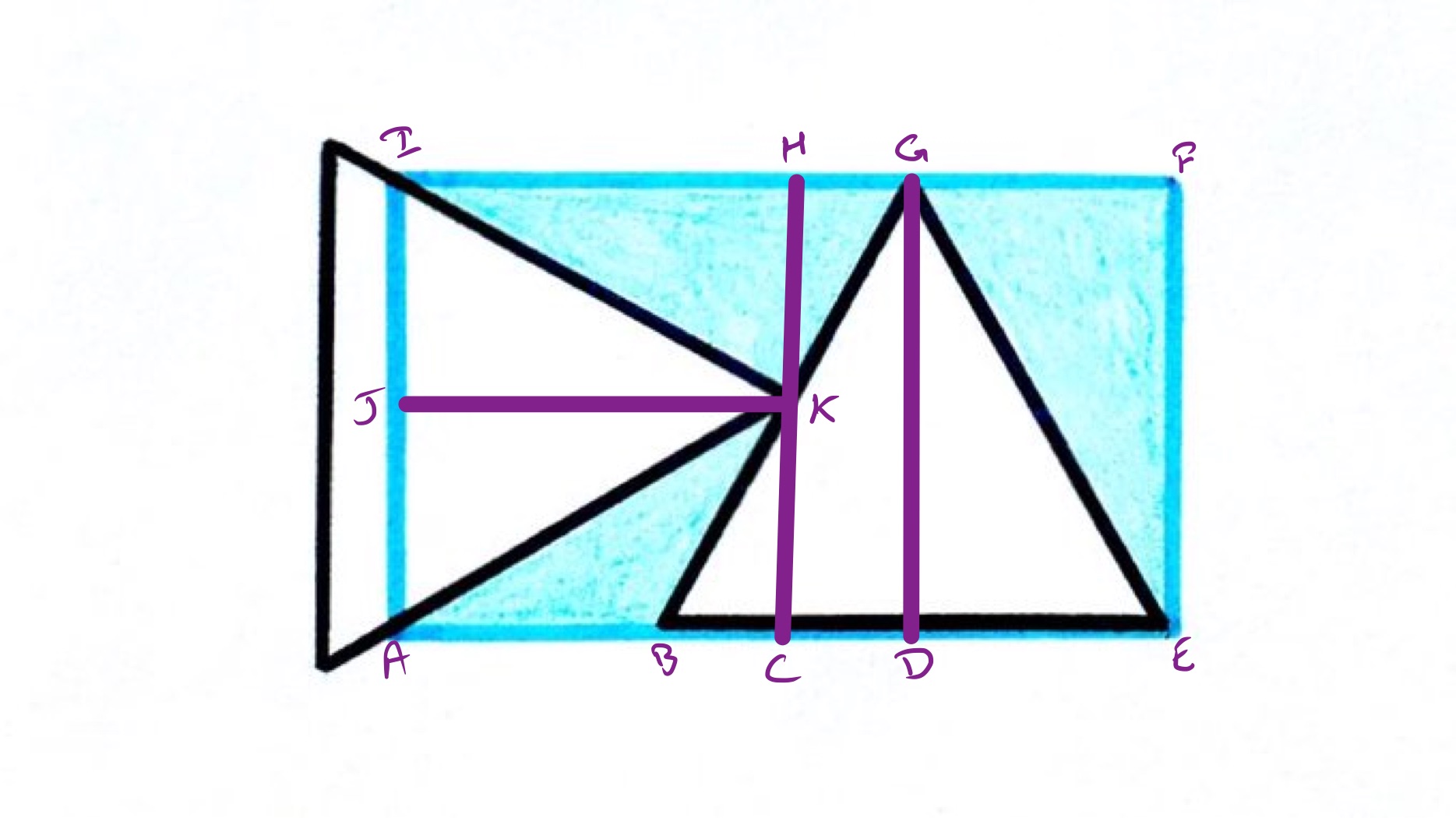
Let $x$ be the side length of the right-hand triangle, so the length of $B E$. From lengths in an equilateral triangle, the length of $G D$ is $\frac{\sqrt{3}}{2} x$. As this is the length of $I A$, the length of $J K$ is $\frac{\sqrt{3}}{2}$ times this, so is $\frac{3}{4} x$.

Since $K$ is horizontally aligned with $J$ which is the midpoint of $I A$, it is also the midpoint of $B G$ and so $C$ is the midpoint of $B D$. Therefore, $C E$ is $\frac{3}{4} x$ and so the width of the rectangle is $\frac{3}{2} x$.

The blue regions can be matched with the white (inside the rectangle) except for the strip between $H C$ and $G D$: triangle $I H K$ matches $I J K$, triangle $G F E$ matches $G D E$, the combination of triangle $K H G$ with $A B K$ matches $A K J$. Therefore the blue regions comprise half of the rectangle without the strip between $H C$ and $G D$. This strip has width $\frac{1}{4} x$, meaning that the rest of the rectangle (which is split between the white and blue regions) is equivalent to a rectangle with width $\frac{3}{2} x - \frac{1}{4} x = \frac{5}{4}x$ (and the same height as the main rectangle). Half of this is blue, so the blue regions are equivalent to a rectangle of width $\frac{5}{8}x$. Therefore the blue regions form $\frac{5}{8}x \div \frac{3}{2} x = \frac{5}{12}$ths of the rectangle.