Notes
two squares on a circle solution
Solution to the Two Squares on a Circle Puzzle
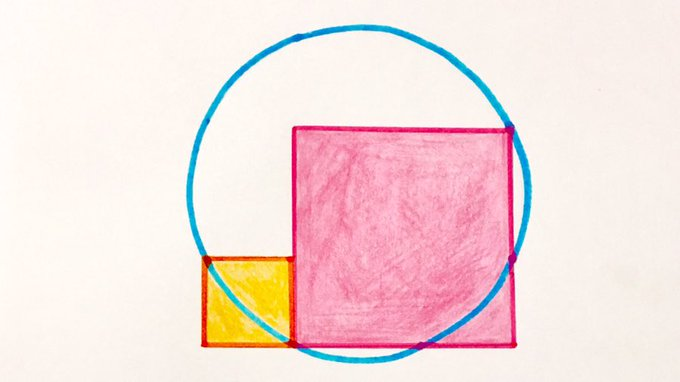
The radius of the circle is . What’s the combined area of the two squares?
Solution by Angle in a Semi-Circle and Pythagoras' Theorem
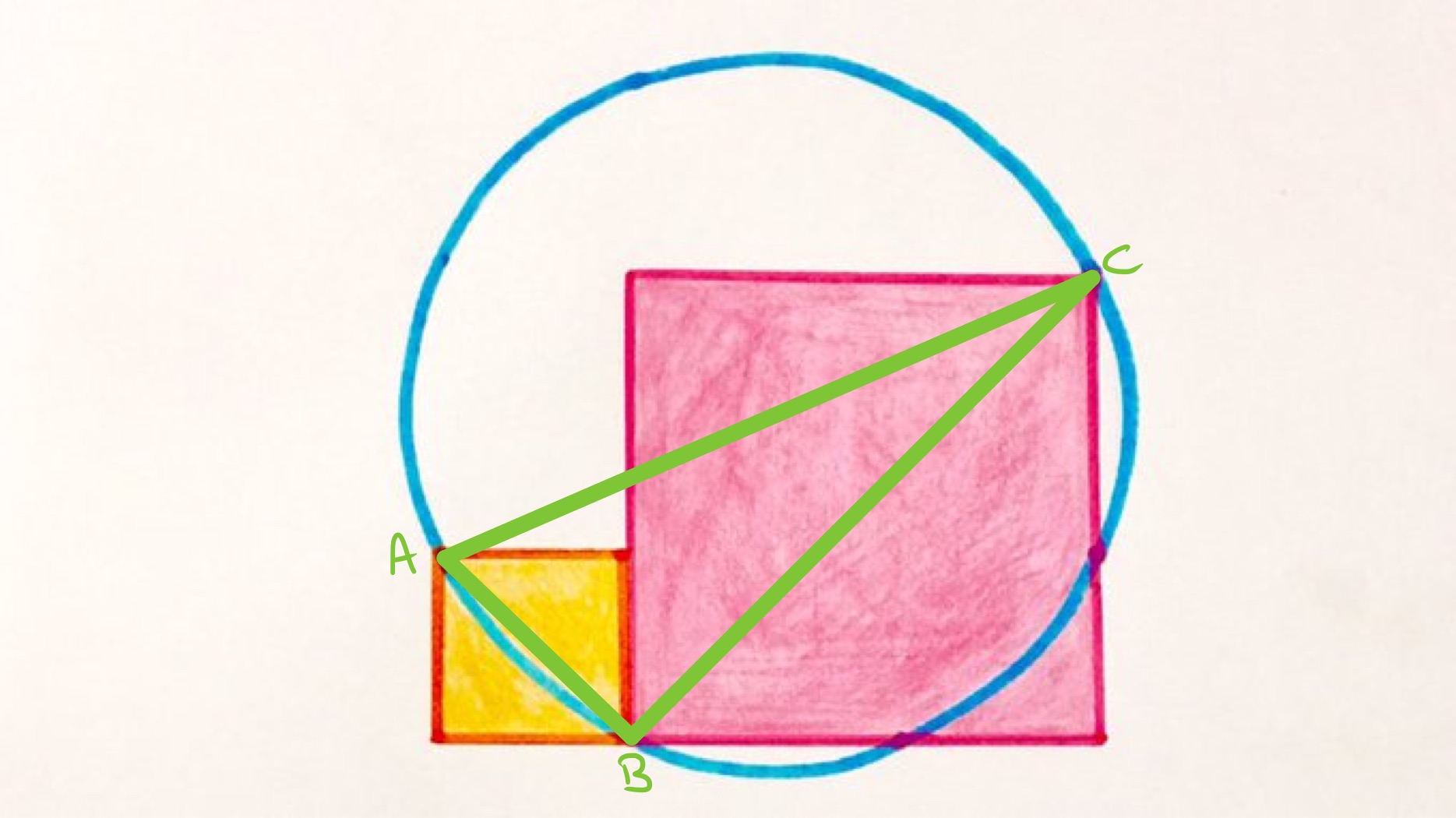
In the above diagram, angle is a right-angle, so as the angle in a semi-circle is a right-angle, must be a diameter of the circle. It therefore has length . Let the squares have side lengths and , with of the yellow square and of the pink. Then has length and has length . Applying Pythagoras' theorem to triangle shows that:
So . Since the areas of the squares are and , this shows that the combined area of the two squares is .