Notes
two squares inside a semi-circle solution
Solution to the Two Squares Inside a Semi-Circle Puzzle
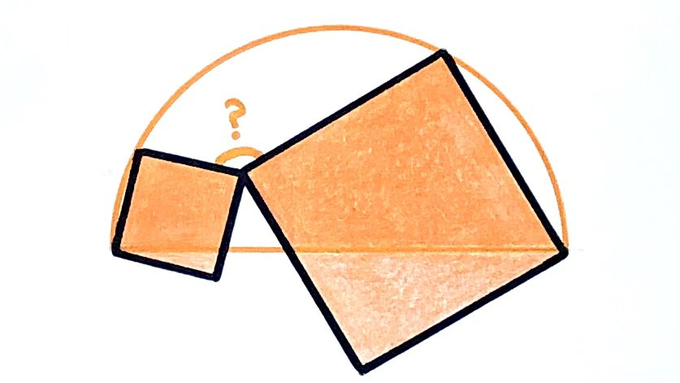
Two squares inside a semicircle. What’s the angle?
Solution by angle in a semi-circle

Consider the diagonals of the squares, as in the above diagram. The claim is that the lines and are straight lines. To see this, consider the triangle . The point is on the circumference of the circle of which is a diameter, so by angles in a semi-circle, angle is a right-angle. Since angle is also a right-angle, the line segments and must overlap and hence is a straight line. The same argument shows that is a straight line.
This means that angle , and then since is the diagonal of a square, leading to .