Notes
two semi-circles inside a semi-circle ii solution

Solution to the Two Semi-Circles Inside a Semi-Circle II Puzzle

Solution by Angle Between a Radius and Tangent, Pythagoras' Theorem, Angle in a Semi-Circle, and Similar Triangles

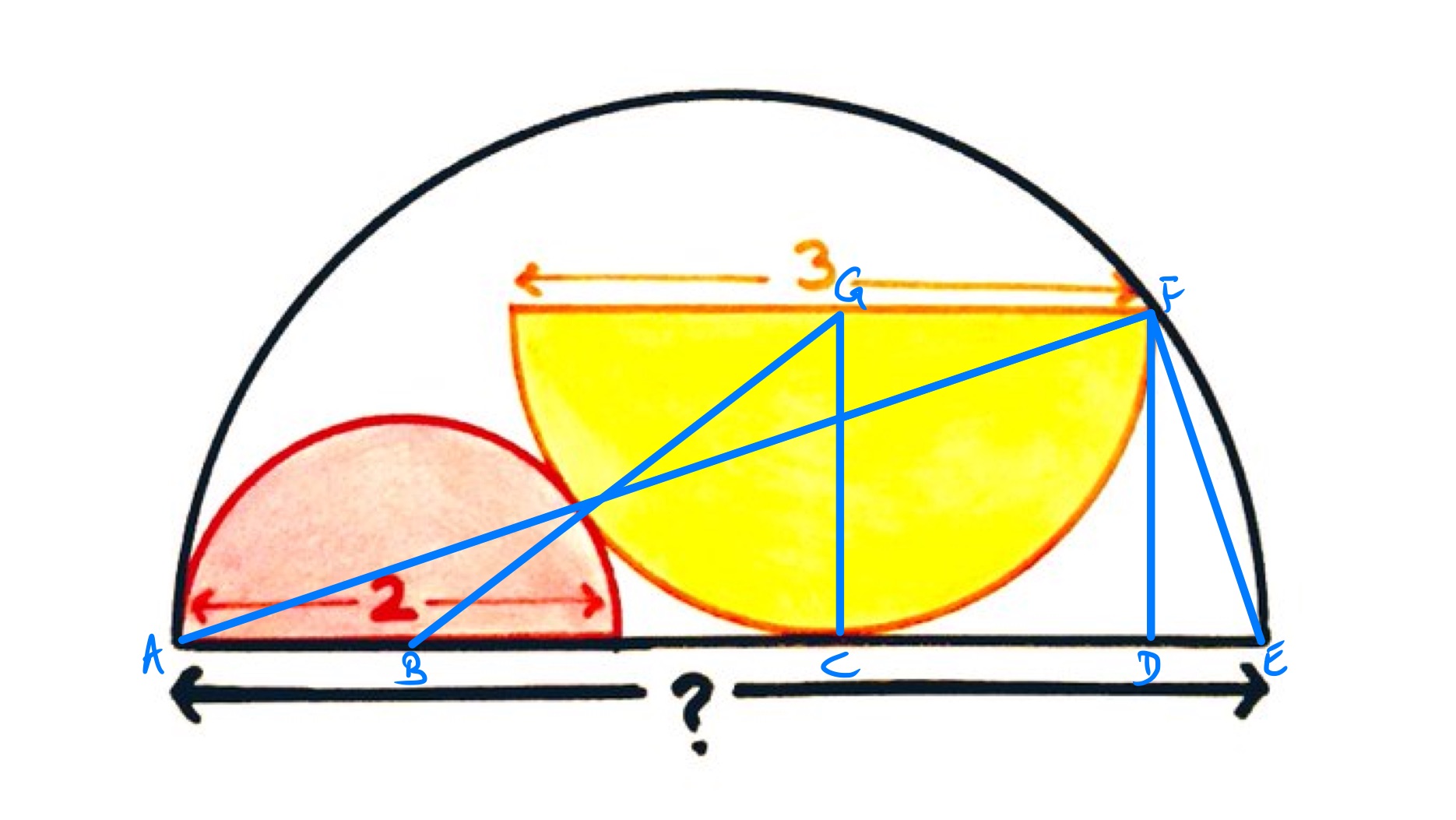
With the points labelled as above, $B$ and $G$ are the centres of their respective circles and $D$ is directly below $F$.

The length of $B G$ is $1 + 1.5 = 2.5$ and of $G C$ is $1.5$. As $A E$ is tangential to the yellow semi-circle at $C$, angle $G \hat{C} A$ is the angle between a radius and tangent so is a right-angle. Therefore, triangle $B C G$ is a right-angled triangle. Applying Pythagoras' theorem shows that $B C$ has length $2$. The full length of $A D$ is then $1 + 2 + 1.5 = 4.5$.

Angle $A \hat{F} E$ is the angle in a semi-circle so is a right-angle. Therefore angles $A \hat{F} D$ and $D \hat{F} E$ add up to $90^\circ$ and so triangles $A D F$ and $F D E$ are similar. As $F D$ has length $1.5$ and $A D$ length $4.5$, the length of $D E$ is one third of that of $F D$ and so is $.5$. Therefore $A E$ has length $5$.