Notes
two semi-circles in a square solution

Solution to the Two Semi-Circles in a Square Puzzle

Solution by Angle Between a Radius and Tangent, Pythagoras' Theorem, Angle in a Semi-Circle, and Similar Triangles

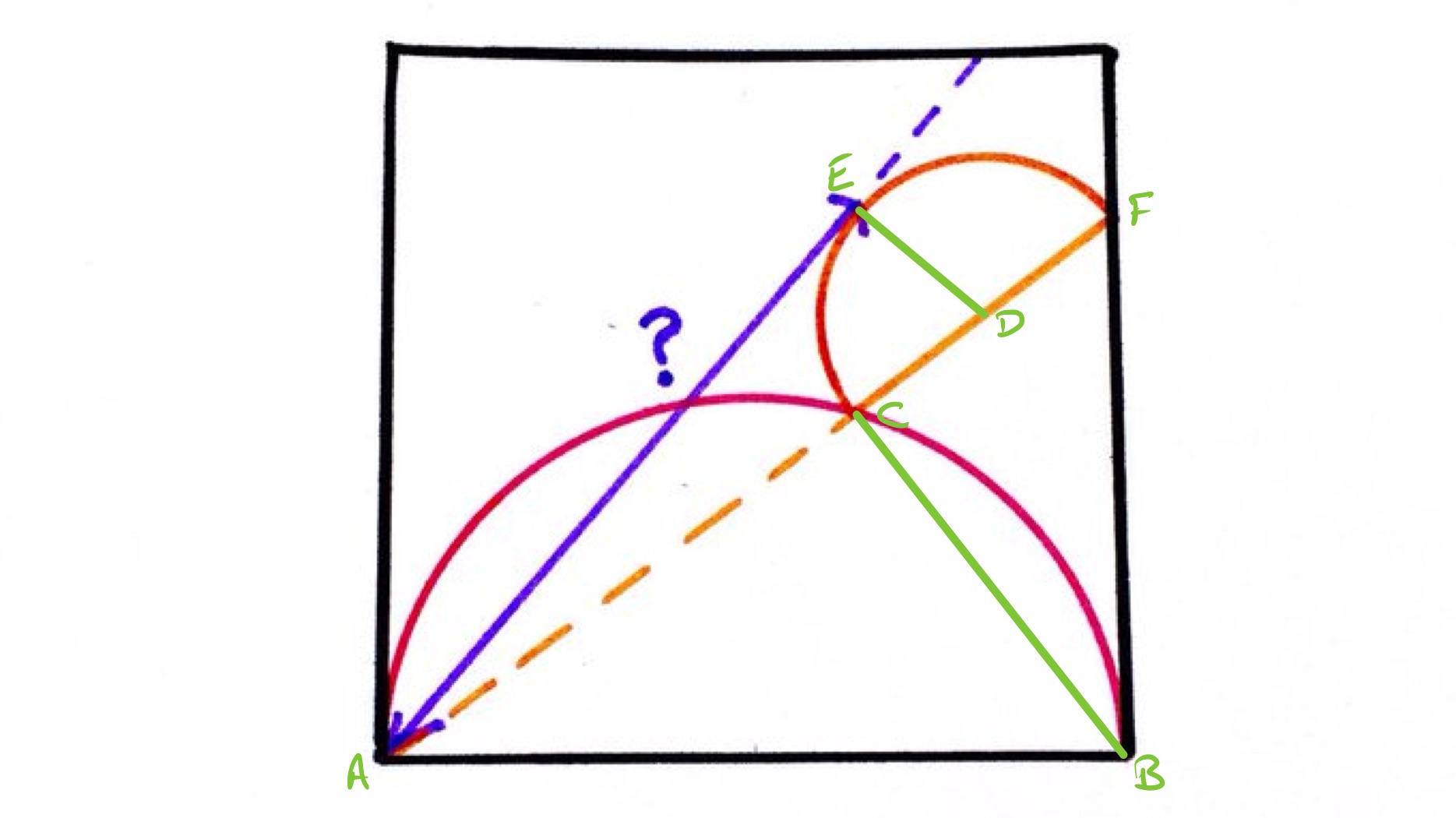
With the points labelled as in the above diagram, let $A C$ have length $a$, $B C$ have length $b$, and let $r$ be the radius of the smaller semi-circle. Let $c$ be the length of $A E$. As the square has area $100$, it has side length $10$.

As the angle between a radius and tangent is $90^\circ$, triangle $A E D$ is right-angled so Pythagoras' theorem applies and shows that:

So $c^2 = a^2 + 2 a r$.

Angle $A \hat{C} B$ is $90^\circ$ as it is the angle in a semi-circle, so triangles $A C B$ and $A B F$ are similar. This means that the ratio of the lengths of $A B$ to $A C$ is equal to that of $A F$ to $A B$. That is, $\frac{10}{a} = \frac{a + 2 r}{10}$. This rearranges to $10^2 = a^2 + 2 a r$ and so $c^2 = 10^2$.

The missing length is therefore $10$.