Notes
two rectangles overlapping a square solution

Solution to the Two Rectangles Overlapping a Square Puzzle

Solution by Dissection and Area of a Rectangle

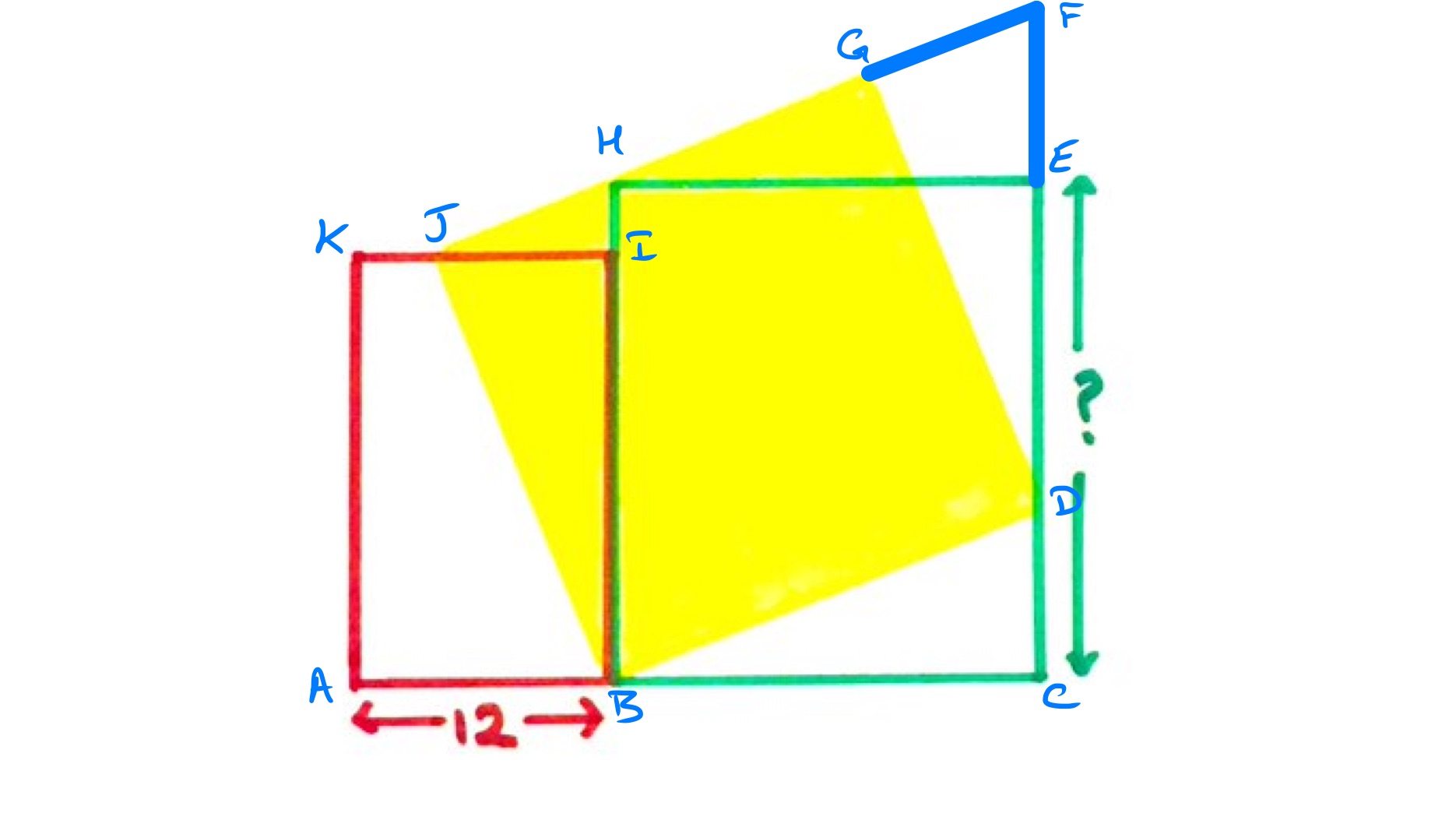
Triangle $J H B$ is congruent to triangle $G F D$, then triangle $H F E$ is congruent to triangle $B D C$. These establish that the green rectangle has the same area as the yellow square. (This can also be established by shears: first, parallel to $B D$; second, parallel to $B H$.)

Triangle $B C D$ is congruent to triangle $B I J$, meaning that the length of $B C$ is the length of $B I$. Therefore, since the green rectangle has twice the area of the red, the length of $B H$ must be twice that of $B A$, namely $24$.