Notes
two quarter circles in a semi-circle solution

Solution to the Two Quarter Circles in a Semi-Circle Puzzle

Solution by Similar Triangles and Properties of Chords

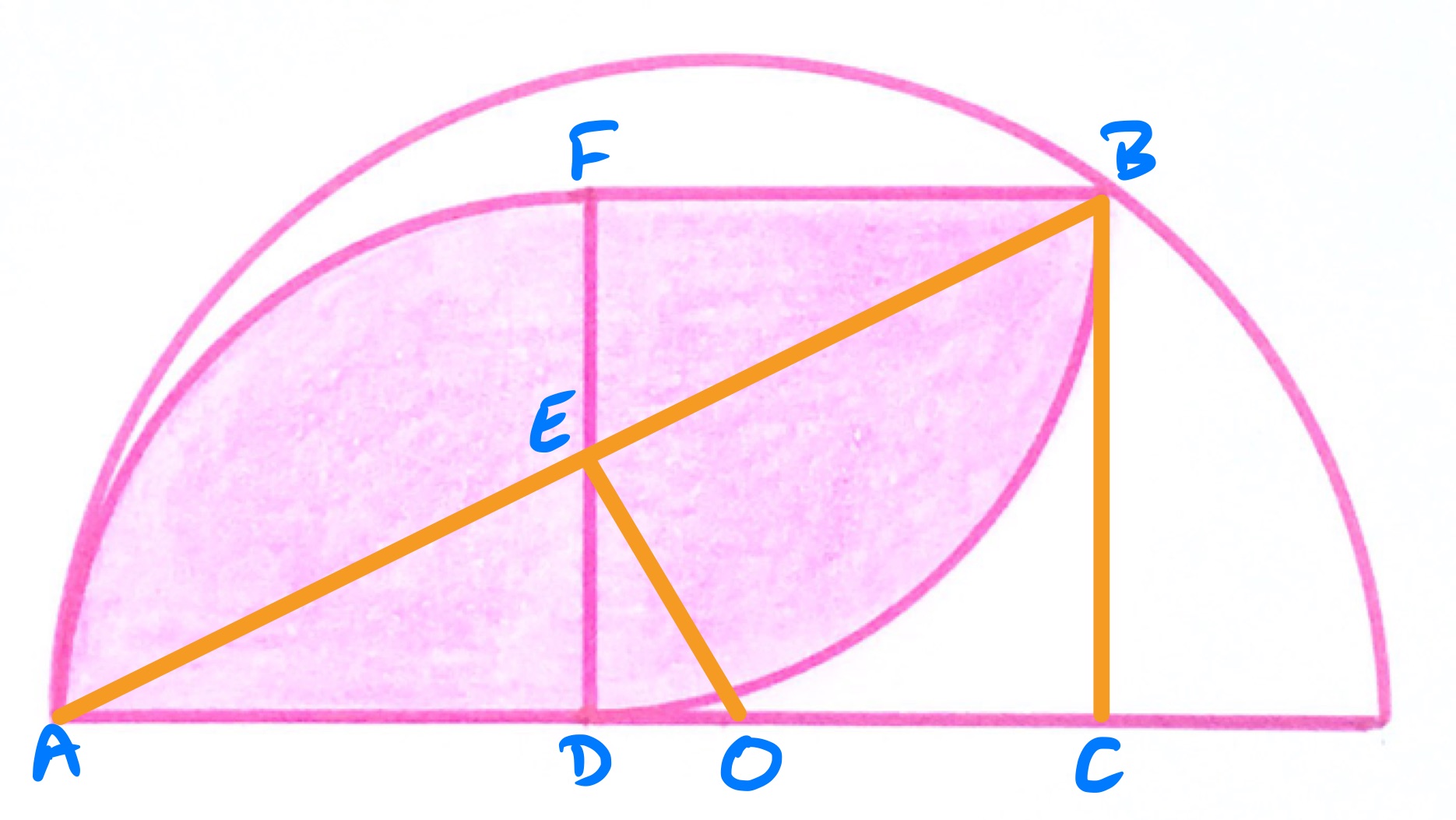
In the above diagram, the point $O$ is the centre of the circle, the point $E$ is where the chord ​$A B$ intersects the line up from $D$, and $C$ is so that $B C$ is perpendicular to the diameter.

As the quarter circles have the same radius, $D$ is the midpoint of $A C$ and so $E$ is the midpoint of $A B$. The line segment $O E$ is therefore perpendicular to the chord $A B$. This means that angles $A \hat{E} D$ and $D \hat{E} O$ add up to $90^\circ$, but also angles $A \hat{E} D$ and $D \hat{A} E$ add up to $90^\circ$ since angles in a triangle add up to $180^\circ$, so angle $D \hat{E} O$ is equal to angle $D\hat{A}E$. As angle $O \hat{D} E$ is a right-angle, this establishes triangle $E D O$ as similar to triangle $A \hat{D} O$.

As $E$ is the midpoint of $A B$, it is also the midpoint of $D F$ and so $D E$ has length half that of $A D$. By similarity, therefore, $D O$ has length half that of $D E$ and so a quarter of that of $A D$. The length of $A O$ is therefore $\frac{5}{4}$ of that of $A D$, equivalently the length of $A D$ is $\fract{4}{5}$ of $A O$.

The area of the quarter circles is $2 \times \frac{1}{4} \pi A D^2$ and of the semi-circle is $\frac{1}{2} \pi A O^2$. The ratio of these areas is therefore $\left(\frac{4}{5}\right)^2 = \frac{16}{25}$.