Notes
two overlapping squares solution
Solution to the Two Overlapping Squares Puzzle
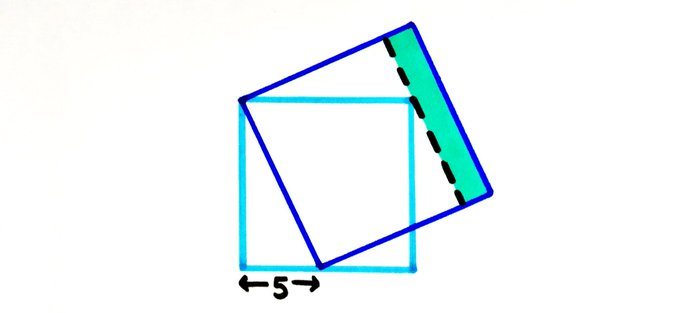
Two overlapping squares. What’s the shaded area?
Solution by Pythagoras' Theorem and Similar Triangles
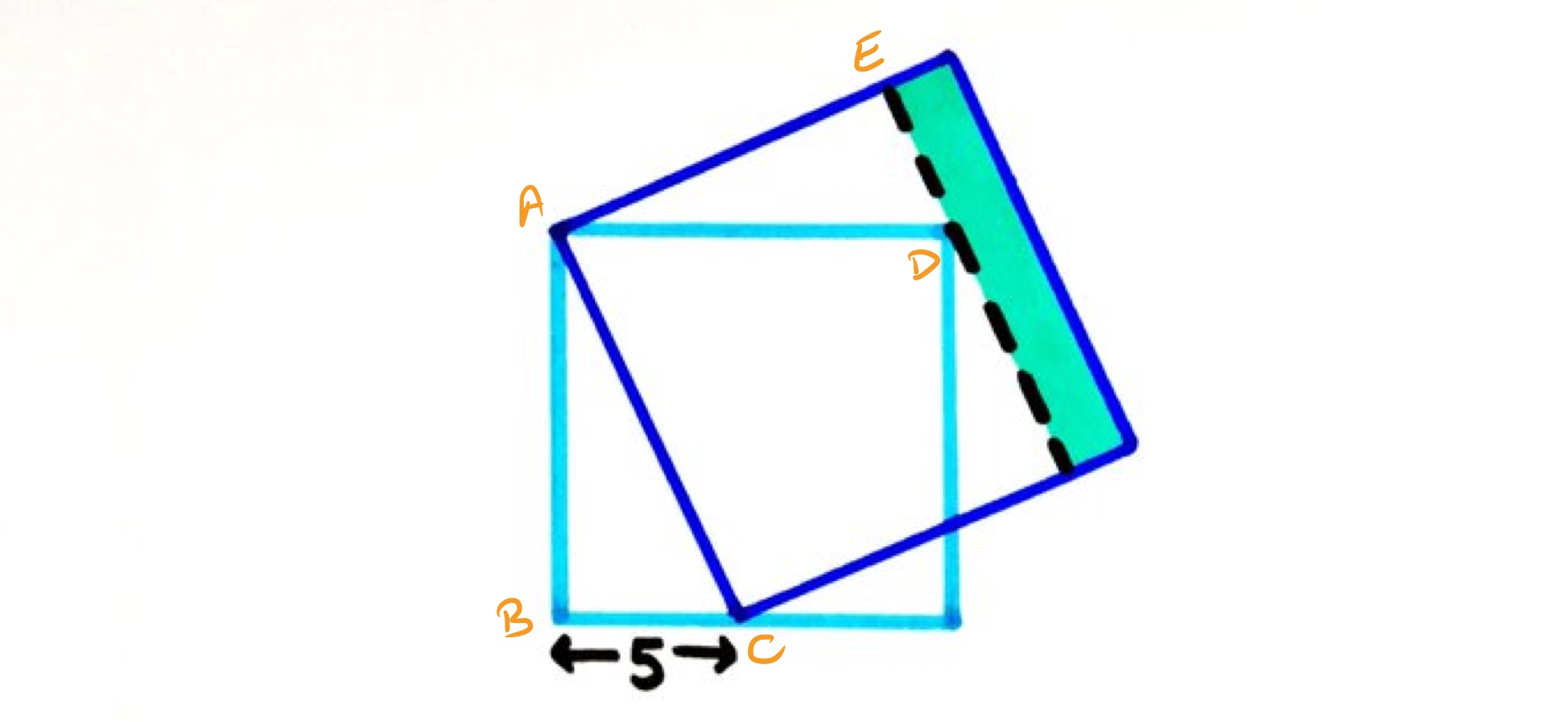
Let the two squares have side lengths and , with for the light blue and for the dark blue. Applying Pythagoras' theorem to triangle shows that:
Triangle is similar to triangle with scale factor , so the length of is . The area of the shaded region is then given by: