Notes
triangle inside circle and triangle solution

Solution to the Triangle Inside Circle and Triangle Puzzle

Solution by Angles in a Cyclic Quadrilateral, Angles at a Point on a Straight Line, and Similar Triangles

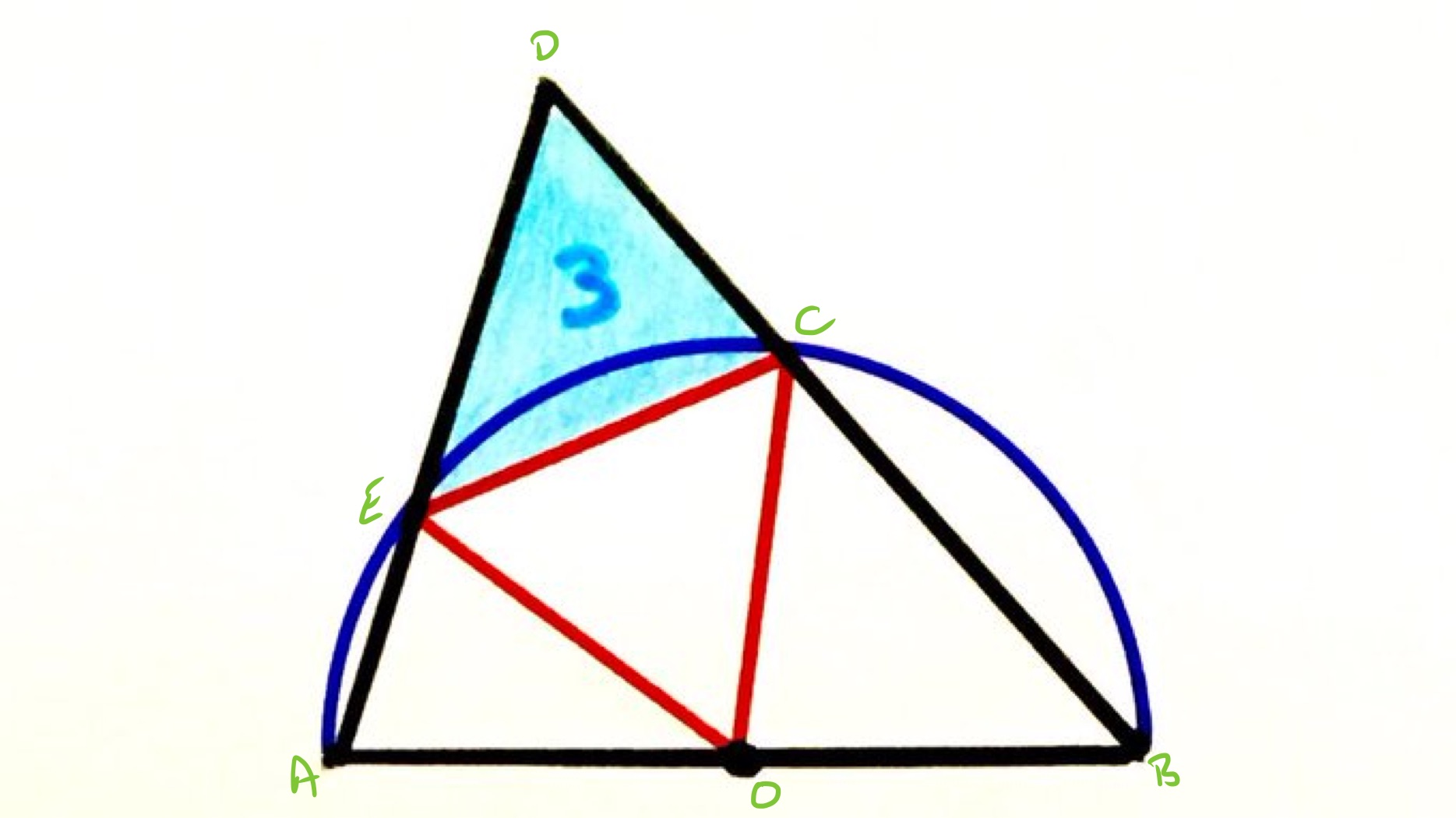
With the points labelled as above, angles $A \hat{E} C$ and $C \hat{B} A$ add up to $180^\circ$ as they are the opposite angles in a cyclic quadrilateral, so angle $C \hat{E} D$ is the same as angle $C \hat{B} A$ since angles at a point on a straight line also add up to $180^\circ$. Similarly, angles $D \hat{C} E$ and $B \hat{A} E$ are equal. So triangles $D E C$ and $D B A$ are similar, with $C$ corresponding to $A$ and $E$ to $B$. The scale factor comes from comparing $E C$ with $A B$: since $E C$ has length equal to a radius of the semi-circle and $A B$ is a diameter, the scale factor is $2$. Therefore triangle $D A B$ has are four times that of $D E C$, so has area $12$.