Notes
triangle in semi-circle with square solution
Solution to the Triangle in Semi-Circle with Square Puzzle
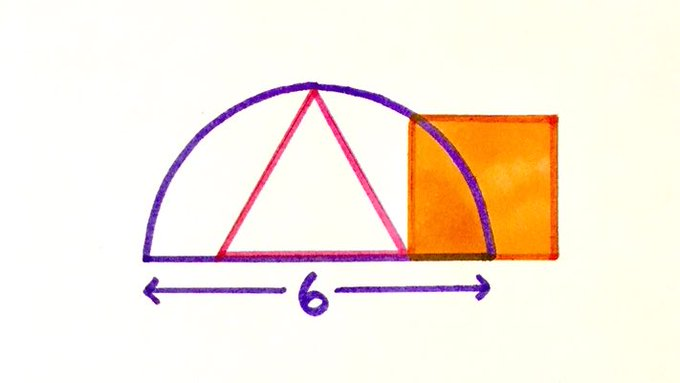
The equilateral triangle sits in the centre of the semicircle. What’s the area of the square?
Solution by Lengths in an Equilateral Triangle and Pythagoras' Theorem

With the points labelled as above, the radius of the semi-circle is and this is the height of the equilateral triangle. From lengths in an equilateral triangle, the length of is . Applying Pythagoras' theorem to triangle shows that the square of the side length of the square is and so the area of the square is .