Notes
three squares inside a triangle solution

Solution to the Three Squares Inside a Triangle Puzzle

Solution by Similar Triangles and Pythagoras' Theorem

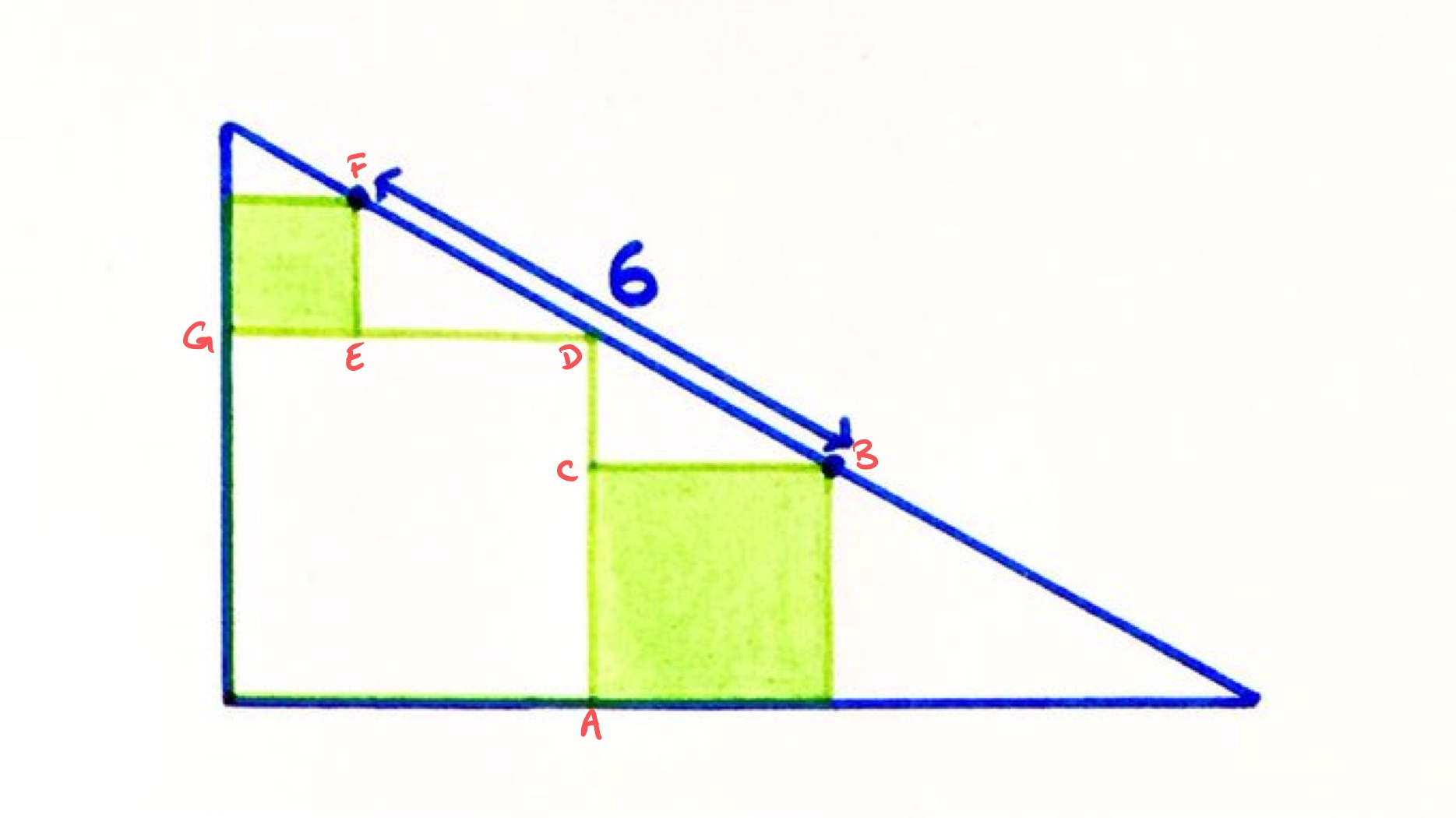
With the points labelled as in the above diagram, triangles $D E F$ and $B C D$ are similar. The lengths of $C B$ and $C A$ are the same, and the lengths of $E F$ and $G E$ are the same. So the full length of $D A$ is the sum of the lengths of $D C$ and $C B$, and the full length of $G D$ is the same as the sum of the lengths of $G E$ and $E F$. These are sides of the white square, so $G D$ and $D A$ are the same length, and so the triangles $D E F$ and $B C D$ are actually congruent.

This means that the length of $B D$ is half of that of $B F$, so is $3$. Also $E F$ and $D C$ are the same length. Writing $a$ for the length of $B C$ and $b$ for the length of $C D$, the sum of the two shaded areas is $a^2 + b^2$. Applying Pythagoras' theorem to triangle $B C D$ shows that $a^2 + b^2 = 3^2 = 9$ so the sum of the two shaded areas is $9$.