Notes
three squares in a triangle solution

Three Squares in a Triangle

Solution by Similar Triangles

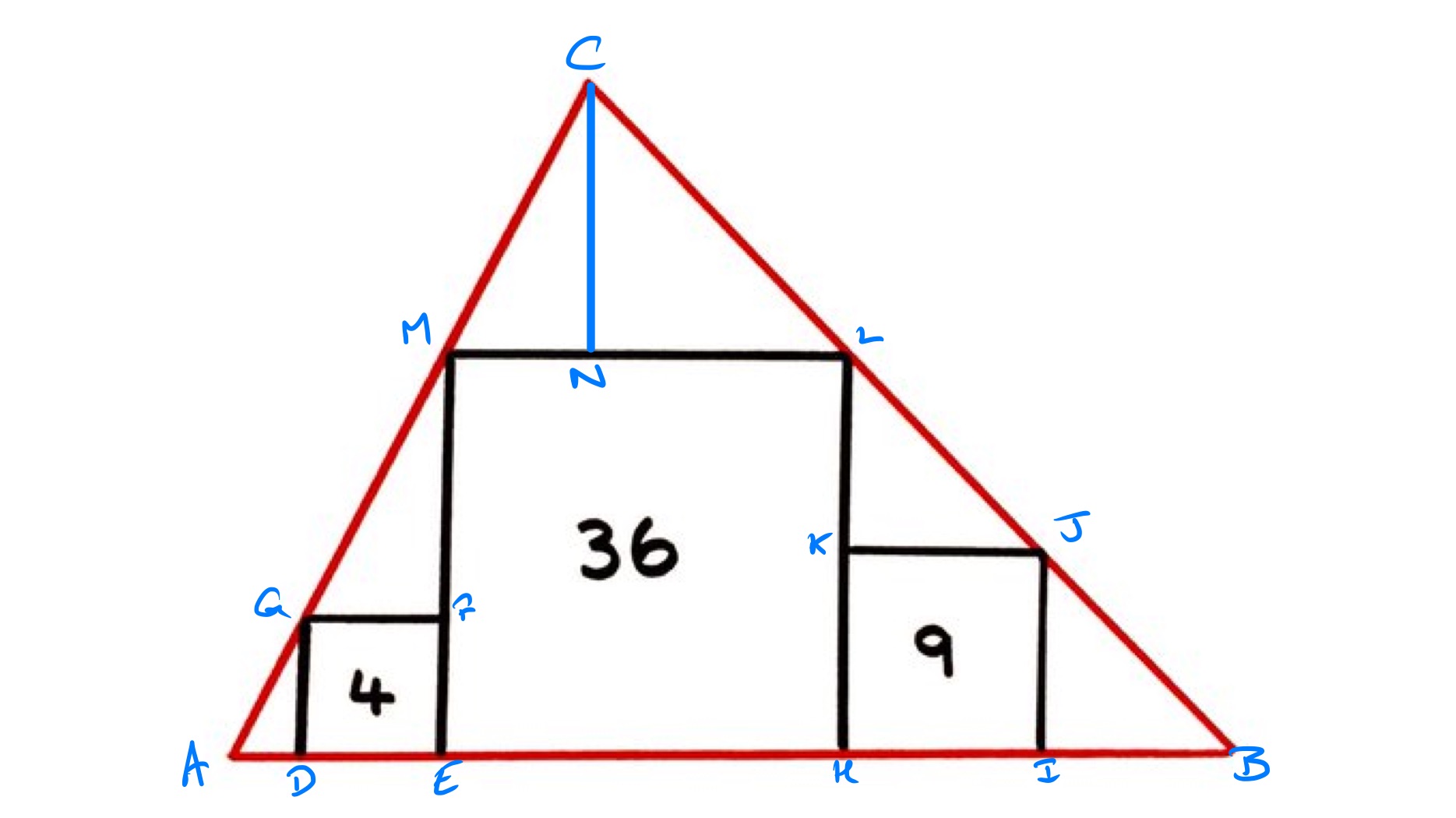
With the points labelled as above, the areas of the squares mean that the side lengths are as follows: the length of $D E$ is $2$, the length of $E H$ is $6$, and the length of $H I$ is $3$.

Then $L K$ has length $6 - 3 = 3$ which is the same length as $K J$ so triangle $L K J$ is an isosceles right-angled triangle. Triangles $J I B$ and $C N L$ are similar to this triangle, so $I B$ also has length $3$ and $N L$ is the same length as $C N$.

On the other side, $M F$ has length $6 - 2 = 4$ so in right-angled triangle $G F M$, the lengths of the horizontal to vertical sides are in the ratio $1:2$. This means that $A D$ has length $1$ and the length of $M N$ is half that of $C N$.

Putting these together, the length of $M L$ is $\frac{3}{2}$ times the length of $C N$, so the length of $C N$ is $4$ as that of $M L$ is $6$.

The length of the base of the triangle, so of $A B$, is then $1 + 2 + 6 + 3 + 3 = 15$ and its height is $6 + 4 = 10$. Therefore its area is $75$.