Notes
three squares iii solution
Solution to the Three Squares III Puzzle
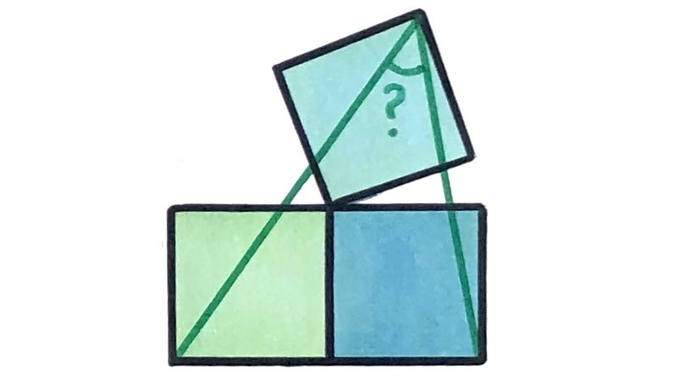
All three squares are the same size. What’s the angle?
Solution by Angle at the Circumference is Half the Angle at the Centre
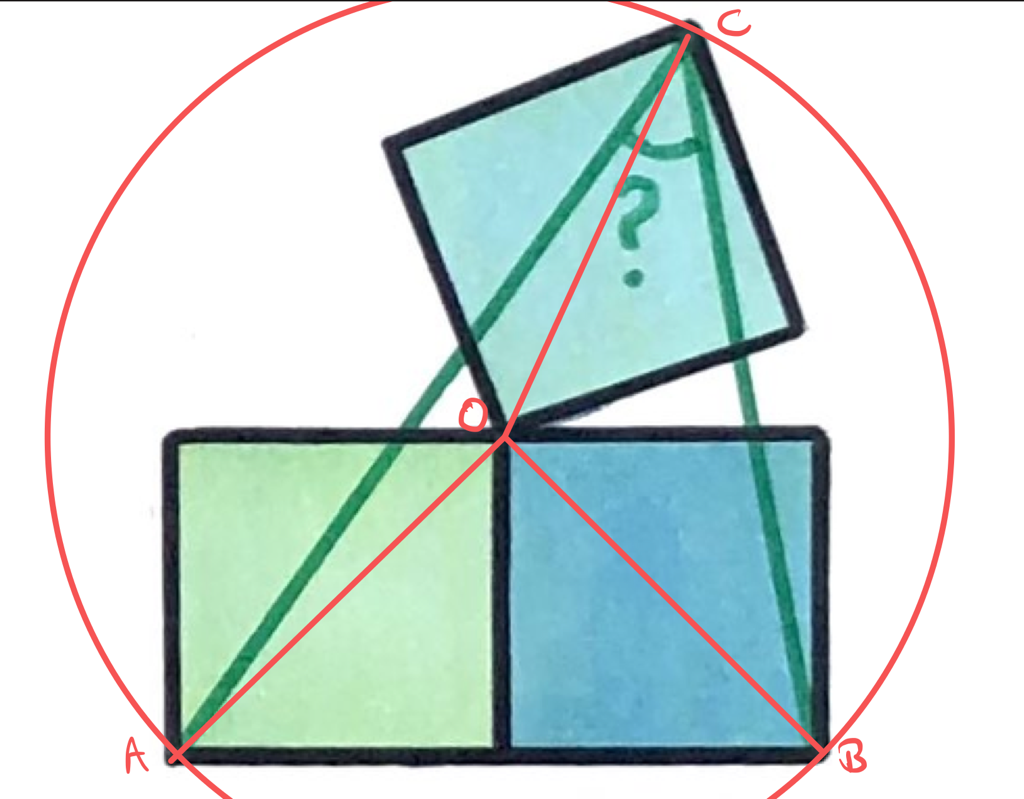
With the points labelled as above, the line segments , , and are all diagonals of congruent squares, so are the same length. Therefore the circle centre that passes through also passes through and .
Then since the angle at the circumference is half the angle at the centre, angle is half angle . But this is as it is formed from the diagonals of two adjacent squares.
Hence angle .
Solution by Isosceles Triangles and Angles in a Triangle
With the same labelling as above, triangles , , and are isosceles. Since and are the diagonals of squares, angles and are both . Since the angles in triangle add up to , this means that the sum of angles , , , and is .
Then since triangles and are isosceles, angles and are equal to each other, as are angles and . Therefore, angle is half of so is .
Solution by Agg Invariance Principle and Angles in a Square
The upper square is tilted, but the angle of the tilt is not specified. A special case is where it aligns with the right-hand lower square, as in the diagram below.
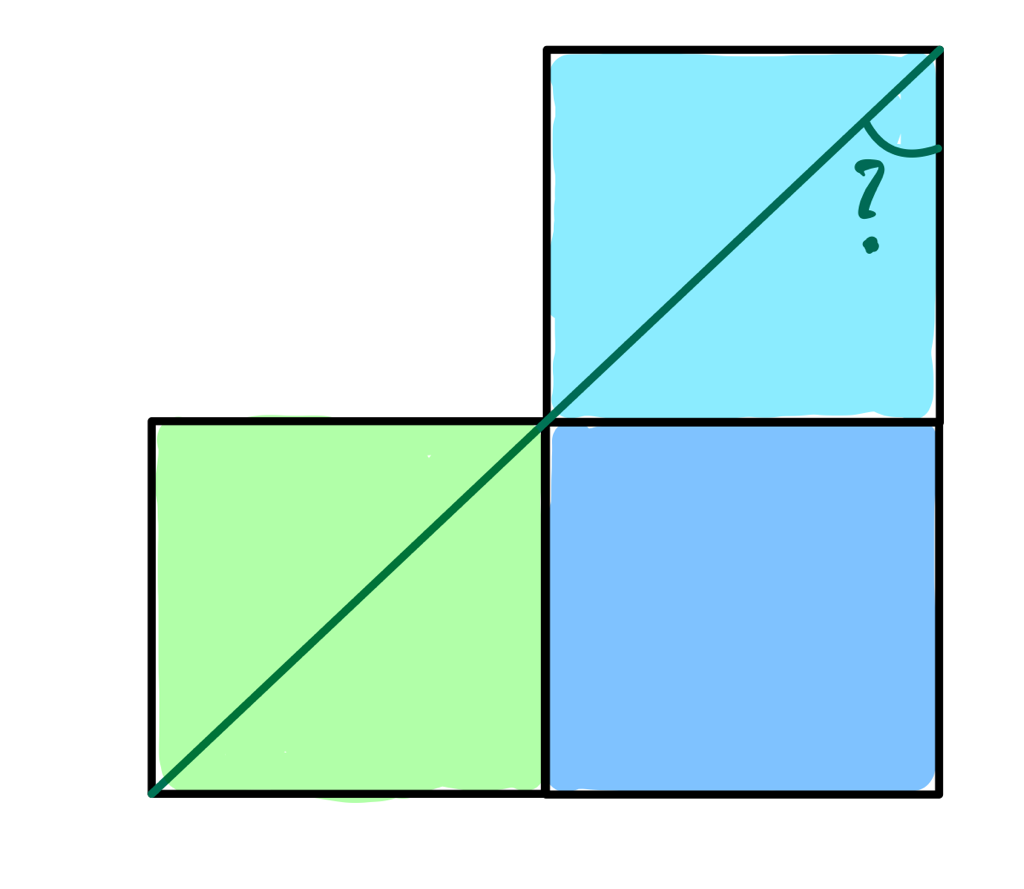
In this case, the requested angle is the angle between a diagonal and side in a square, hence is .