Notes
three squares and a semi-circle solution

Solution to the Three Squares and a Semi-Circle Puzzle

Solution by Properties of Chords and Pythagoras' Theorem

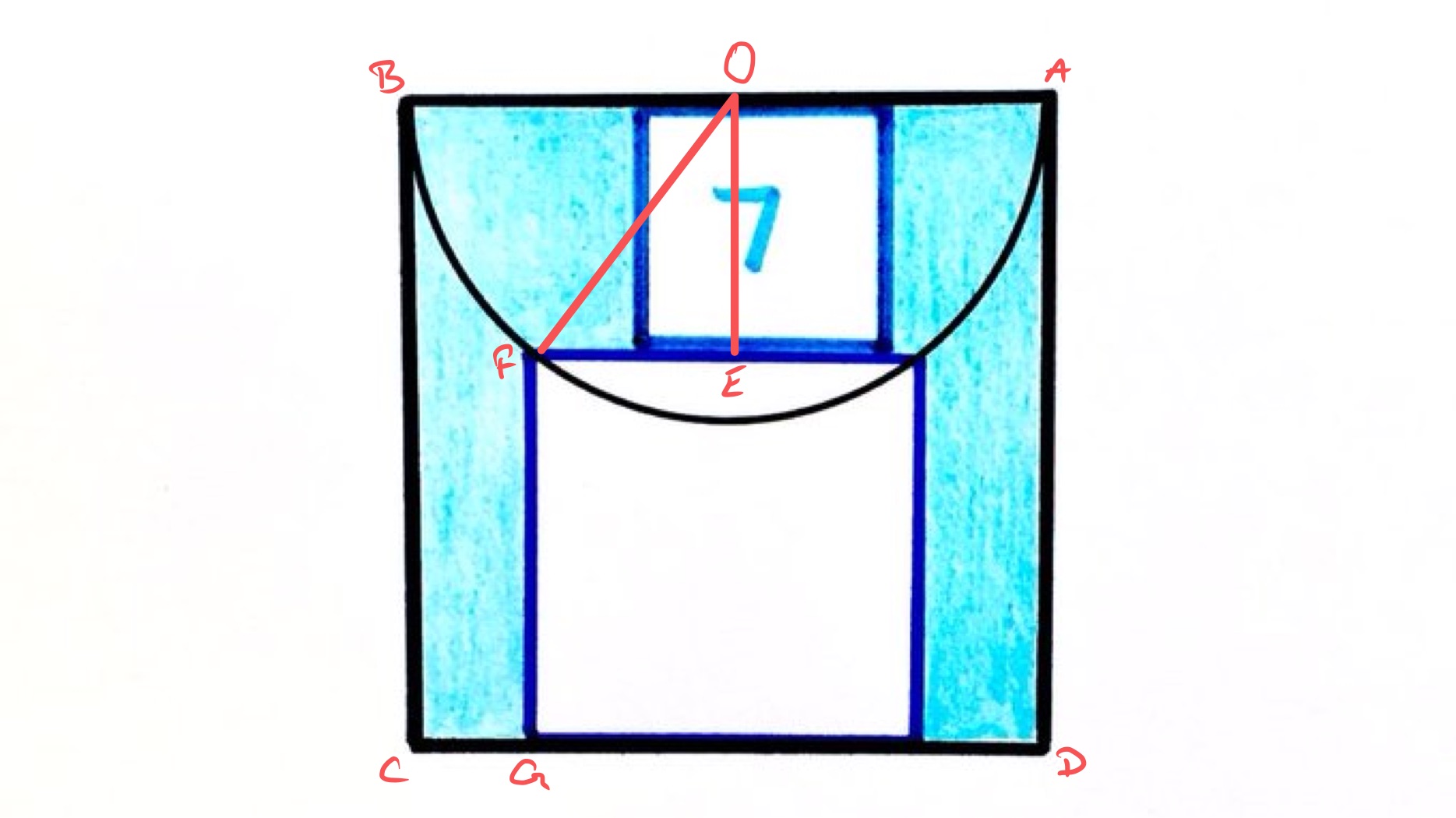
In the diagram above, the point labelled $O$ is the centre of the semi-circle.

Let the squares have side lengths $a$, $b$, and $c$ in increasing order, so that $a^2 = 7$. The area of the shaded region is $c^2 - b^2 - a^2 = c^2 - b^2 - 7$.

As one of the sides of the middle square forms a chord across the circle, $F E$ is half of that side and is perpendicular to $O E$. Therefore triangle $O F E$ is right-angled and has side lengths $a$, $\frac{b}{2}$, and $\frac{c}{2}$. Applying Pythagoras' theorem shows that:

Then the shaded region has area $c^2 - b^2 - 7 = 21$.