Notes
swirling semi-circles solution

Solution to the Swirling Semi-Circles Puzzle

Solution by Circle Area and Pythagoras' Theorem

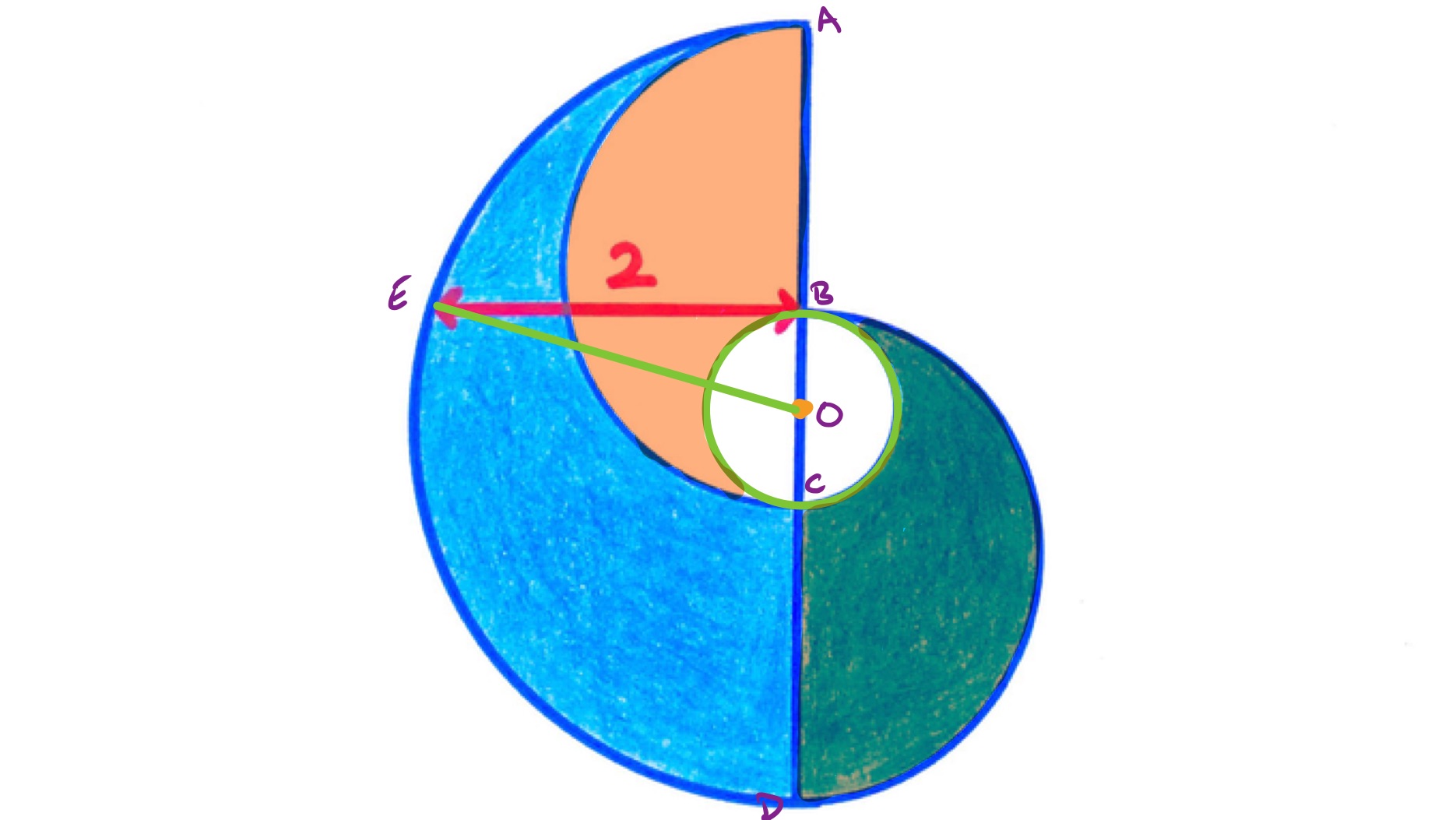
In the above diagram, $O$ is the centre of the smallest circle. As the largest and smallest semi-circles are concentric, the lengths of $O A$ and $O D$ are equal, so the lengths of $C A$ and $B D$ are also equal. These are the diameters of the two middle size semi-circles, so they are the same size. This means that the orange and green areas in the above diagram are congruent.

The shaded area is therefore the same as the difference between the areas of the largest and smallest semi-circles. Let $a$ be the radius of the smallest semi-circle and $b$ of the largest. These two radii form two sides of the right-angled triangle $O B E$. So by Pythagoras' theorem,

The area of the shaded region is therefore given by $\frac{1}{2} \pi b^2 - \frac{1}{2} \pi a^2 = \frac{1}{2} \pi \times 4 = 2 \pi$.