Notes
subdivided parallelogram solution

Solution to the Subdivided Parallelogram Puzzle

Solution by Similar Triangles and Angles in Parallel Lines

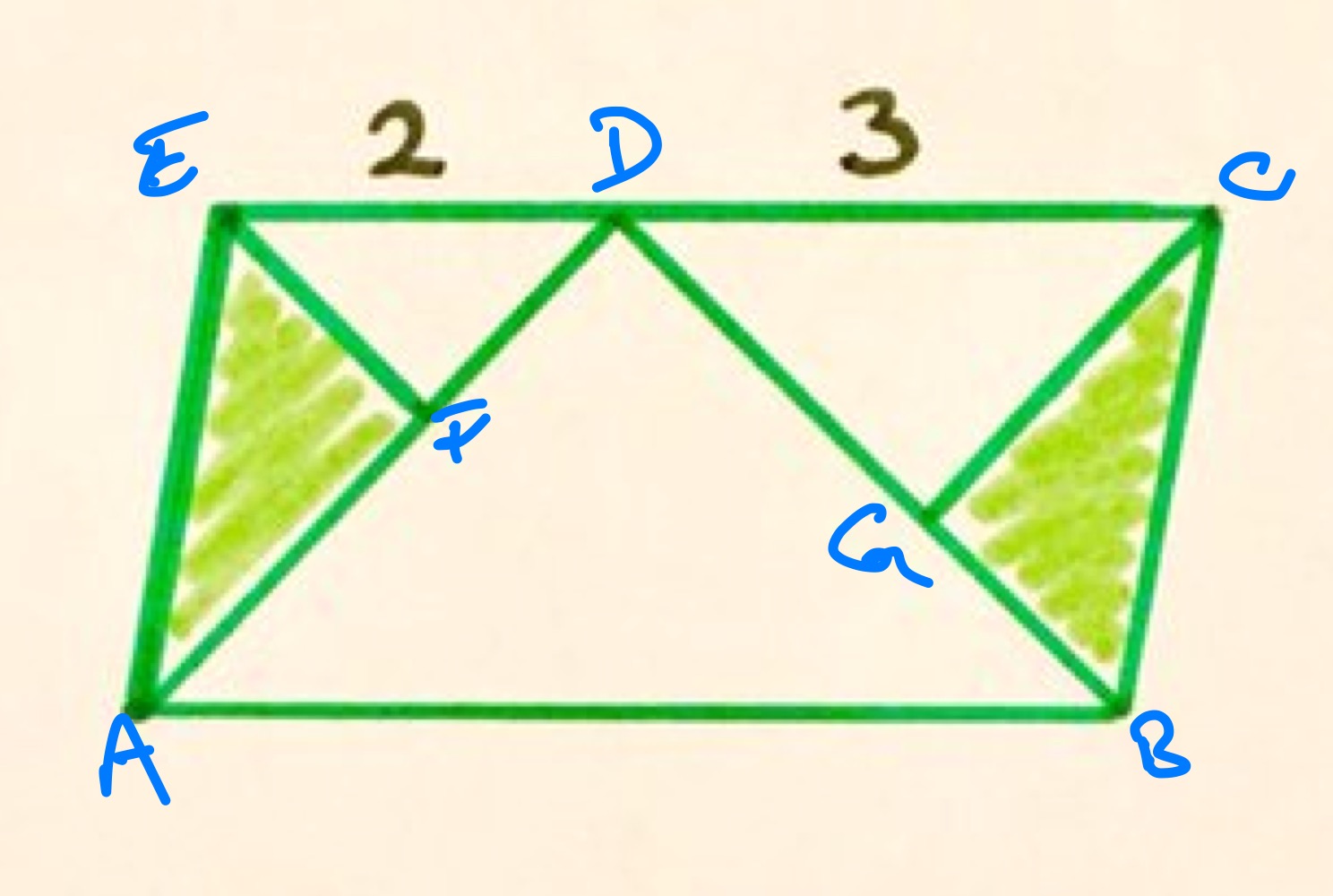
Note: there is a hidden assumption here that the bases of the unshaded triangles lie along the edges of the outer parallelogram.

Consider the diagram as labelled above. As the outer shape is a parallelogram, line segments $E C$ and $A B$ are parallel. Therefore by angles in parallel lines, angles $D \hat{B} A$ and $B \hat{D} C$ are equal, as are angles $D \hat{A} B$ and $A \hat{D} E$.

The base angles of the isosceles triangles $A D B$, $E F D$, and $D G C$ are therefore all the same and so these triangles are all similar. The sides $E D$, $D C$, and $A B$ correspond, so the scale factors from the larger triangle to each of the smaller are, respectively, $\frac{2}{5}$ and $\frac{3}{5}$. The corresponding area scale factors are $\frac{4}{25}$ and $\frac{9}{25}$.

The area of triangle $A D B$ is half that of the parallelogram so the unshaded area is

of the parallelogram. The shaded area is therefore $\frac{6}{25}$ths of the parallelogram.