Notes
stack of congruent rectangles solution

Solution to the Stack of Congruent Rectangles Puzzle

Solution by Similar Triangles and Pythagoras' Theorem

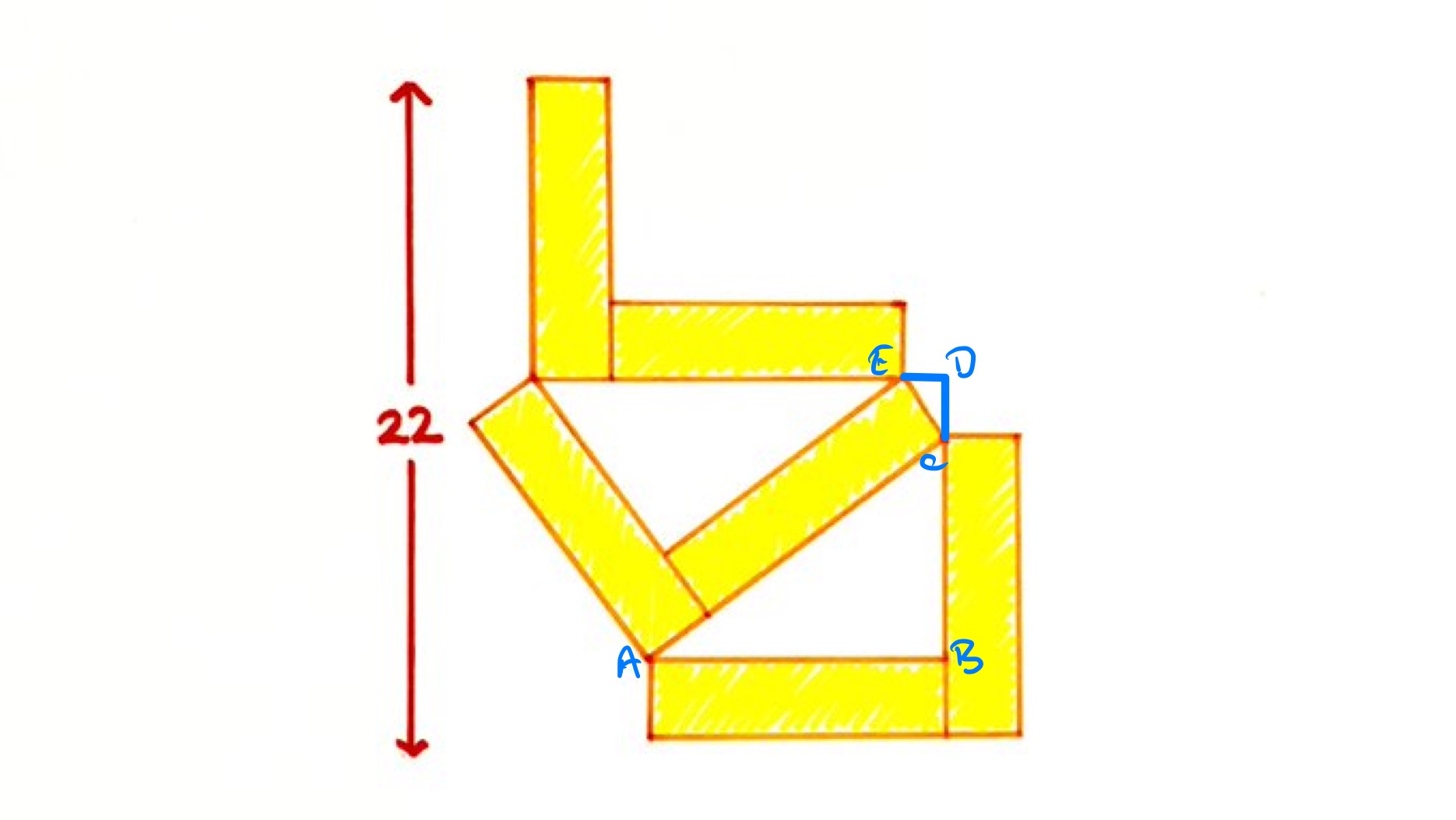
In the diagram above, $D$ is the point so that $E D$ is parallel to $A B$ and $C D$ is the continuation of $B C$.

Let $a$ and $b$ be the side lengths of the rectangles, so that $A B$ has length $a$ and $E C$ has length $b$. Then $B C$ has length $a - b$ and $A C$ has length $a + b$. Triangles $A B C$ and $C D E$ are similar since they are both right-angled triangles and angles $A \hat{C} B$ and $D \hat{C} E$ add up to $90^\circ$. By comparing $E C$ with $A C$, the scale factor is $\frac{b}{a + b}$ so the length of $C D$ is $\frac{a b}{a + b}$. This means that the total height of the figure is $2 a + \frac{a b}{a + b} = \frac{2 a^2 + 3 a b}{a + b}$.

Applying Pythagoras' theorem to triangle $A B C$ shows that:

which simplifies to $4 a b = a^2$ so $a = 4 b$. Substituting in to the expression for the height, and setting that equal to $22$, gives:

So $b = \frac{5}{2}$ and $a = 10$. The total area of the rectangles is therefore: