Notes
square on an octagon solution

Solution to the Square on an Octagon Puzzle

Solution by Symmetry and Isosceles Right-Angled Triangle

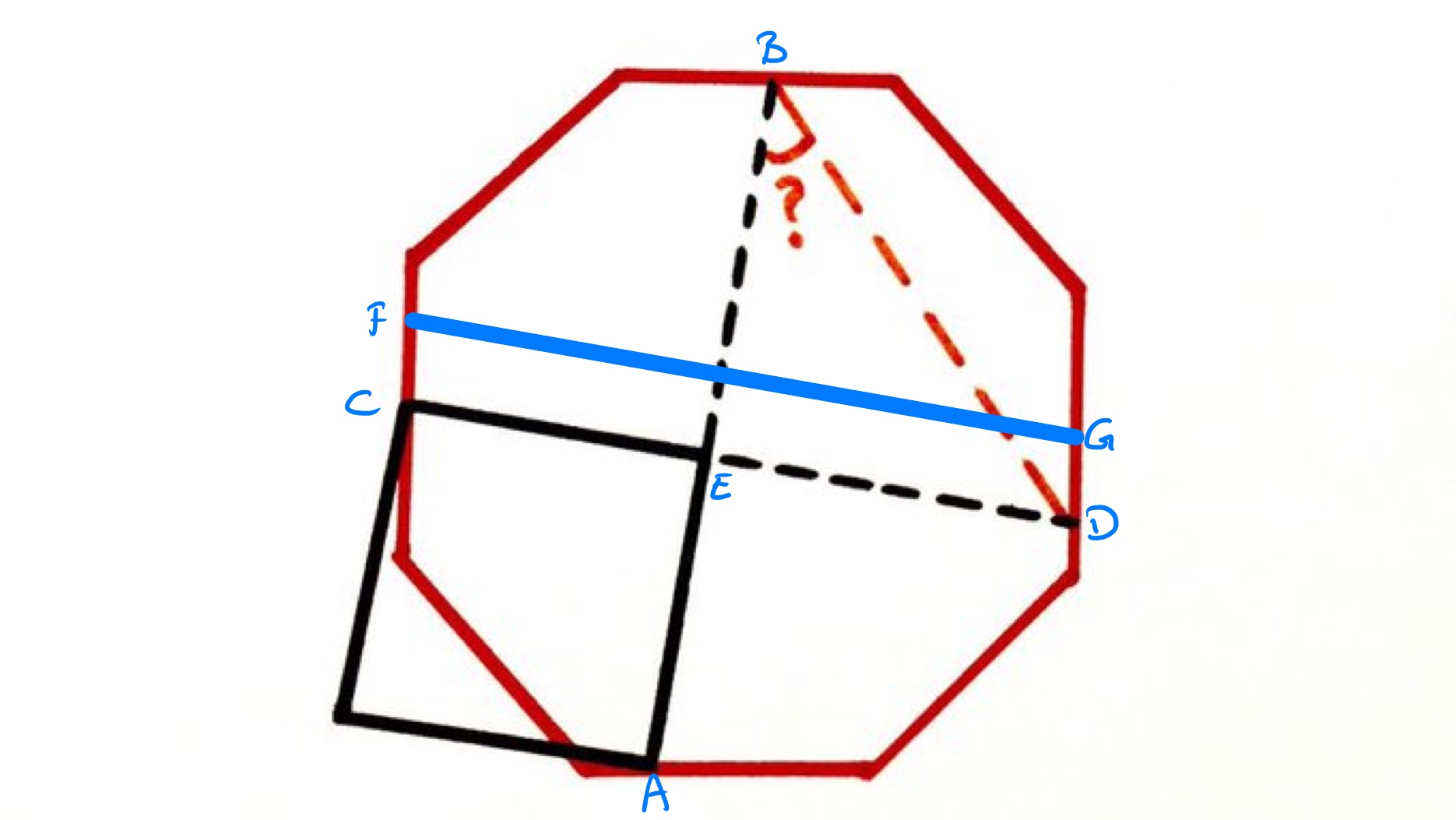
In the above diagram, the line segment $F G$ is the rotation of $A B$ about the centre of the octagon. It therefore has the same length as $A B$. The line segment $C D$ is parallel to $F G$ since it also intersects $A B$ at right-angles. As both $F G$ and $C D$ end on the same sides of the octagon, they are the same length. So $C D$ is the same length as $A B$. Since $E A$ and $E C$ are sides of the square, they have the same length and hence $E B$ and $E D$ have the same length. Therefore $D B E$ is an isosceles right-angled triangle and so angle $E \hat{B} D$ is $45^\circ$.