Notes
square and rectangle overlapping in a semi-circle solution

Square and Rectangle Overlapping in a Semi-Circle

Solution by Area of a Triangle and Congruent Triangle

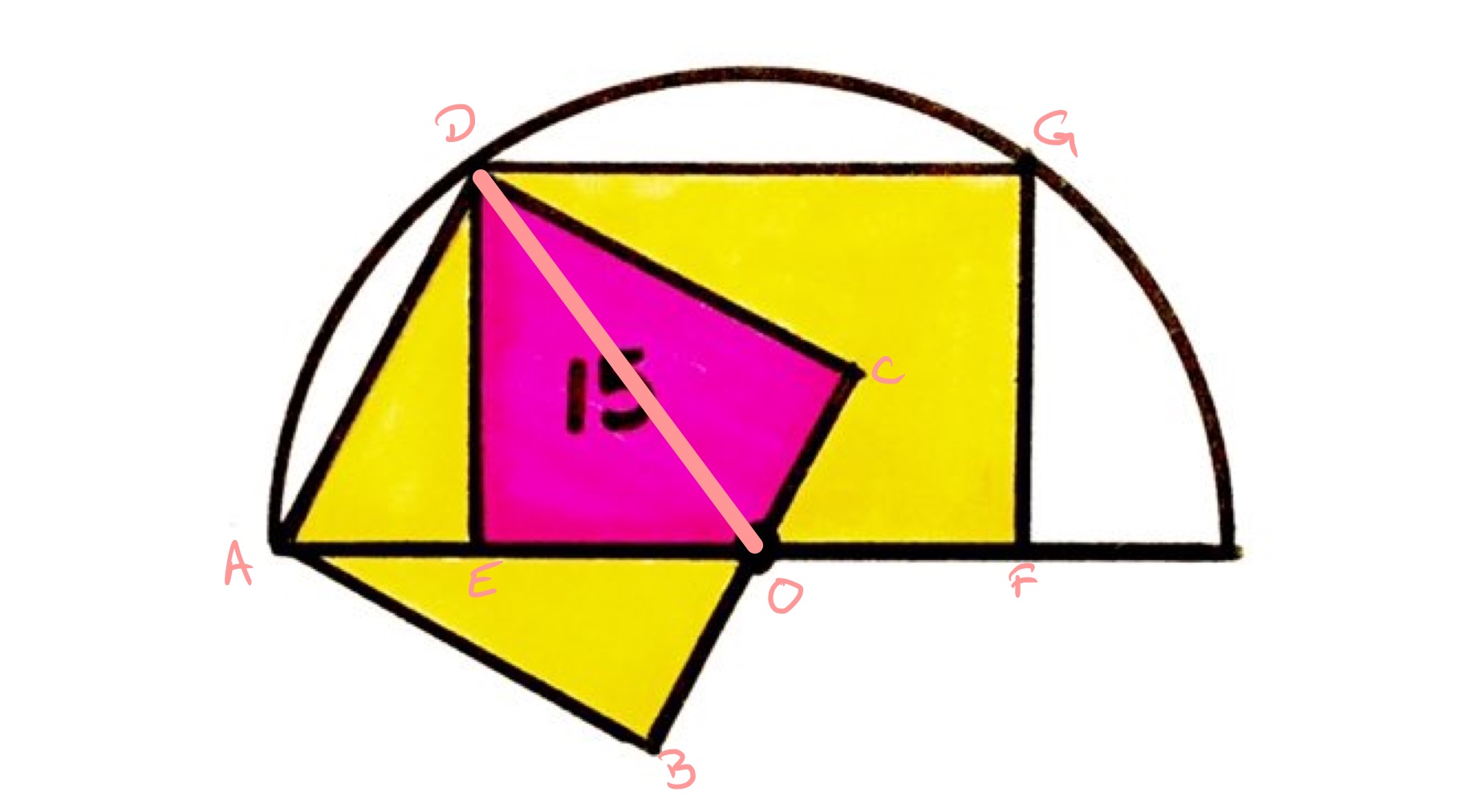
With the points labelled as above, both $O D$ and $O A$ are radii of the semi-circle and so have the same length. Triangle $A O D$ is therefore isosceles and so triangles $D C O$ and $A B O$ are congruent. This means that $O C$ is half the length of $B C$ and so the area of triangle $D C O$ is a quarter of that of the whole square.

A similar argument applies to rectangle $D E F G$ to show that the area of triangle $D E O$ is a quarter of that of the whole rectangle.

The two rectangles taken separately (that is, without considering their overlap) is therefore $4 \times 15 = 60$. To find the area of the yellow regions, each then needs the purple region removed so the area of the yellow region is $60 - 2 \times 15 = 30$.