Notes
similar rectangles solution
Solution to the Similar Rectangles Puzzle
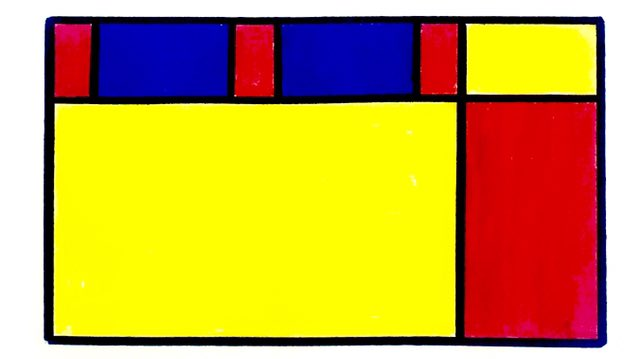
All the coloured rectangles are similar. What fraction of the design is red?
Solution by Similarity
Let the short side of the small red rectangle be and the long side . Then the blue rectangles and the smaller yellow rectangle have short side and so long side . The larger red rectangle therefore has short side and so long side . This is the short side of the larger yellow rectangle so its long side is . This is the same as lots of the short side of the small rectangle and lots of the long side of the blue, which is . Thus:
This rearranges to and this factorises as . Since is a length, and so positive, it must be .
The total area is . The area of the red rectangles is . The red area therefore comprises of the design.