Notes
seven quarter circles inside a semi-circle solution

Solution to the seven quarter circles inside a semi-circle problem

Solution using chords and similar triangles

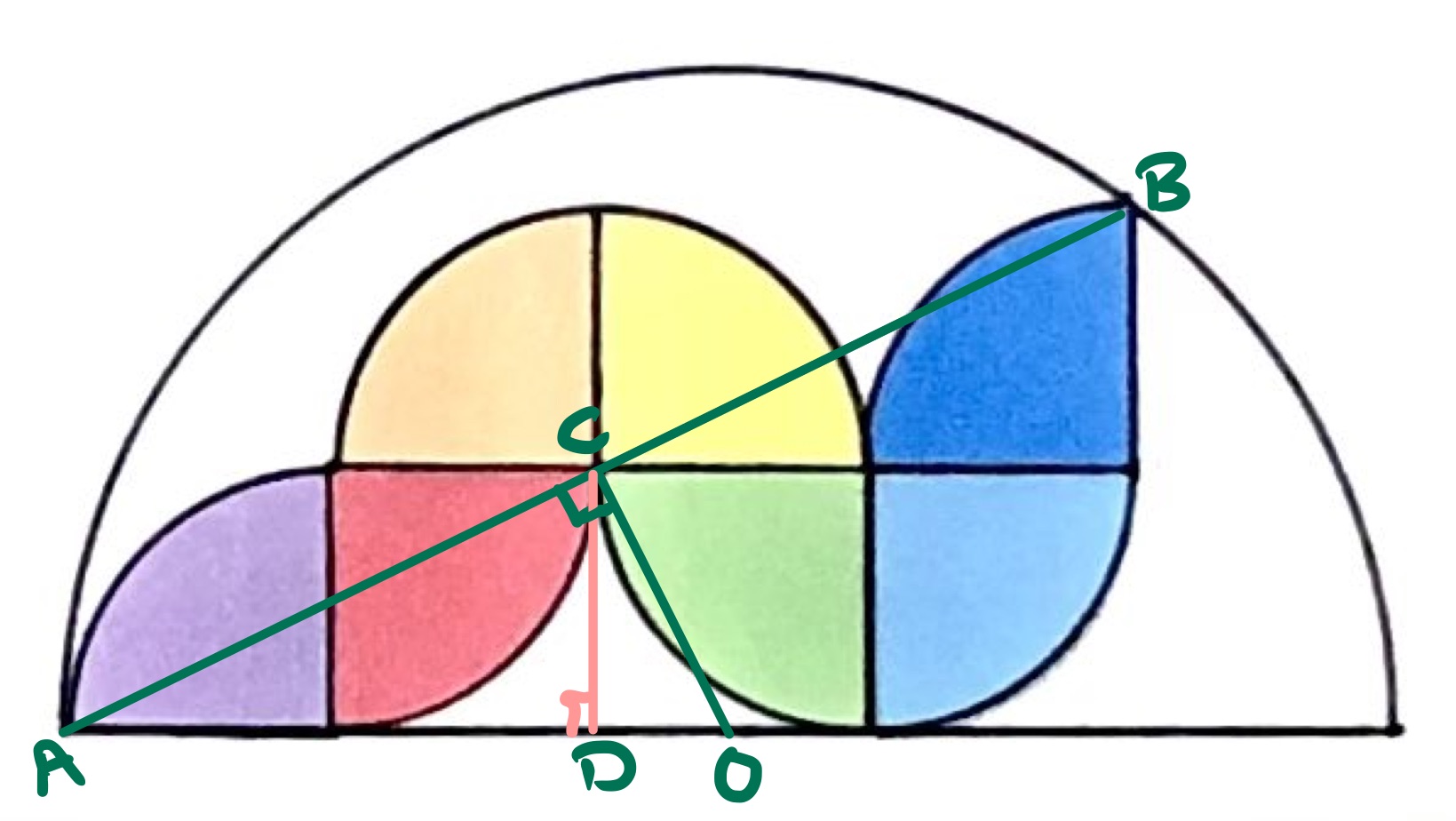
Consider the chord $A B$ across the diagram. The centre of the circle is located on its perpendicular bisector, which passes through the point $C$. As the base of the semi-circle is a diameter, the centre is therefore at the point where the perpendicular bisector of $A B$ meets this diameter, marked $O$ on the diagram.

Let $D$ be the point on the diameter below $C$, so that angle $C \hat{D} A$ is a right-angle. Then triangles $A D C$ and $C D O$ are similar. As the length $A D$ is twice that of $D C$, so also the length $C D$ is twice that of $D O$. Comparing these to the length of a radius of one of the quarter circles, $A O$ is therefore $2.5$ times this distance.

The semi-circle is therefore $2 \times 2.5^2 = 12.5$ times bigger than one of the quarter circles. There are seven of the quarter circles, so the area of the semi-circle is $\frac{12.5}{7} = \frac{25}{14}$ times bigger than the area of the combined quarter circles. Equivalently, the quarter circles cover an area $\frac{14}{25}$ of the area of the semi-circle.