Notes
region of an octagon solution
Region of an Octagon
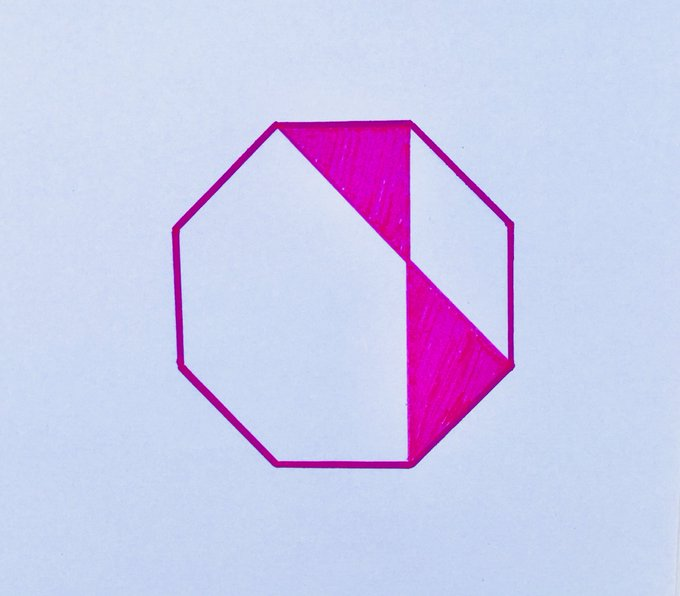
The shaded area has the same value as the perimeter of the regular octagon. What is this value?
Solution by Isosceles Right-angled Triangles
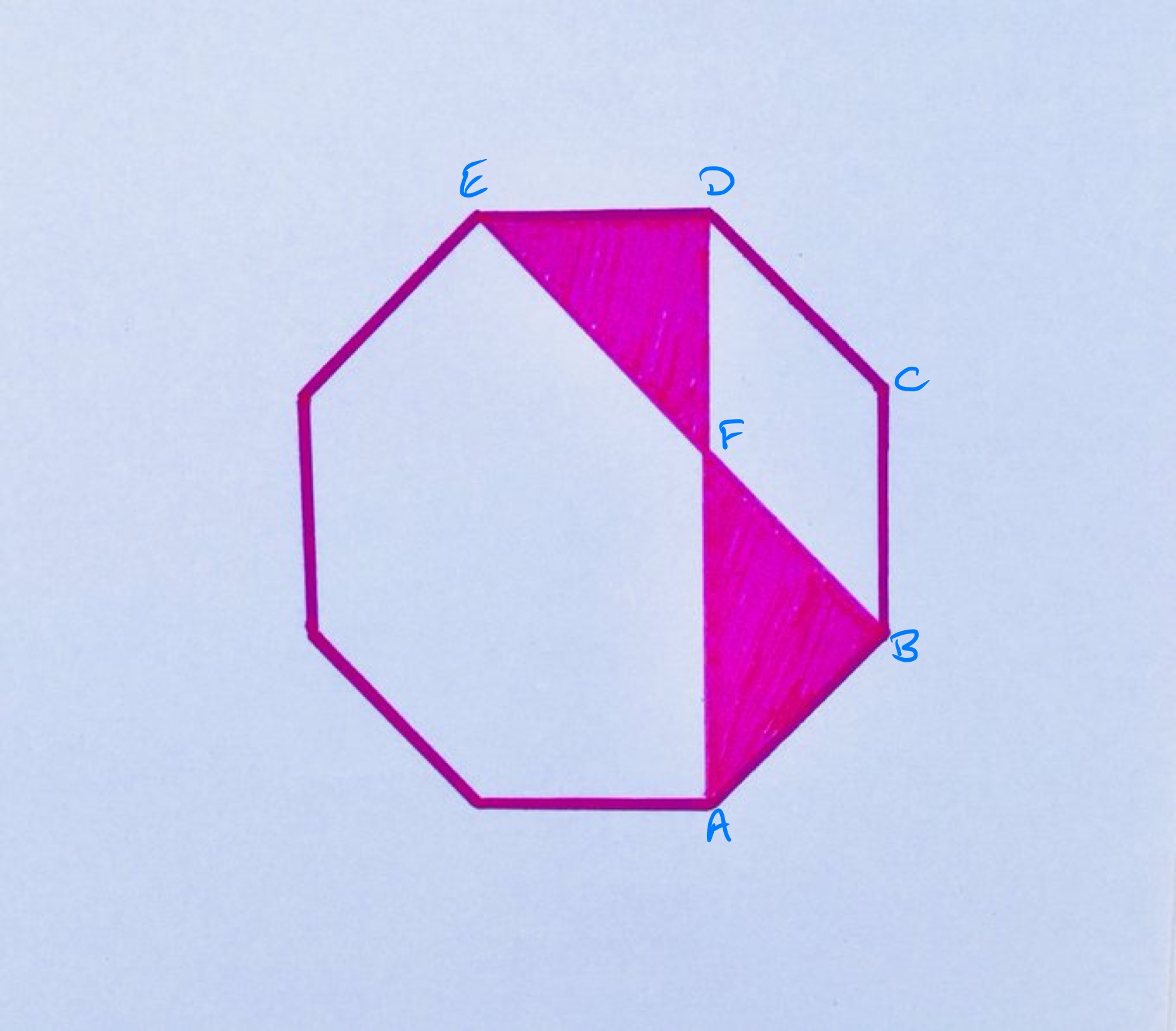
With the points labelled as above, is perpendicular to , and is at to , so triangle is an isosceles right-angled triangle. Let have length , then triangle has area . The same applies to triangle , so the shaded region has area . The perimeter of the octagon is , so and hence , so the common value for the area of the shaded region and the perimeter of the octagon is .