Notes
rectangle quartered by semi-circles solution

Solution to the Rectangle Quartered by Semi-Circles Puzzle

Solution by Alternate Angles and Similar Triangles

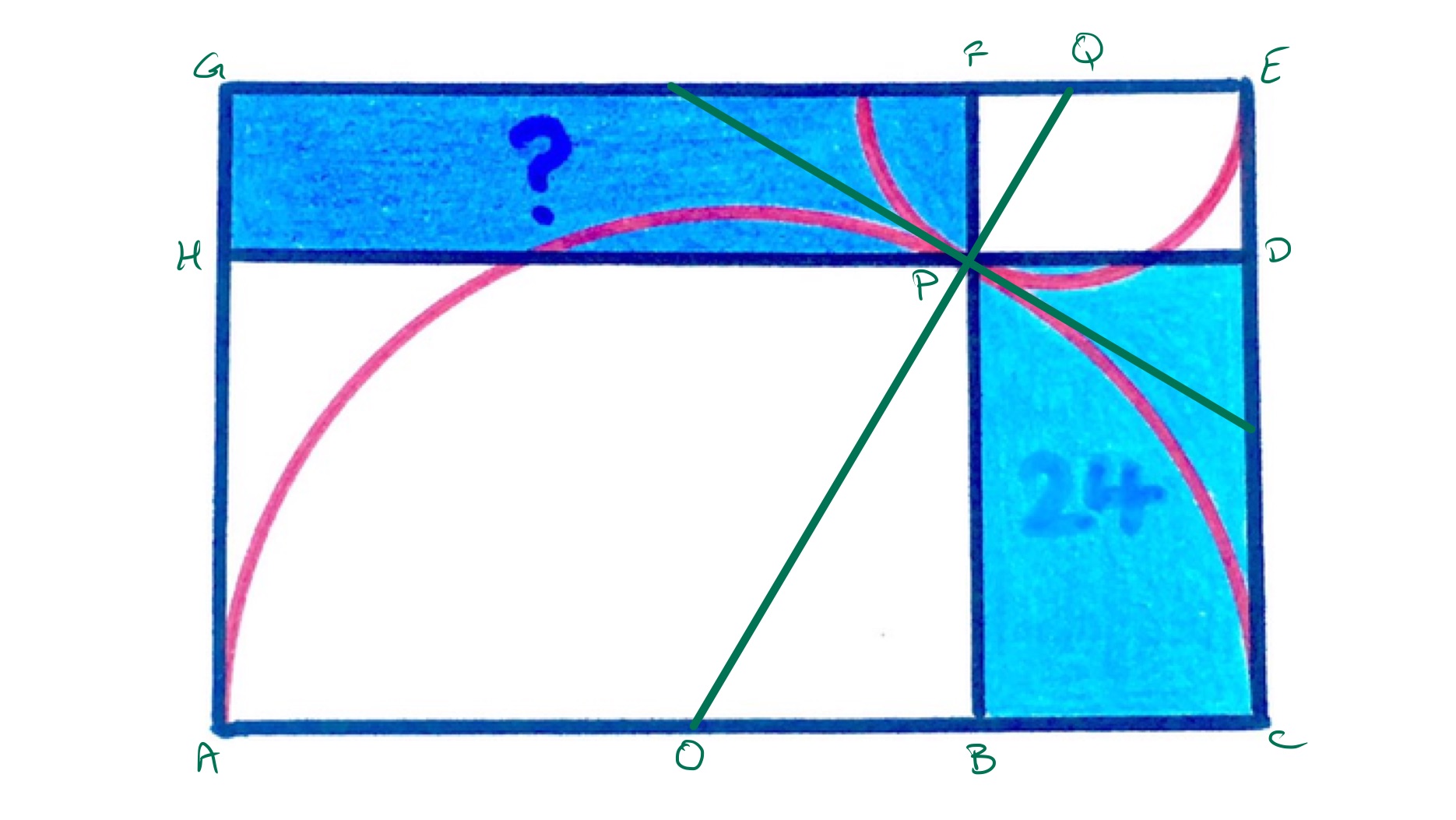
In the above diagram, the points labelled $O$ and $Q$ are the centres of their respective semi-circles. The line $O Q$ passes through the point $P$ where the circles touch. Angles $P \hat{O} B$ and $F \hat{Q} P$ are alternate angles so are equal and thus triangles $O B P$ and $Q F P$ are similar triangles. Since $O A$ and $O P$ are radii of the semi-circle, they have the same length; similarly, $P Q$ and $Q E$ have the same length. Therefore, the lengths of $A B$ and $E F$ are in the same ratio as those of $P B$ and $P F$. Replacing $A B$ by $H P$ and $E F$ by $P D$ in that leads to:

which rearranges to $H P \times P F = B P \times P D$ and so the blue rectangles have the same area, which is $24$.