Notes
quarter circles in a rectangle solution
Quarter Circles in a Rectangle
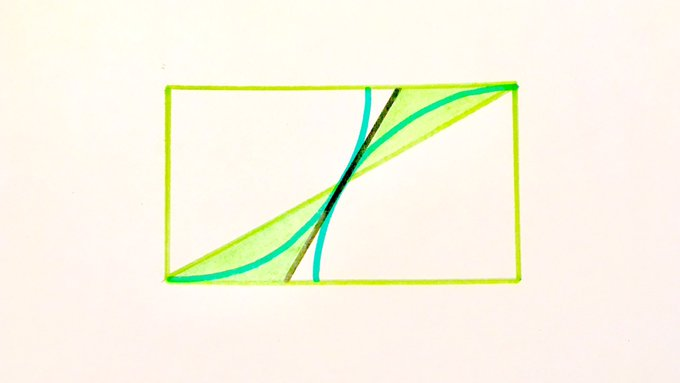
The black line is tangent to both quarter circles. What fraction of the rectangle is shaded?
Solution by Equilateral Triangles and Angle Between a Radius and Tangent
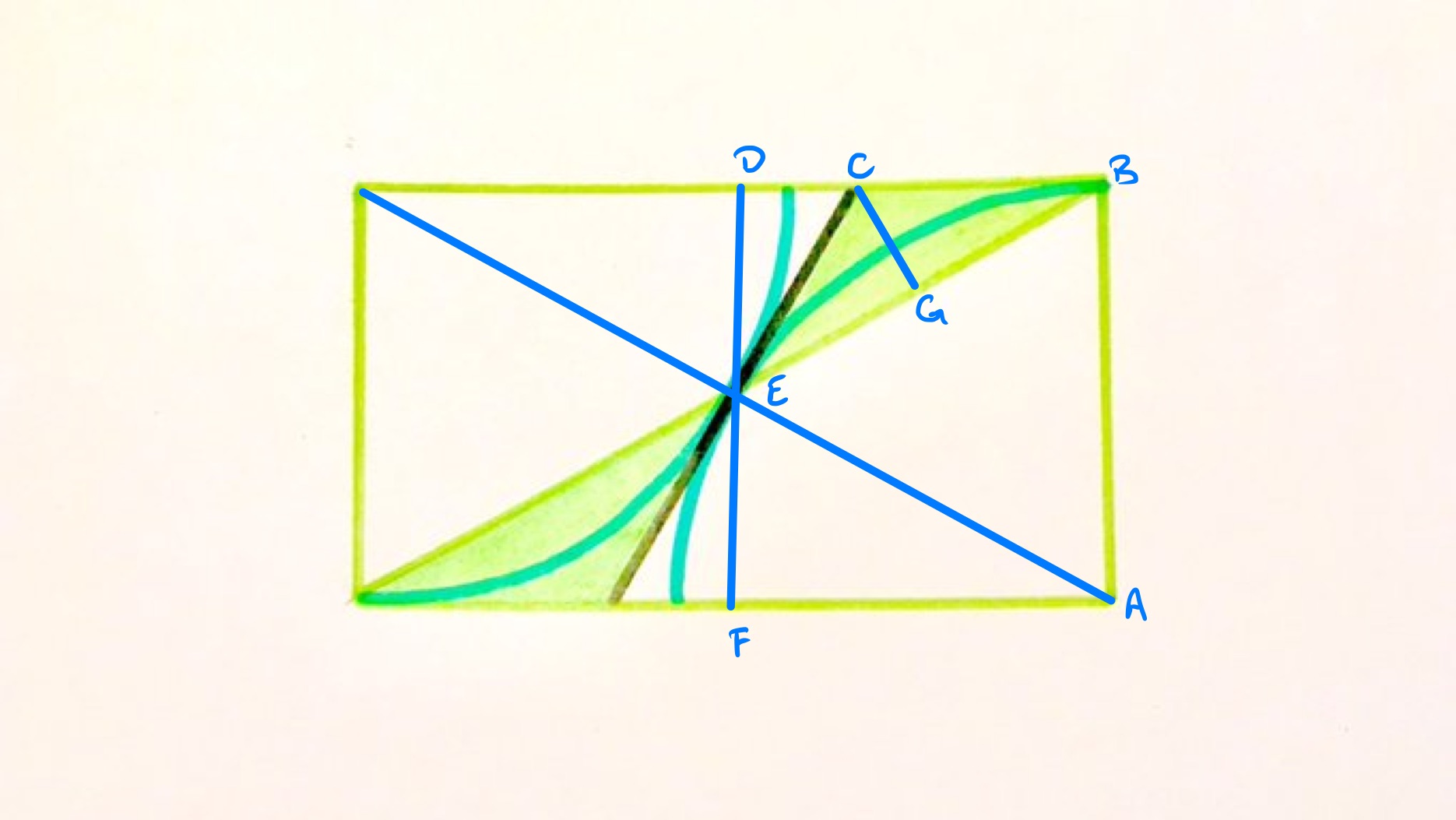
With the point labelled as above, line segments and are both half-diagonals so have the same length. Since and lie on a circle centred at , and have the same length. Therefore, triangle is equilateral. Triangle is then half an equilateral triangle of the same size, and the area of the whole rectangle is the same as four of these equilateral triangles.
Since the angle between a radius and tangent is , angle is and so triangle is isosceles. Point is the midpoint of , and so triangles and are congruent. Since is also a right-angled triangle with angle at vertex , it is also congruent to triangle , so the area of triangle is two thirds of that of .
Putting all of that together, the area of the shaded region is two thirds of that of the equilateral triangle and so is one sixth of the area of the full rectangle.