Notes
quarter circles in a rectangle in a circle solution

Quarter Circles in a Rectangle in a Circle

Solution by Similar Triangles, Angle in a Semi-Circle, and Angle Between a Radius and Tangent

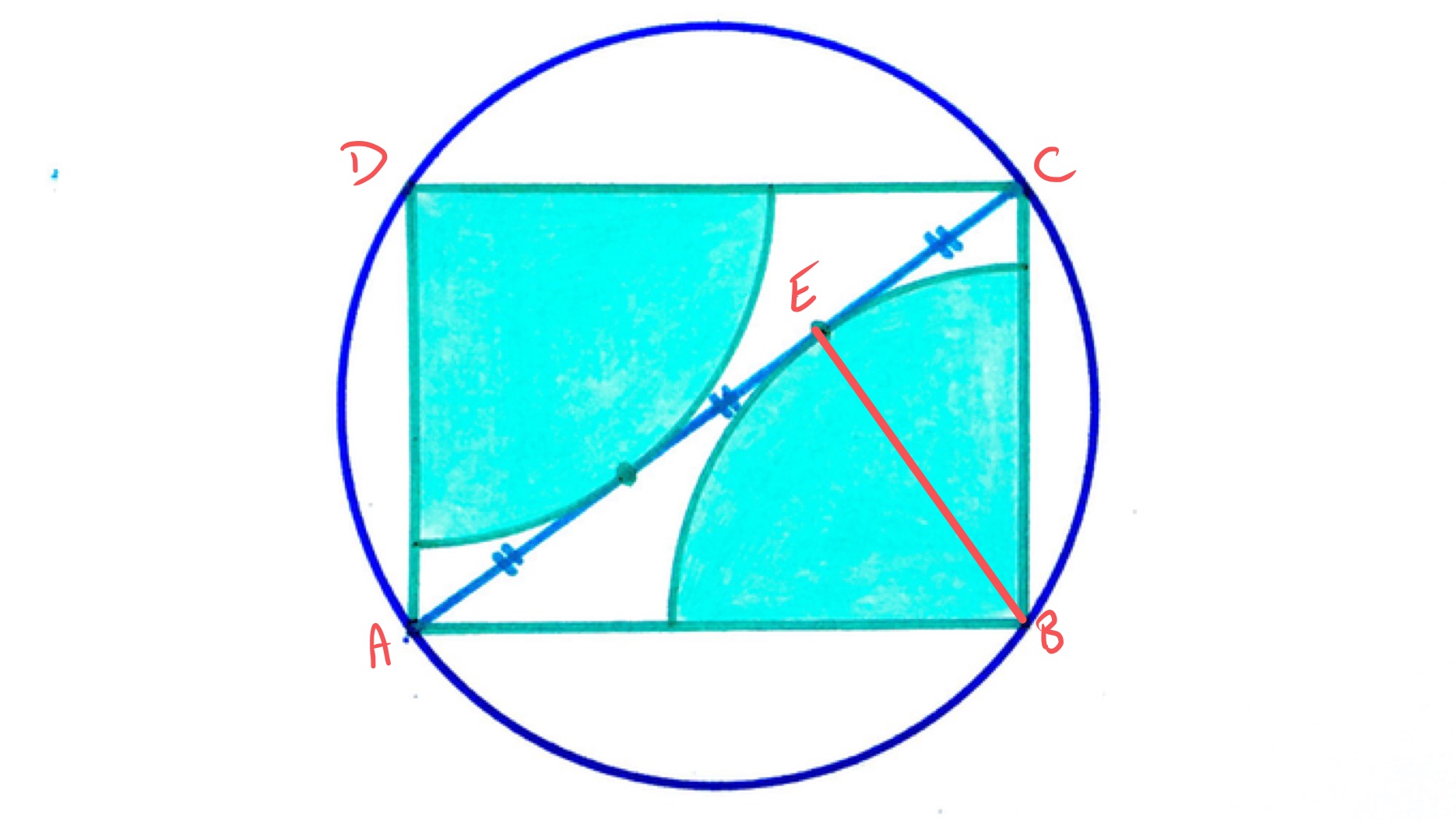
With the points labelled as above, $B E$ is a radius of the quarter circle and $A C$ a tangent where the radius meets the circle, so angle $B \hat{E} C$ is the angle between a radius and tangent so is a right-angle. This means that triangles $B E C$ and $A E B$ are similar, with $A E$ corresponding to $B E$.

Let $r$ be the length of $B E$, so that $r$ is the radius of the quarter circle, and let $x$ be the length of $E C$, so that $A E$ has length $2 x$. Then as $B E C$ and $A E B$ are similar, the ratio $x : r$ is equal to $r : 2 x$ which means that $r^2 = 2 x^2$.

As angle $C \hat{B} A$ is a right-angle, and the angle in a semi-circle is a right-angle, $C A$ is a diameter of the larger circle. Its radius is therefore $\frac{3}{2} x$.

The area of the larger circle is then $\frac{9}{4} \pi x^2$ and of the two quarter circles is $2 \times \frac{1}{4} \pi r^2 = \pi x^2$. Therefore the fraction that is shaded is $\frac{4}{9}$.