Notes
partitioned square solution
Solution to the Partitioned Square Puzzle
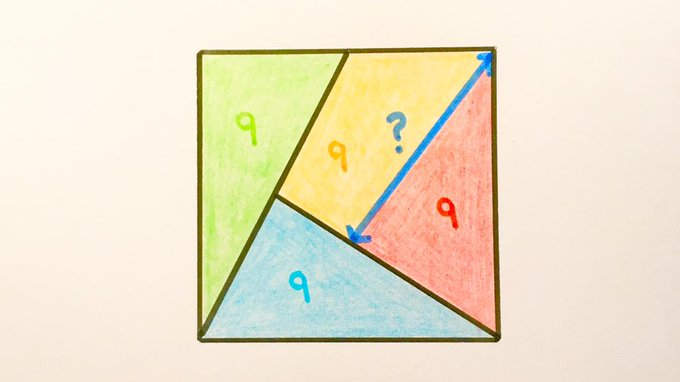
The four sections in this square have the same area. What’s the missing length?
Solution by Similar Triangles, Area of a Triangle and Square, and Pythagoras' Theorem
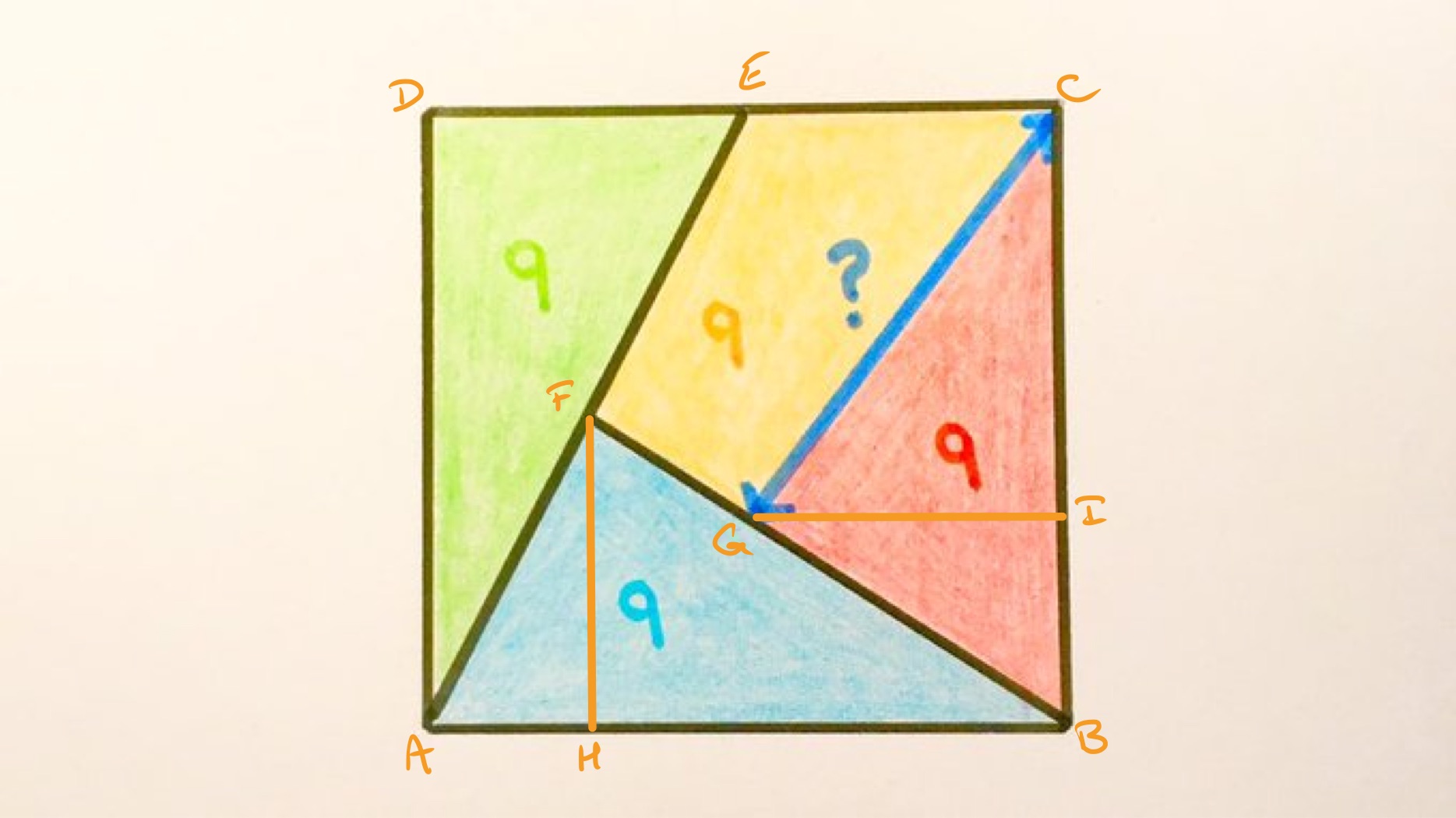
The area of the full square is , so its side length is . Considering triangle , it has area so applying the area of a triangle then the length of must be . Similarly, the lengths of and are also .
Triangle is similar to triangle , so as is half the length of , is half the length of , namely . Therefore has length . Similarly, triangle is similar to , and the ratio of the lengths of to is , so as corresponds to it has length .
Therefore has length , and so triangle is a right-angled triangle with shorter sides and . Its hypotenuse, which is , therefore has length by Pythagoras' theorem.