Notes
multiple semi-circles iv solution
Solution to the Multiple Semi-Circles IV Puzzle
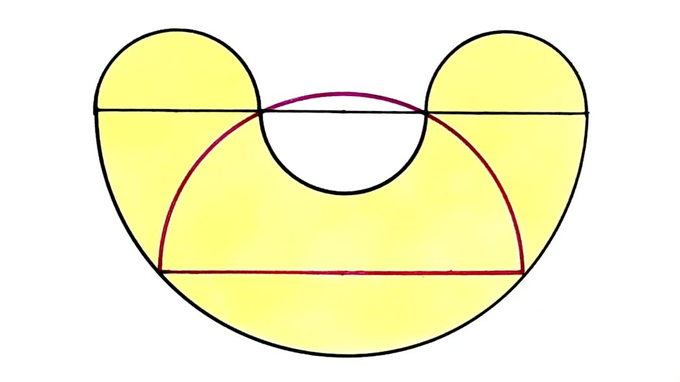
The three smallest semicircles are the same size. The area of the red semicircle is . What’s the total yellow area?
Solution by Pythagoras' Theorem and the Area of a Circle
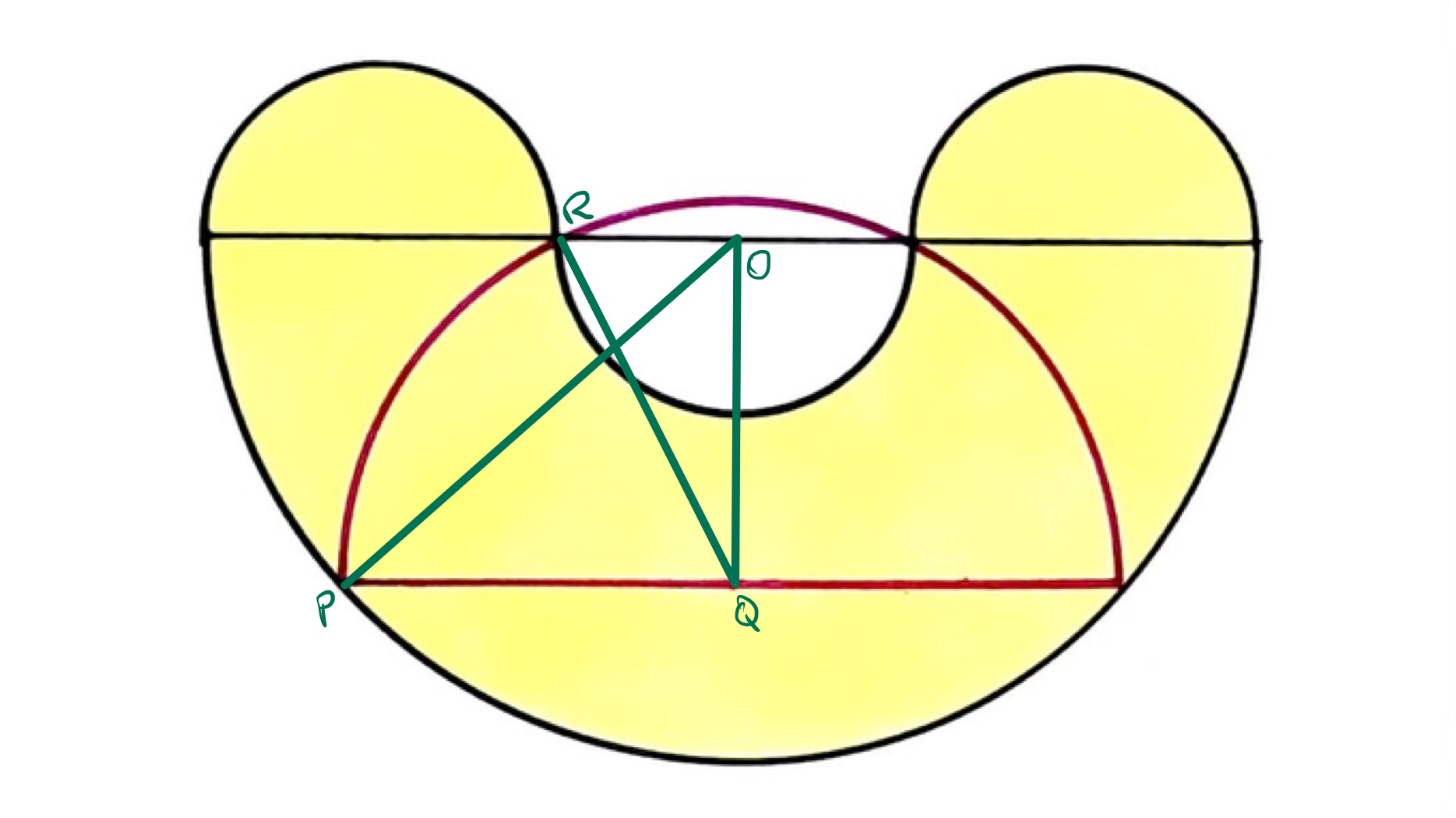
Let , , and be the radii of the circles, in ascending order. So with the points labelled as above then has length , and both have length , and has length . As the three smallest semi-circles are the same size, and span across the largest semi-circle, then . Let be the length of .
Applying Pythagoras' theorem to triangle shows that
Applying it to triangle shows that
Eliminating from these gives , so . The yellow region has area:
The information given in the puzzle shows that , so the yellow region has area .