Notes
four squares viii solution

Solution to the Four Squares VIII Puzzle

Solution by Pythagoras' Theorem

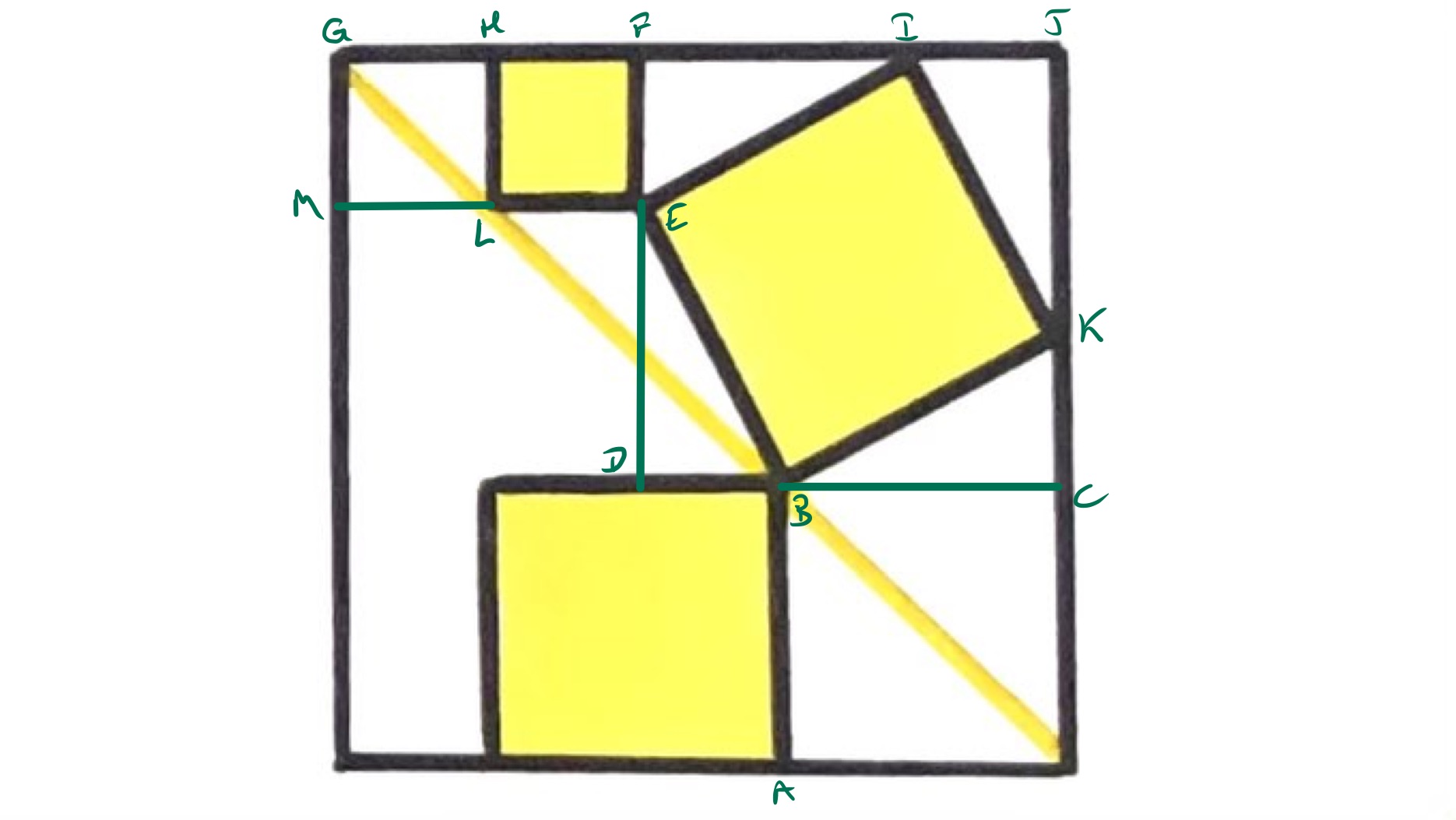
With the points labelled as in the above diagram, as the yellow line is a diagonal of the square, the lengths of $A B$ and $B C$ are the same. Similarly, $M L$ and $H L$ have the same length. The symmetry of the square $D C J F$, and the tilted square $B K I E$, shows that the line segments $B C$, $E D$, and $F I$ all have the same length.

Now let $a$ be the length of $A B$ and $b$ of $H F$. Reading across the top of the outer square, its width is $b + b + a + b = 3 b + a$. Reading up the middle, the height is $a + a + b = 2 a + b$. As it is a square, these are equal showing that $2 b = a$. The width of the outer square is then $5 b$ so its area is $25 b^2$.

Let $c$ be the length of $E B$. This is the hypotenuse of the right-angled triangle $E B D$ so applying Pythagoras' theorem shows that $c^2 = a^2 + b^2 = (2 b)^2 + b^2 = 5 b^2$. The area of shaded region is therefore $a^2 + b^2 + c^2 = (2 b)^2 + b^2 + 5 b^2 = 10 b^2$.

Therefore the fraction that is shaded is $\frac{10 b^2}{25 b^2} = \frac{2}{5}$.