Notes
four squares v solution
Solution to the Four Squares V Puzzle
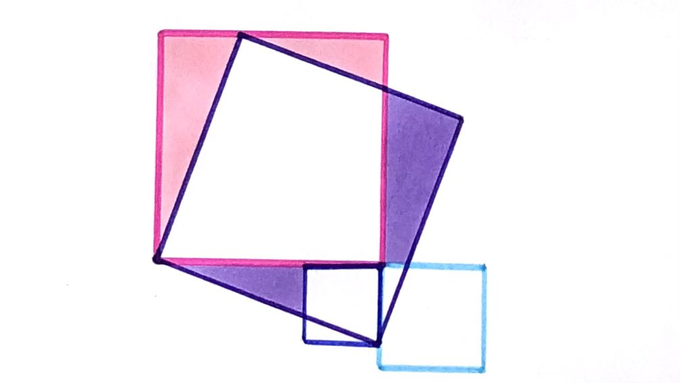
A design made from four squares. Is more of it shaded pink or purple?
Solution by Symmetry and Dissection
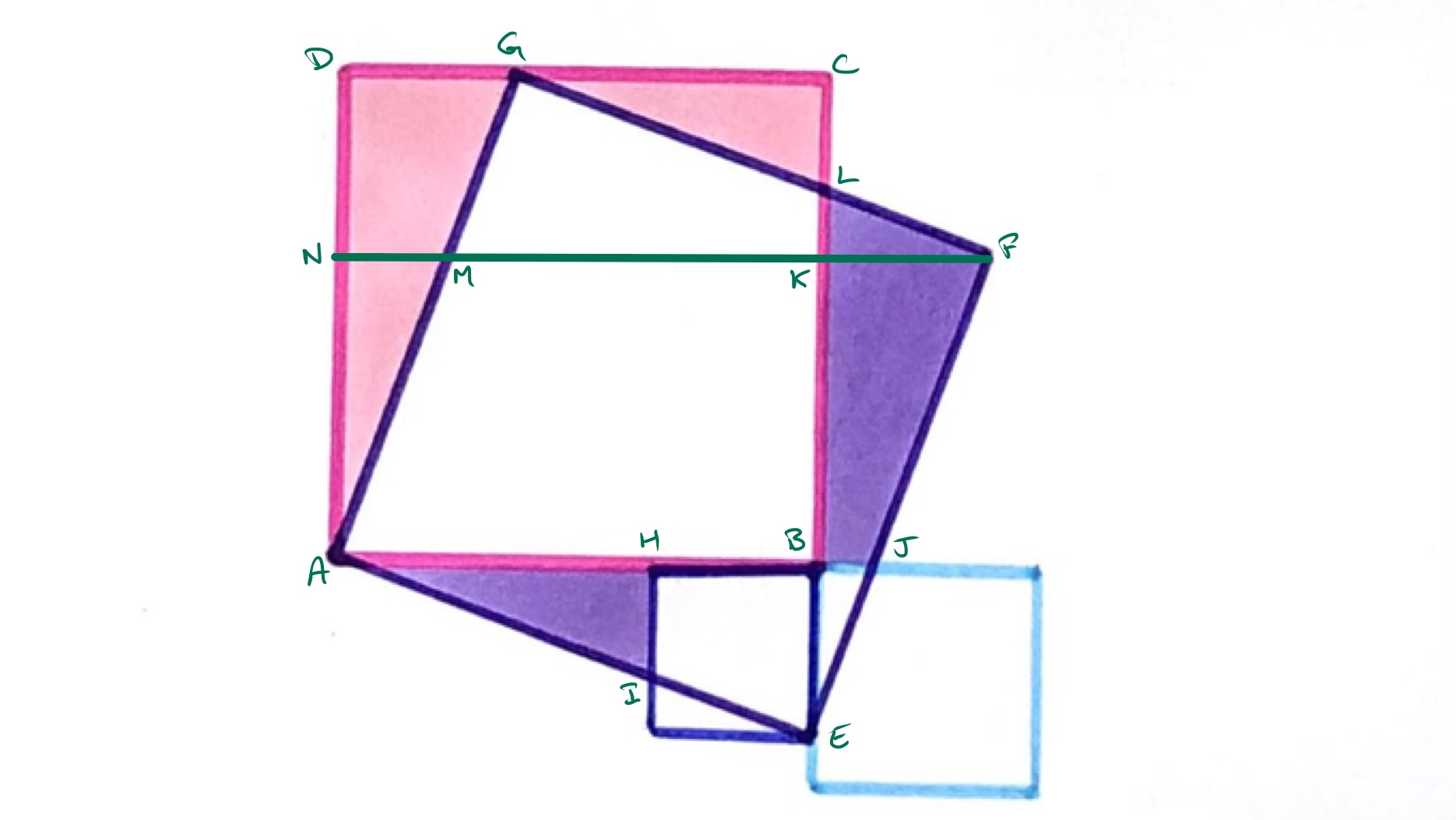
With the points labelled as above, consider rotating the diagram about the centre of the purple square by clockwise. This takes the line segment to , and the side rotates so that it overlaps with side , with rotating to . The point , as the intersection of with the line through and , therefore rotates to . Comparing lengths, and are the same length, and are the same length, and therefore and are the same length.
Since angles and are the same, this establishes triangles and as congruent and therefore the area of quadrilateral is the same as that of triangle . This triangle is congruent to , so the purple region and has the same area as the pink region .
This also establishes as having the same length as . Since and also have the same length, this means that and have the same length. Therefore, triangles and are congruent and so have the same area.
Therefore the pink and purple regions have the same area.
Solution by Invariance Principle
Given that the solution doesn’t depend on the angle of the purple square, there is a configuration of the squares that makes it clear, as below.