Notes
four squares iv solution
Solution to the Four Squares IV Puzzle
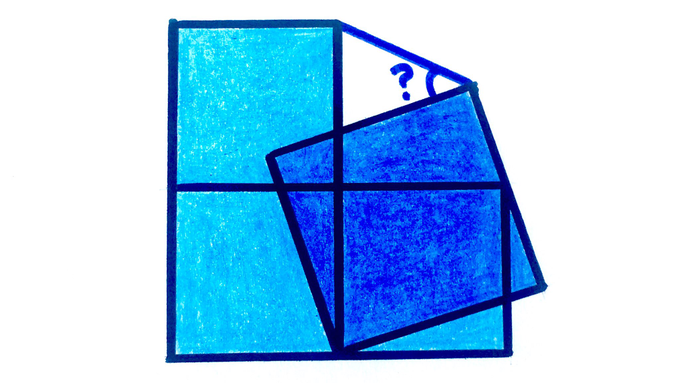
Four squares. What’s the angle?
Solution by Angle at the Centre is Twice the Angle at the Circumference and Angle in a Semi-Circle
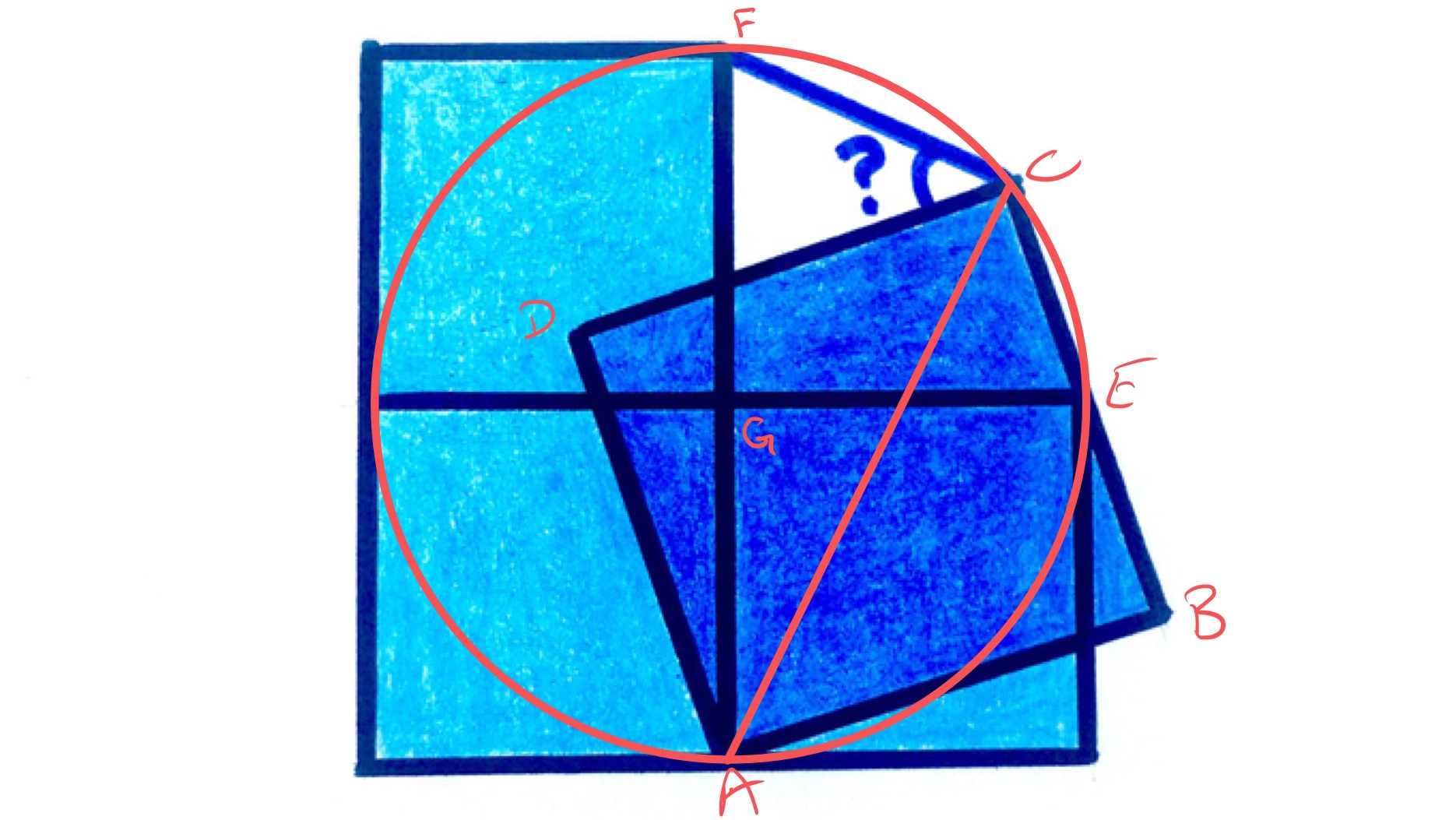
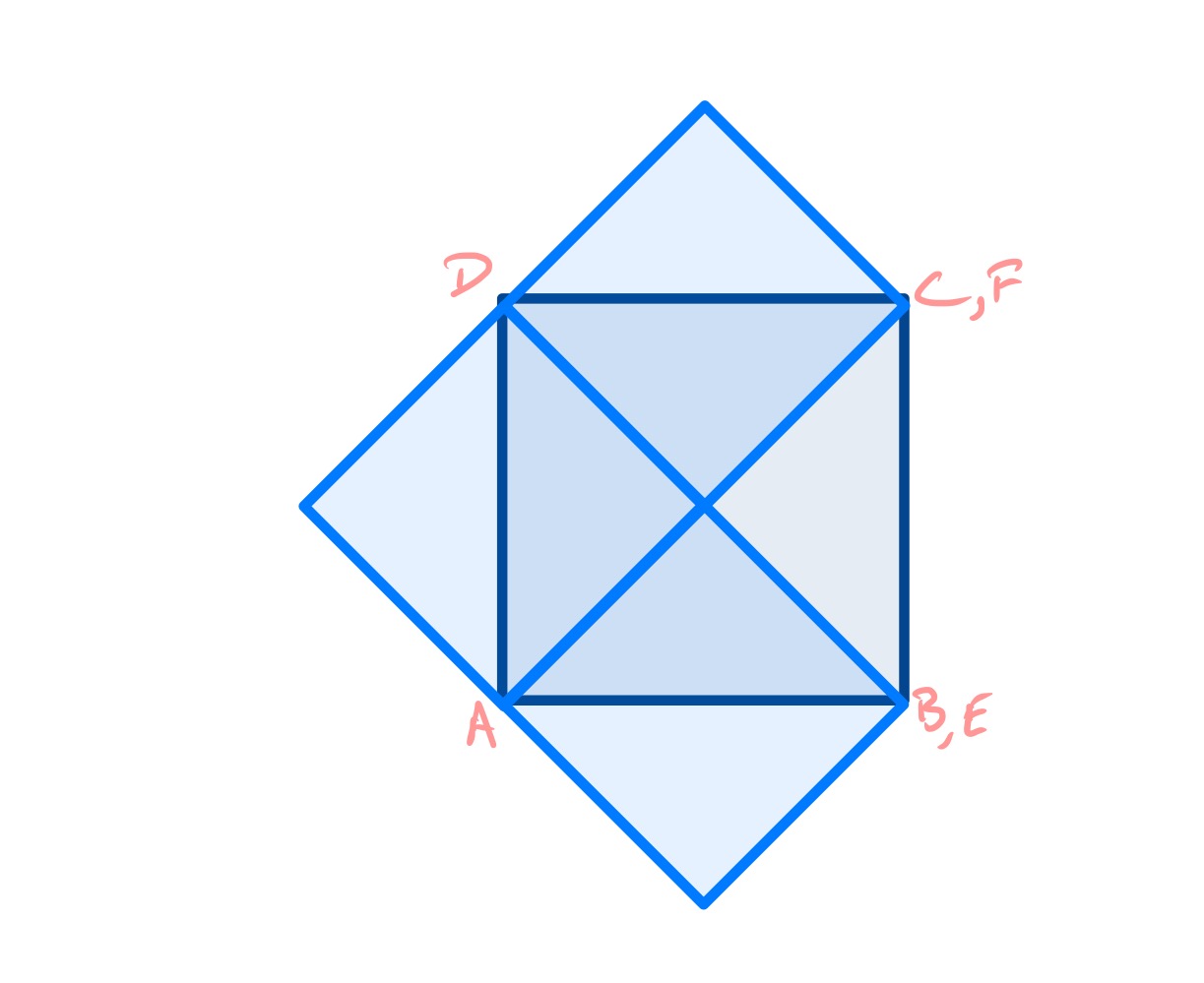
With the points labelled as in the above diagram, the circle is centred at and passes through the vertices , , and of the light blue squares.
As point lies on the line segment , angle is , while angle is . By the converse to the Angle at the Centre is Twice the Angle at the Circumference, this means that lies on the circle. Since is a diameter of the circle, angle is the angle in a semi-circle and so is . Then as angle is , angle is .
Solution by the Invariance Principle
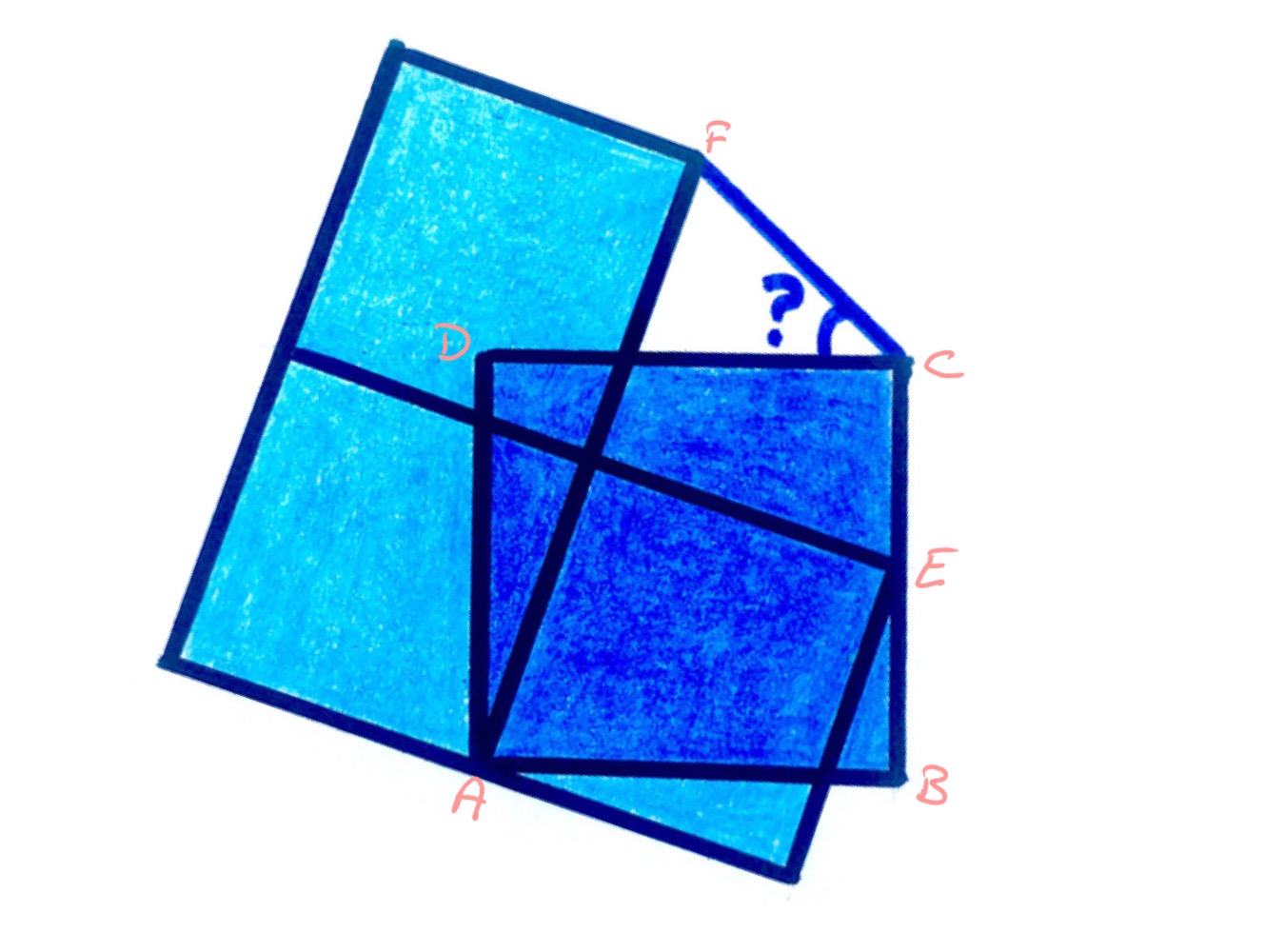
Consider the rotated diagram above and view the dark blue square, , as fixed. In this scenario, the point labelled is free to move on the line segment . As it does so, the point labelled also moves on a straight line (relative to the dark square). The extremes are when is at and at and are shown below. From this, moves on a line from at an angle of to the line segment .