Notes
four squares and a semi-circle solution

Four Squares and a Semi-Circle

Solution by Intersecting Chords

Let $a$, $b$, $c$, and $d$ be the side lengths of the four squares in ascending order. Then $d = a + c$.

Reflecting the diagram in the diameter produces the following diagram, in which $A P$ is the diagonal of the smallest square, $P B$ is the reflection of the diagonal of the second largest, and $P D$ is the reflection of the side of the second smallest.

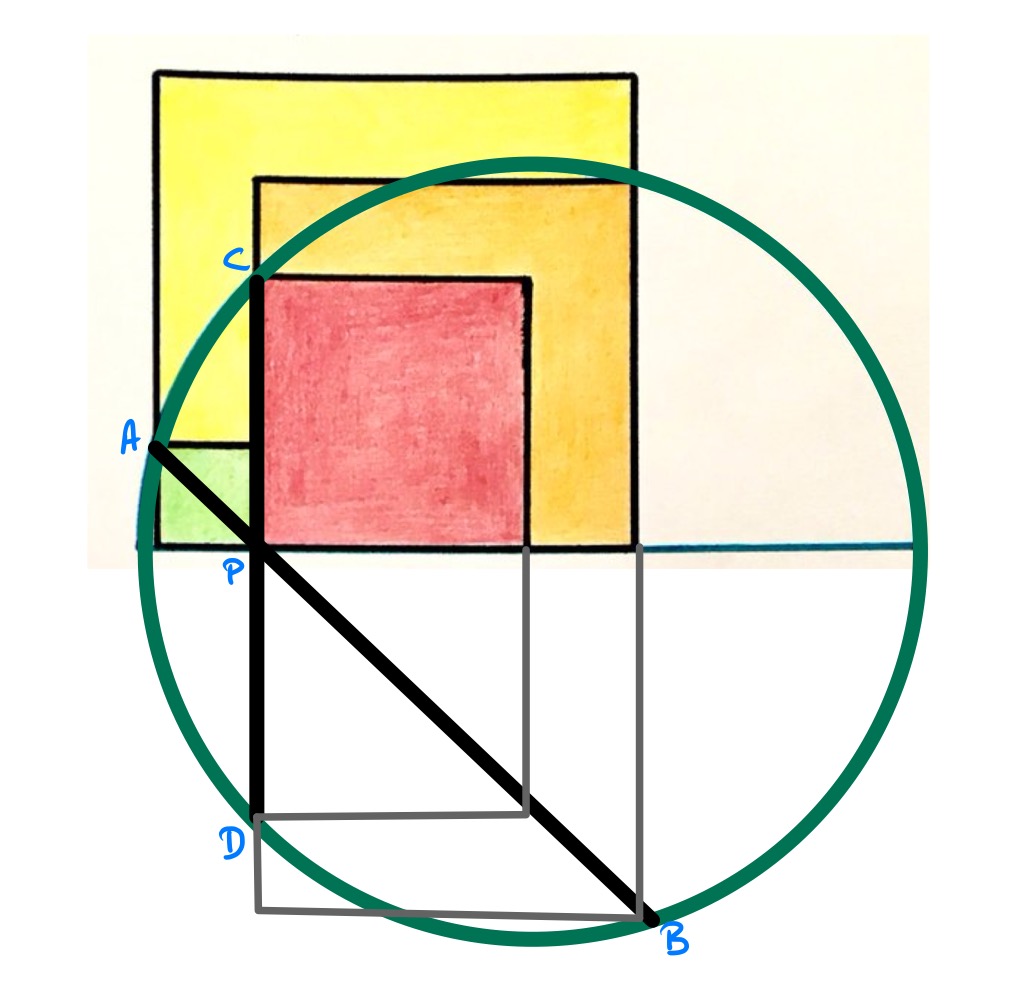
In this diagram, $A P$ has length $\sqrt{2} a$, $P C$ and $P D$ have length $b$, and $P B$ has length $\sqrt{2} c$. Using the intersecting chords theorem, then, these lengths fit into the equation

Now, since $d = a + c$, the area of the largest square is equal to:

Therefore, the three smaller squares have the same combined area as the largest. The total area is thus $36$.