Notes
five triangles in a rectangle solution
Five Triangles in a Rectangle
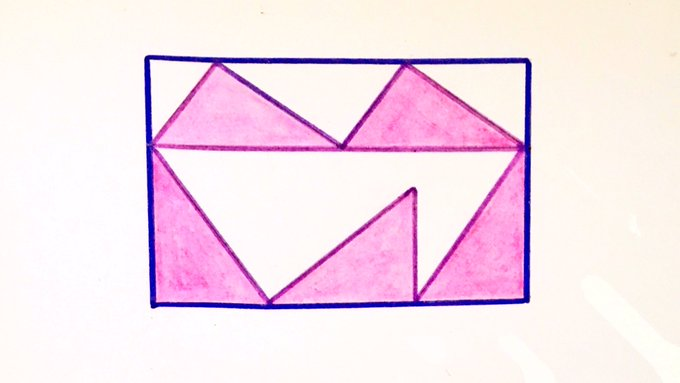
Five copies of the same triangle have been fitted into a rectangle. What fraction of it do they cover?
Solution by Pythagoras' Theorem
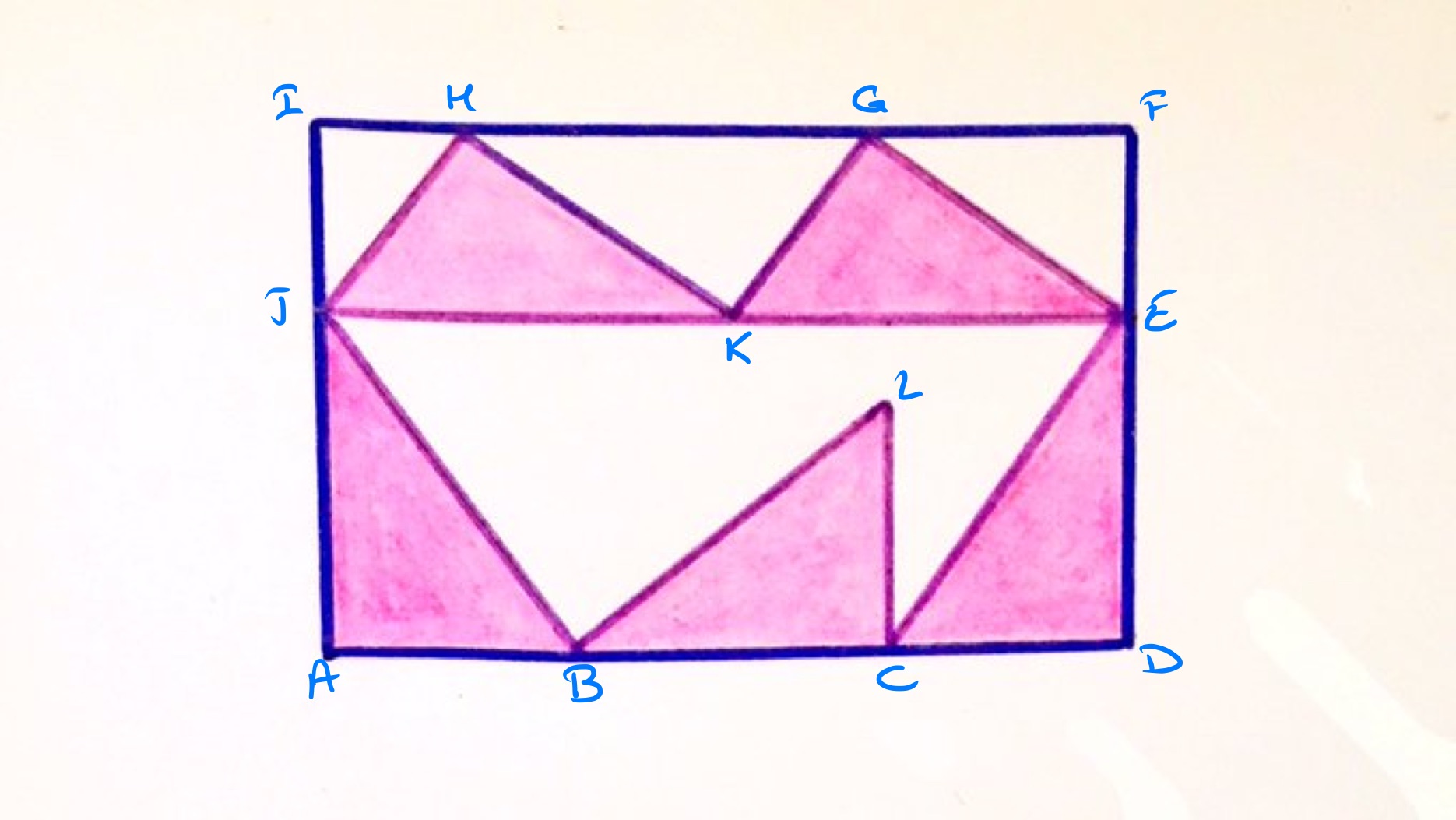
With the points labelled as above, let , , and be the lengths of the sides of the triangle in increasing order, so that is the length of , of , and of . Comparing the lengths of with gives the identity . Since triangle fits in the corner of the rectangle, it is a right-angled triangle and so Pythagoras' theorem applies to give that .
Squaring the first identity shows that which shows that and so . Let , then , , and .
The area of one triangle is then , so the area of all the triangles is . To calculate the area of the rectangle, split it along . The area of is the area of four triangles, so is . The area of is . The total area is then . The fraction of the rectangle covered is therefore .