Notes
five squares across a square solution

Solution to the Five Squares Across a Square Puzzle

Solution by Similar Triangles

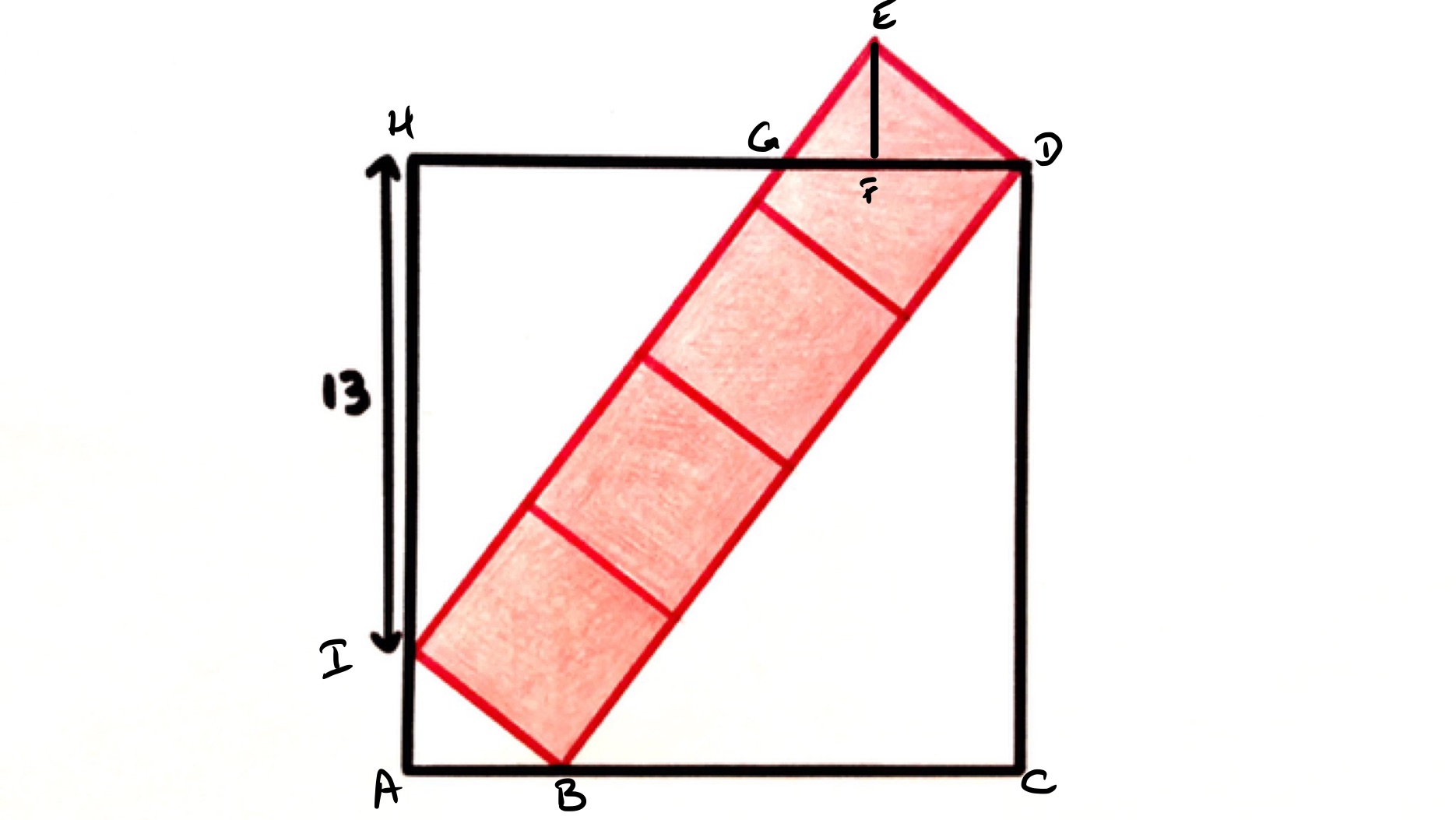
There are many similar triangles in this diagram (using angles on a straight line and angles in a triangle to demonstrate their similarity). Triangles $A B I$, $C D B$, and $H I G$ are all similar (with vertices corresponding in the given orders). The length of $B D$ is four times that of $B I$, so the lengths of $C D$ and $B C$ are four times those of $A B$ and $I A$ respectively. Since $A C$ and $C D$ have the same length, $B C$ must therefore be $\frac{3}{4}$ of the length of $C D$. Then the length of $I A$ is $\frac{3}{4}$ of that of $A B$, which is a quarter of the length of $C D$, so the length of $I A$ is $\frac{3}{4} \times \frac{1}{4} = \frac{3}{16}$ of the length of $C D$. The remainder of the side $A H$, namely $I H$, is therefore $\frac{13}{16}$ of the full length. As this side has length $13$, the side length of the square is therefore $16$.

Many of the lengths can now be filled in:

• $I A$ has length $3$
• $A B$ has length $4$
• $B C$ has length $12$
• $H G$ has length $\frac{3}{4} \times 13 = \frac{39}{4}$
• $G D$ has length $16 - \frac{39}{4} = \frac{25}{4}$
• $E F$ has the same length as $I A$, namely $3$ ($F$ is the point directly below $E$ on $G D$)

The side length of the shaded squares can be calculated as $5$ using Pythagoras' Theorem giving a total shaded area as $4 \times 25 = 100$, but that is not necessary to solve the stated problem as the shaded area can be calculated by finding the areas of the white triangles using the lengths above, subtracting that from the area of the square, and adding the area of triangle $G D E$. These areas are:

• $A C D H$ has area $16^2 = 256$
• $B C D$ has area $\frac{1}{2} \times 12 \times 16 = 96$
• $A B I$ has area $\frac{1}{2} \times 3 \times 4 = 6$
• $H I G$ has area $\frac{1}{2} \times \frac{39}{4} \times 13 = \frac{507}{8}$
• $E G D$ has area $\frac{1}{2} \times \frac{25}{4} \times 3 = \frac{75}{8}$

So the shaded region has area: