Notes
five overlapping circles solution
Five Overlapping Circles
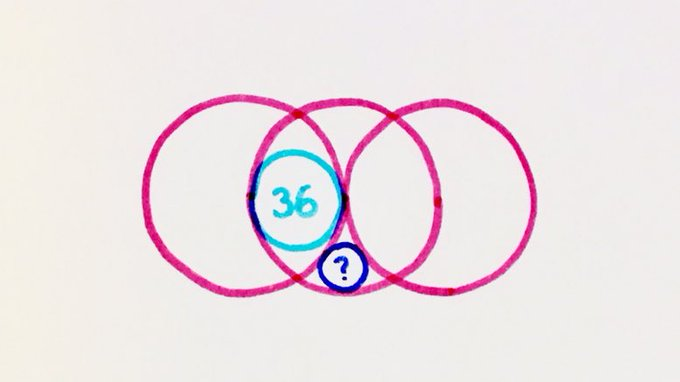
What’s the area of the smallest circle?
Solution by Pythagoras' Theorem
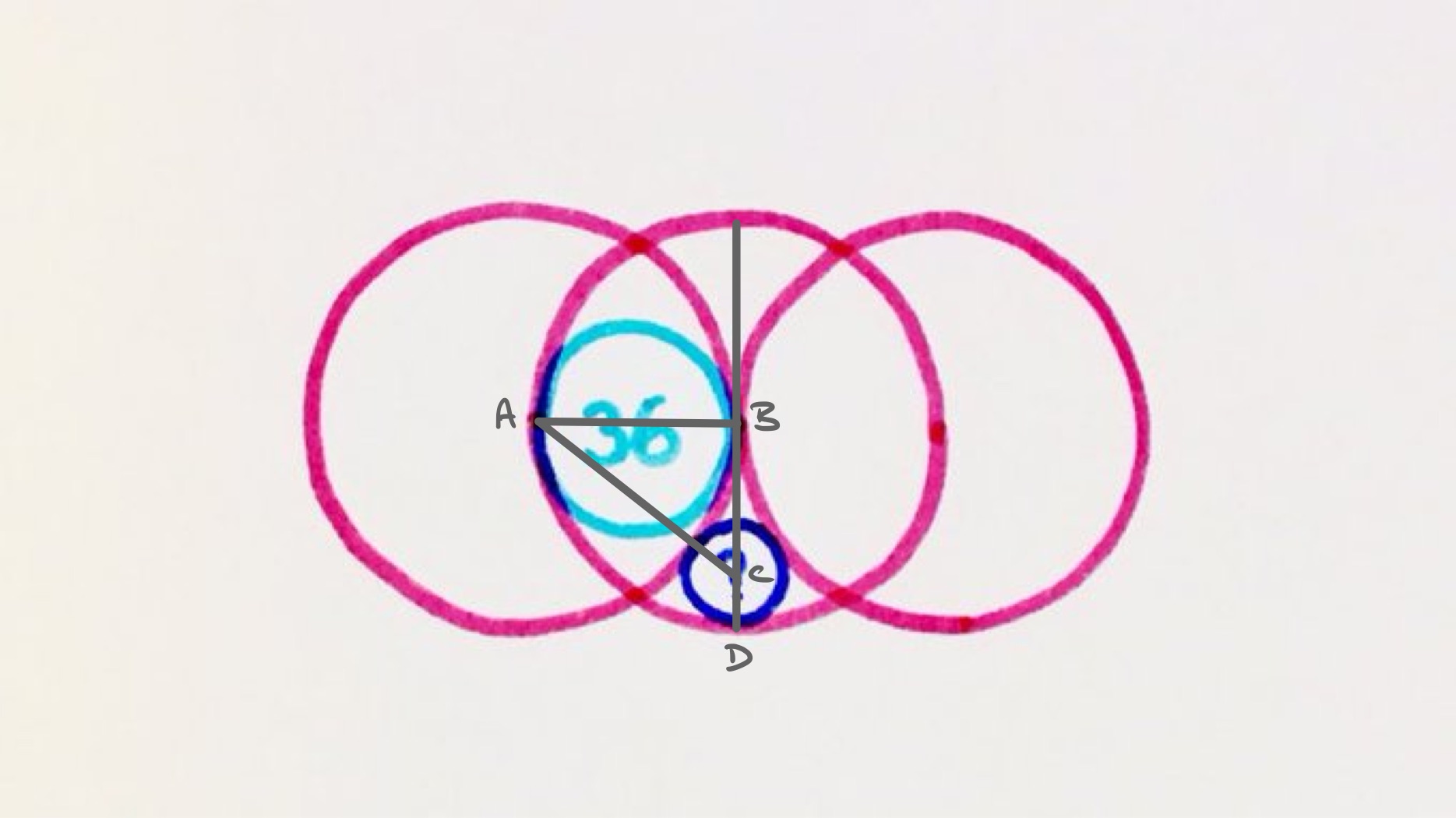
Let be the radius of the cyan circle and of the dark blue. Then the length of is , of is , and of is . Applying Pythagoras' theorem to triangle shows that:
This simplifies to , so . This means that the area of the small circle is a quarter of the area of the cyan circle, so has area .