Notes
five congruent rectangles solution
Solution to the Five Congruent Rectangles Puzzle
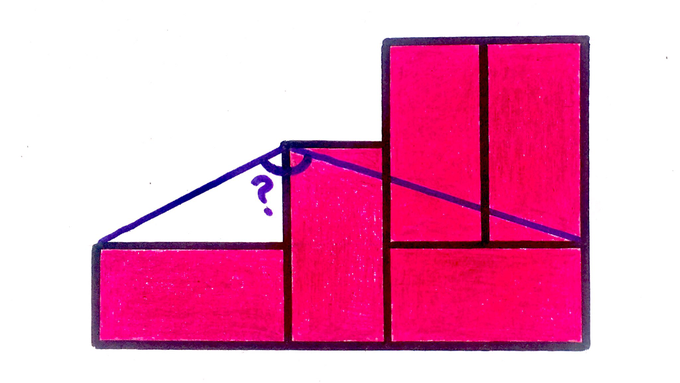
Five congruent rectangles. What’s the angle?
Solution by Congruent Shapes
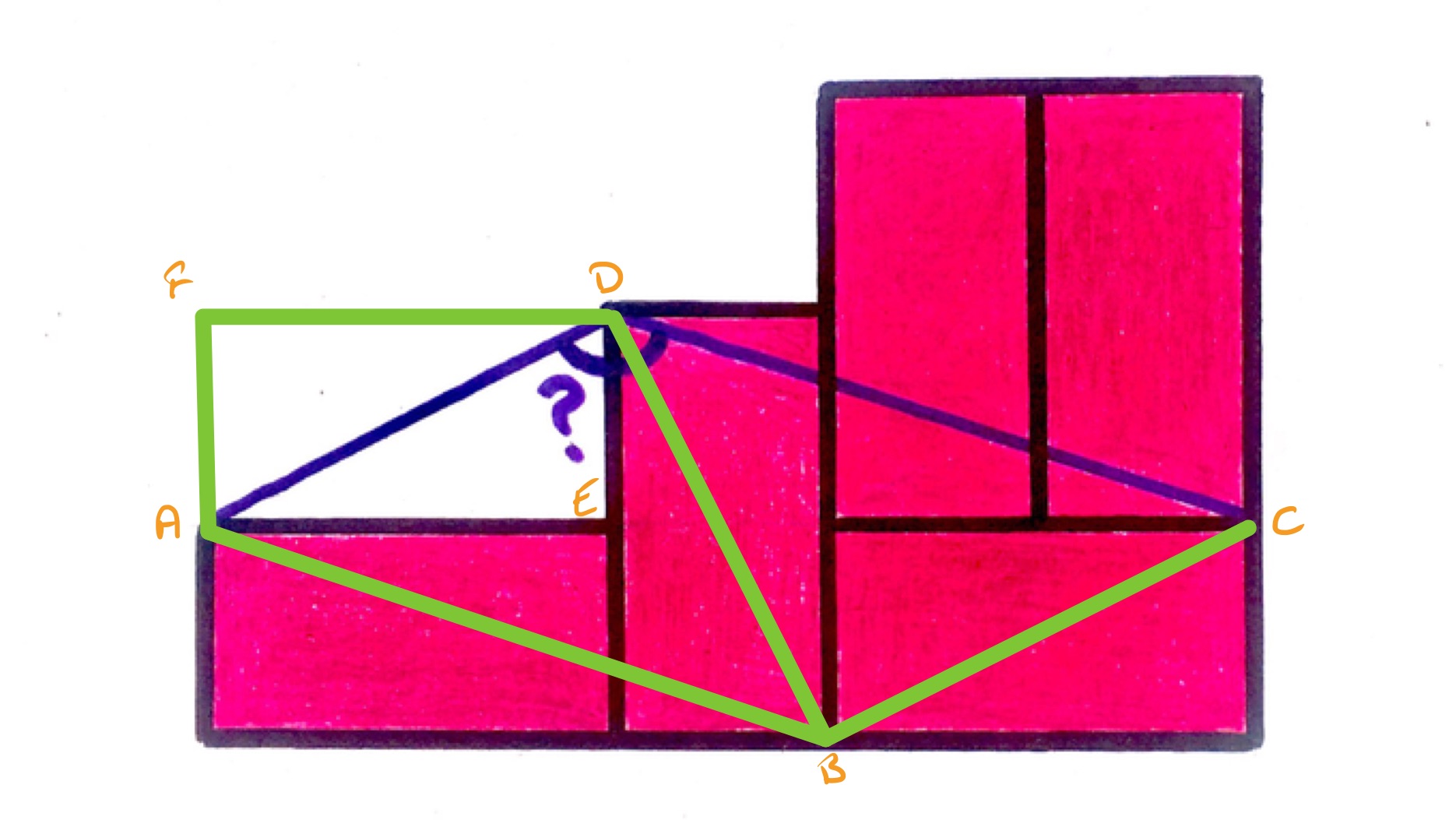
By considering the three rectangles on the right-hand side, the basic rectangle in this diagram has sides with lengths in the ratio . The point is therefore the midpoint of the side of the vertical rectangle and so the rectangle is therefore also congruent to the basic rectangle.
The line segments , , and are then all diagonals of their respective rectangles and since all these rectangles are congruent, they have the same length. The middle of these three rectangles is oriented vertically while the other two are horizontal, so the line segment is perpendicular to the other two. So angle is a right-angle. Triangle is then an isosceles right-angled triangle so angle is . Putting these together, angle is .