Notes
five circles in a square solution
Solution to the Five Circles in a Square Puzzle
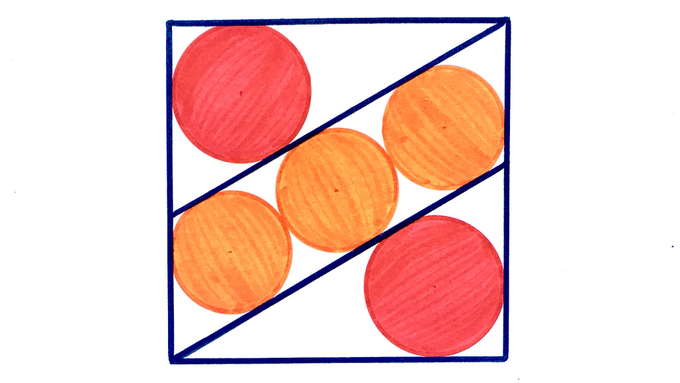
Five circles in a square. The total red area is . What’s the total orange area?
Solution by Trigonometry and Lengths in an Equilateral Triangle
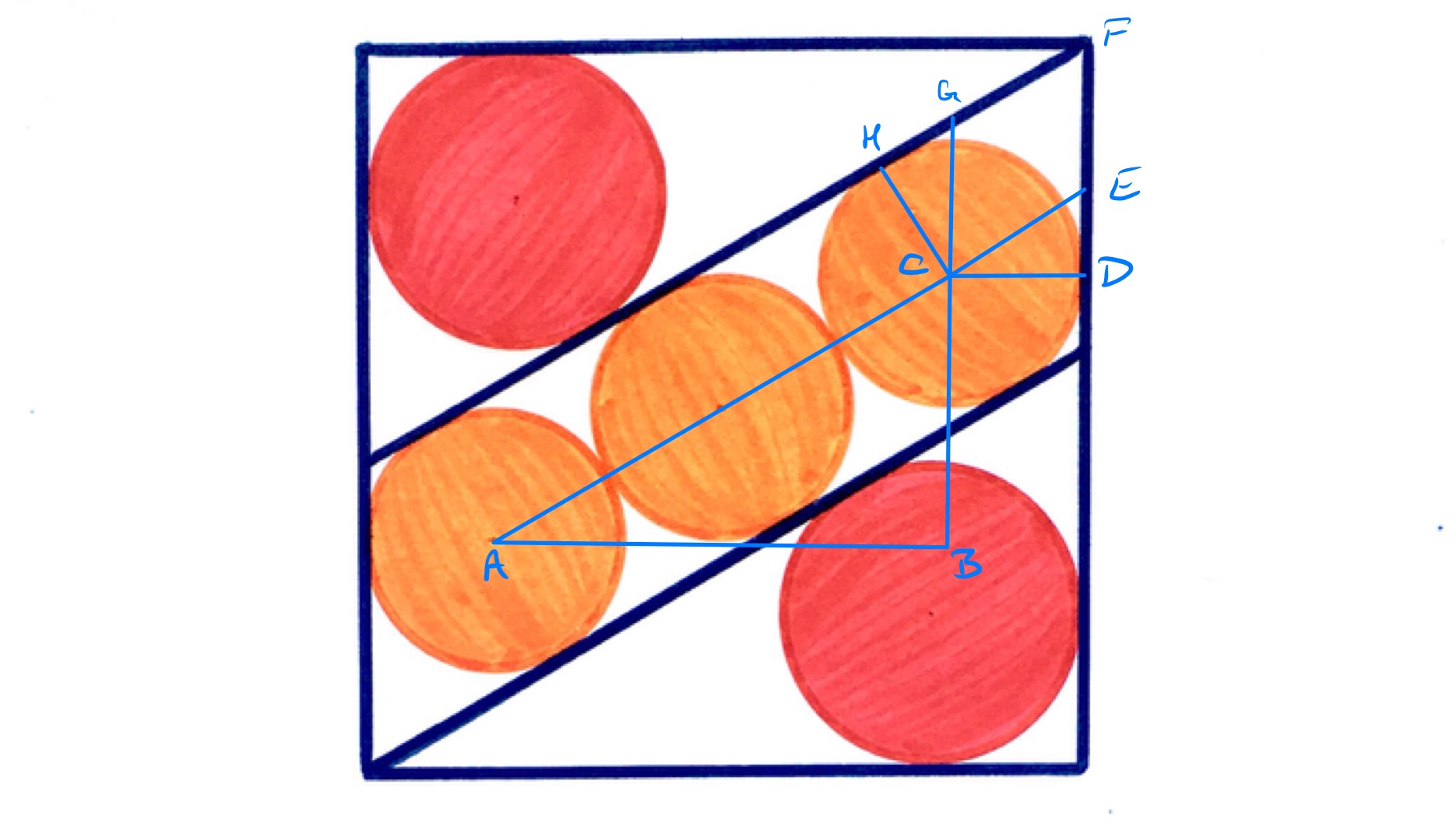
In the above picture, let be the radius of the orange circles. The points and are centres of their circles and is so that is horizontal and is vertical. Let be angle .
The length of the line segment is , so has length and has length . Since has length , the horizontal length of the square is .
As has length , has length and has length . So the total height of the square is .
Since it is a square, these two expressions must be equal. Setting them equal and working through goes as follows:
The last step uses the compound angle formula with one of the angles set to together with the fact that .
This has solutions and , where is some integer. The first rearranges to , so , and the second to . Since is acute, the only solution is .
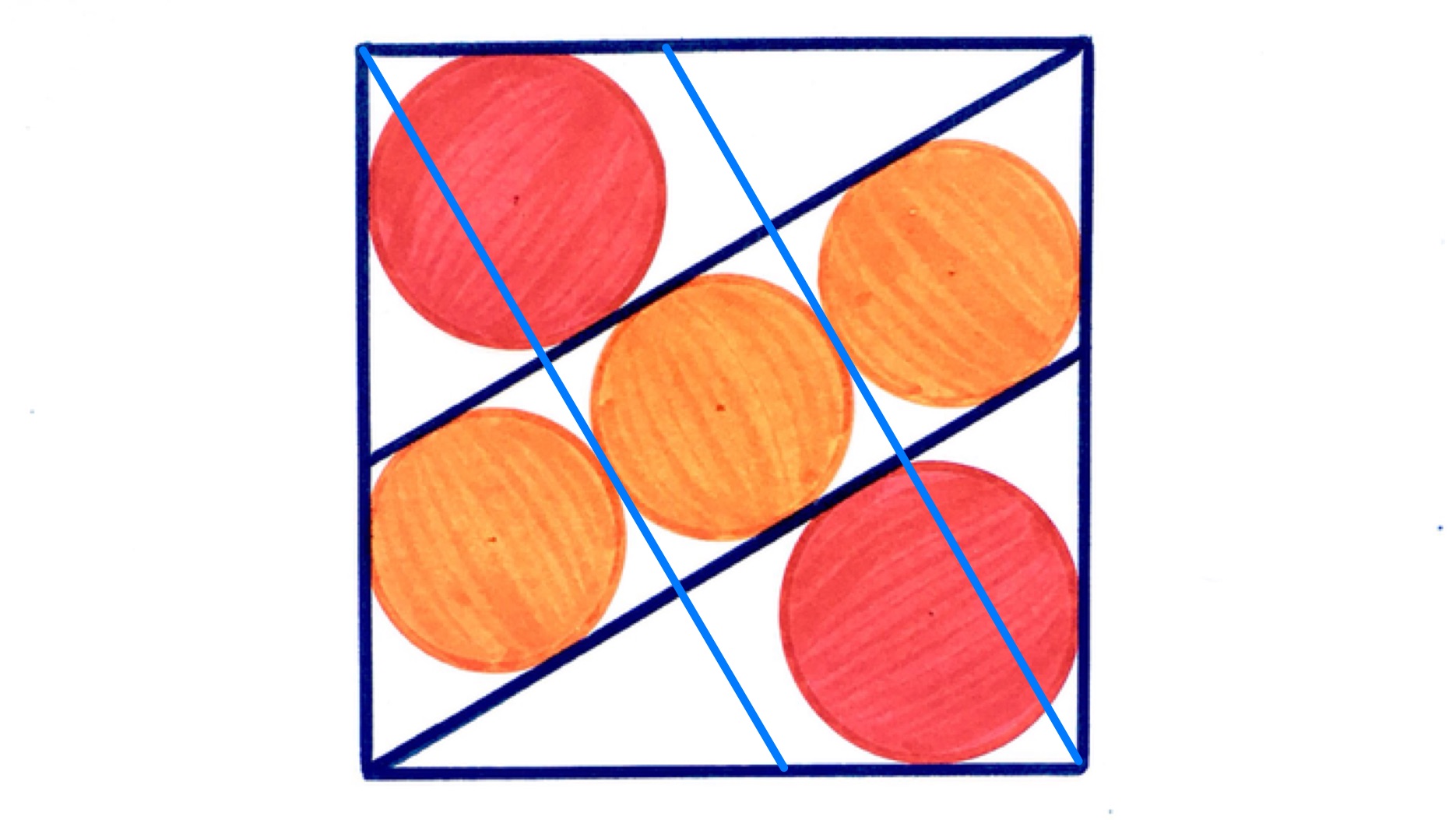
With established as , the above diagram shows the relationship between the orange and red circles. Each is the incircle of a -- triangle, with the side adjacent to on the larger circle being the hypotenuse of the smaller. The length scale factor is therefore the ratio of the length of the hypotenuse to the length of that side, which from lengths in an equilateral triangle is . So the area scale factor is . That is, the area of each orange circle is that of the red. Since the total red area is , each red circle is and thus each orange circle is meaning that the total orange area is .